3. THE CMBR AND INFLATION

The measurement of the characteristics of the anisotropies in the temperature of the CMBR can teach us a lot not only about the processes that gave rise to the density perturbations responsible for the appearance of the large-scale structures we observe today, but also about cosmological parameters, like the Hubble constant and the matter and energy content in the Universe, and physical events that happened between decoupling and the present, like re-ionization and the formation of structure (e.g. cluster formation through the Sunyaev-Zel'dovich effect). However, there are so many possible ways in which the properties of the CMBR anisotropies can be altered, the most troublesome being by changing the nature of the initial perturbations and/or of the dark matter (energy), that in order to extract a tight interval of confidence for some parameter, e.g. the value of the Hubble constant, it is necessary to make a priori some restrictions to the nature of the phenomena that might affect the characteristics of the CMBR temperature anisotropies.

Therefore, once again the strategy has to be to initialy work within a simplified framework, and only if the observational data demands it, expand the initial assumptions so as to widen the allowed parameter space. At present, the simplified framework within which most analysis of the present CMBR anisotropy data are made has 10 associated parameters, which may or may not be allowed to vary freely, and assumes primordial, adiabatic perturbations with a Gaussian distribution. The dark matter is taken to be of only two possible types, cold or massive standard neutrinos, and any dark energy is assumed to behave dynamically as a classical cosmological constant. We will follow the choice of parameters of (73), as slightly different, in practice equivalent, sets of parameters can be worked with. We then have 5 cosmological parameters: _k, the spatial curvature, equal to - k/a²H²; , the energy density associated with a classical cosmological constant, , which is equal to / 3H²; w_{cdm cdm}h², the matter density in the form of cold dark matter; w h², the matter density in the form of standard neutrinos; w_{b b}h², the matter density in the form of baryons. There are also 2 parameters that characterise the primordial power spectrum of the scalar adiabatic perturbations, which is assumed to have a power-law dependence with scale, _H²(k) k^{n_s - 1}: n_s, the spectral index of the scalar perturbations; A_s, the contribution to the CMBR quadrupole anisotropy by scalar perturbations, which is in practice equivalent to using _H, the amplitude of the scalar perturbation power spectrum. And 2 parameters that characterise the primordial power spectrum of tensor perturbations, i.e. gravity waves, which is also assumed to have a power-law dependence with scale, P_t(k) k^n_t: n_t, the spectral index of the tensor perturbations; A_t, the contribution to the CMBR quadrupole anisotropy by tensor perturbations. Finally, there is 1 physical parameter: , the optical depth to the surface of last scattering (equal to zero if there is no re-ionization after recombination occurred). From these quantities other well known parameters can be obtained, like the total matter density in the Universe, _m = 1 - _k - , and the value of the Hubble constant.

Again, it should be emphasised that any conclusions on the value of these parameters, obtained by comparing the theoretical expectation with the CMBR anisotropy data, is restricted to the tight framework imposed. The conclusions could be relaxed substantially if one was to consider introducing the added possibilities of part of the perturbations responsible for the CMBR anisotropies being active, incoherent, isocurvature, or with a non-Gaussian distribution, or the existence of more exotic types of dark matter (warm, or decaying, or self-interacting) or dark energy which does not behave dynamically as a cosmological constant (e.g. an evolving scalar field).

Through the study of the distribution of CMBR temperature anisotropies in the sky at different angular scales, it is possible to confirm or disprove the assumption of a Gaussian distribution for the perturbations. If these have a Gaussian distribution, them the anisotropies should also have one. At present only the COBE data has been searched for evidence of deviations from Gaussianity. Early results [see e.g. (47, 30, 34)] were negative. However, more recently, there have been reports of strong evidence for deviations from a Gaussian distribution in the COBE data (29, 63, 65, 28, 60). However, some analysis continue to dispute the presence of a clear non-Gaussian signal (10, 4, 8).

Among the contradicting reports, it has become clear that the one-point distribution of the COBE anisotropies is indeed Gaussian, but the anisotropies seem not to be as randomly distributed across the sky as they should be under the Gaussian (random-phase) hypothesis, (10). The later is supported by the fact that the statistical techniques that find evidence for non-Gaussianity seem to be those which are most sensitive to phase-correlations. The source for these could be instrument noise, the method of extraction of the monopole, dipole or the Galactic contribution, unaccounted for systematic effects associated with the way COBE collected data (3), or of course real features in the microwave sky, which could be at the surface of last scattering or due to some unknown source of foreground contamination.

Observational evidence for a primordial and adiabatic origin for the perturbations that produced the CMBR temperature anisotropies is scarce at present, even after the release of the Antarctica long duration flight data from the BOOMERanG experiment (20, 51) and data gathered by the first flight of the MAXIMA balloon (32).

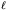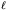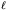The evidence for the primordial nature of the perturbations will come from the signature that the acoustic oscillations induced in the photon-baryon fluid leave in the angular power spectrum of the CMBR anisotropies: a sequence of peaks in space. Their adiabatic nature is revealed by the spacing between the peaks: ₁ : ₂ : 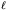₃ ... corresponds to ratios of 1 : 2 : 3 : .... Isocurvature primordial perturbations also generate a sequence of peaks in the CMBR temperature anisotropy angular power spectrum, but at different locations. The first peak due to the acoustic oscillations, generated by the potential wells that result from the isocurvature primordial perturbations after they enter the horizon, is moved to an higher 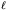value, and the ratios between the first three (real) peak locations are now 3 : 5 : 7 : .... The ratios between the peaks in both cases only depend weakly on the assumed cosmological parameters, and therefore provide an excellent test of the adiabatic or isocurvature nature of the fluctuations (37, 38). Unfortunately, at present, though the BOOMERanG LDF and the MAXIMA-1 temperature anisotropy measurements go to sufficiently high 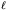to probe the expected location of a second peak in the adiabatic case, the evidence is inconclusive. Nevertheless, purely isocurvature perturbations seem to be highly disfavoured just by the shape of the first peak (49). So, presently one cannot use solely CMBR anisotropy data to distinguish between an adiabatic or an isocurvature origin for the density perturbations. In any case, the present known location of the first acoustic peak (~ 200) is sufficient to say that if the Universe is spatially flat then most probably the initial perturbations were adiabatic, as one then expects 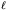₁ 220. Isocurvature perturbations necessarily need the Universe to be closed, which moves the horizon scale at decoupling to larger angular scales, given that if the Universe was flat then the first acoustic peak for primordial isocurvature perturbations would be expected at 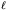₁ 340. If one admits the possibility that both adiabatic and isocurvature modes are present, then even with MAP it will be difficult to estimate the relative amplitude of both. One will have to wait for Planck to measure such a quantity with less than 10 per cent error (7).

As discussed before, the appearance of acoustic peaks in the angular power spectrum of the CMBR temperature anisotropies is not proof of an inflationary (or primordial) origin for the initial perturbations, though their absence could only be (realistically) explained by early and substantial (i.e. close to unity) re-ionization of the intergalactic medium. However, the fact that at least one acoustic peak has been detected argues against the later scenario, and its presence is evidence for some degree of coherence of the initial perturbations (which does not mean their are primordial, as previously mentioned).

In summary, the primordial, adiabatic and Gaussian nature of the initial perturbations seems to be still a viable first assumption, providing the simplest coherent framework within which it is possible to explain the present CMBR temperature anisotropy data. Unfortunately, even under this framework the CMBR data presently does not tell us much about the parameters n_s, A_s,n_t, and A_t, which characterise the power spectrum of the scalar and tensor perturbations in the simplest inflationary models.

Gravitational waves can only contribute significantly to the CMBR temperature anisotropies on scales above 1°, or 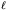< 100, given that at the surface of last scattering any gravitational waves inside the horizon had their amplitude severely diminished by redshifting. This means that only the COBE and Tenerife data (73) might in principle be sensitive to the tensor spectral index n_t. However, the effect of varying n_t can be easily masked by simultaneously varying the scalar spectral index n_s, or by reducing the tensor contribution to the large-angle CMBR anisotropy to levels small enough that (almost) any variation of n_t could be accommodated within the measured errors. Therefore, at present there are no useful observational limits on the value of n_t.

The ratio between A_t and A_s, also sometimes called T/S, should in principle be a more readily measurable quantity, as it affects the height of the first acoustic peak relatively to the large-angle Sachs-Wolfe plateau, and also the CMBR anisotropy normalisation of the density perturbation power spectrum, thus affecting the formation of large-scale structure. By analysing these two effects, (84) concluded that values of T/S as large 4 are still viable, as long as n_s is simultaneously allowed to go up to 1.2. Adding the initial data coming from the BOOMERanG and MAXIMA experiments seems to decrease the maximum possible value for T/S to 2 (46). Restricting n_s to be lower or equal to unity, given that this is what is predicted in the simplest inflationary models that produce a cosmologically significant background of gravitational waves, then the ratio T/S would need to be lower than about 1. In particular, in the case of power-law inflation models the observational data seems to require T/S < 0.5.

Finally, n_s is also not very well constrained at present by CMBR anisotropy data alone or in conjunction with structure formation data. The CMBR data by itself badly constrains n_s if all the 10 parameters associated with the simplest inflationary motivated structure formation models are allowed to vary (73). By fixing the matter density in the form of baryons to be _bh² = 0.02 (as implied by observations if homogeneous standard nucleosynthesis is assumed), and a reasonable range for the Hubble constant, 0.55 < h < 0.75, a interval for n_s can be obtained, going from 0.8 to about 1.5 (73). However, values for n_s higher than 1.3 can only be obtained at the cost of having a very large value for T/S (73, 52). And in any case the the initial data from the BOOMERanG and MAXIMA experiments seem to disfavour values for n_s above 1.2 (40, 43, 46). We have seen that structure formation observations do not constrain very well n_s either, with values between 0.7 and 1.4 being possible. Joining all the data together would thus indicate that a value for n_s close to 1 is preferable, with the possibility of going as low as 0.8 or as high as 1.3. The upper limit is also confirmed by bounds imposed on the production of primordial black holes (42).

Looking into the future, the prospects of measuring the inflationary parameters n_s, A_s, n_t, and A_t from CMBR anisotropies are mixed. Data from balloon experiments like BOOMERanG and MAXIMA will not help much in improving these constraints, except for a better handle on the value of n_s. As mentioned, initial data from the two experiments has already further restricted the value of n_s to being within about 10 per cent of unity (40, 43). The results from the MAP and Planck Surveyor satellites will however change the situation. All studies that have been done to date of the precision with which both MAP and Planck will be able to estimate different cosmological parameters use the so-called Fisher information matrix. This gives the manner in which experimental data depends upon a set of underlying theoretical parameters that one wishes to measure. Within this set of parameters, the Fisher matrix yields a lower limit to error bars and hence an upper limit on the information that can be extracted from such a data set, i.e. the Fisher matrix reveals the best possible statistical error bars achievable from an experiment. With the further assumption of Gaussian-distributed signal and noise, Fisher matrices can be constructed from the specifications of CMBR experiments. However, given that the Fisher matrix formalism in practice asks how well an experiment can distinguish the true model of the Universe from other possible models, the results for the error bars on the parameters of these models will depend on which true model is chosen as input. Several groups have tried to estimate how well MAP and Planck will be able to extract information on the cosmological parameters from CMBR anisotropies using the Fisher matrix formalism [e.g. (6, 83, 80)]. I will quote the results from the work by (25), who were probably those who more thoroughly explored, within the simplest model context described before, the parameter degeneracies that may appear when the information is extracted from the CMBR data. They even included the possibility of a dependence with scale of the spectral index of density perturbations [see also (17)]. Regarding n_s, (25) conclude that among the four inflationary quantities it is the most readily measurable: just by using the CMBR temperature anisotropy angular power spectrum it will be possible to estimate it with an error of about 0.1 (1) through MAP and around 0.05 for Planck. Also using the CMBR polarisation information the error tends to be halved in the case of MAP and reduced by a factor of 5 for Planck. The other quantities, A_s, n_t, and A_t, will not be much better known after MAP and Planck, if just the CMBR temperature anisotropy spectrum is considered. Only by including polarisation information it will be possible to vastly improve the knowledge we have about the values taken by T/S and n_t.

The CMBR is expected to be partially linearly polarised [see (39) for a thorough review]. The polarisation signal can be decomposed into two separate and orthogonal components, the so-called E and B modes, with each mode having their own associated power spectra, which will depend in a different way on cosmological parameters. A cross-correlation signal between the temperature anisotropy and E-mode polarisation maps will also be present [see e.g. (44, 45)]. The knowledge of the polarisation signal will clearly contribute to improving the CMBR constraints on cosmological and perturbation parameters, but not equally on all of them. Those parameters that most gain from the use of the CMBR polarisation information are the ratio T/S, n_t and the optical depth . The reason is that only vector or tensor perturbations can give rise to B-mode polarisation, which can also arise from re-ionization of the intergalactic medium. However, vector perturbations rapidly decay in an expanding Universe, if all perturbations are primordial (e.g of inflationary origin). Consequently, while the total polarisation signal can help in the better estimation of all parameters, the power spectrum information associated with the B-mode polarisation will help particularly in bringing down the relative errors in the estimation of T/S, n_t and , most noticeably if the first and last are small (45, 25). However, from a practical point of view, it will not be easy to disentangle the CMBR polarisation signal from foreground polarisation sources, given the present lack of knowledge about their nature (72).

Interestingly, the polarisation signal can also provide one of the strongest tests known of the inflationary paradigm. Because polarisation is generated at the last scattering surface, models in which perturbations are causally produced, necessarily on sub-horizon scales, cannot generate a polarisation signal on angular scales larger than about 2°, approximately the horizon size at photon-baryon decoupling (71). Hence, polarisation correlations on such scales would indicate either a seemingly acausal mechanism for the generation of the perturbations or re-ionization at work. However, the two mechanisms can in principle be disentangled due to their different 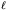dependence on large-angular scales (82). If what seems like acausality at work is proven, then it has been argued (54) that inflation provides the only mechanism through which it can be generated, by expanding initialy sub-horizon quantum fluctuations to super-horizon sizes. Unless it is postulated the existence of super-horizon perturbations since the begining of the Universe, which would give rise to a new initial conditions problem.

The measurement of the polarisation signal, by improving the estimation of T/S and n_t, will also provide the possibility of checking whether the so-called inflationary consistency relation holds, T/S - 7n_t [e.g. (56)]. If this is shown than single-field slow-roll models will receive a tremendous boost. Note, however, that there are inflationary models which do not predict such a relation.

Finally, we have until know only discussed what future constraints can be imposed on the cosmological parameters and the nature of the perturbations solely through the CMBR temperature anisotropy and polarisation signals. Clearly it would help if some of the degeneracies inherent to CMBR analysis could be broken by using large-scale structure data and direct measurements of the geometry and expansion rate of the Universe. The most promising large-scale structure data to be expected in the near future is the SDSS data, which together with the already well known local rich cluster abundance, provides a means of constraining the slope and normalisation of the present-day density power spectrum. Supernova type Ia and direct measurements of the Hubble constant will enable independent estimates of respectively the geometry and expansion rate of the Universe. Further, the evolution with redshift of the number density of rich galaxy clusters is a powerful method for determining the present total matter content, ₀, while gravitational lensing is able to impose interesting constraints on the value of a possible cosmological constant.