


This is a critical topic in regard to our effort to identify the source of the diffuse cosmic background at high galactic latitudes. The interstellar radiation field in the far ultraviolet has been directly measured by Henry, Anderson, and Fastie (1980), and it is found to be flat when expressed in units. Our spectrum, Figure 1, is also consistent with being flat longward of 1216 Å, and if the Voyager measurement is dismissed (we will briefly discuss its likely validity below), then on spectral grounds there obviously is strong reason to hope that the light that is seen at high galactic latitudes is simply starlight scattering from interstellar dust.
The two recent reviews of the diffuse ultraviolet background (Bowyer 1991; Henry 1991) reached very different conclusions concerning the subject of diffuse galactic light (starlight scattering from dust). New developments, and careful reconsideration of earlier discussions, allow us to resolve most of the controversy.
The most important new development is the measurement of the Henyey-Greenstein (1941) scattering parameter g in the ultraviolet by Witt et al. (1992). This parameter g characterizes the scattering pattern of the interstellar grains: g = 1 means complete forward scattering, g = 0 is isotropic scattering, and g = -1 represents complete back-scattering. The Henyey-Greenstein function has no physical basis, it is simply an heuristic tool for model-building. The reason that the value of g is so critical to our present concern is that if the albedo of the grains is high and if g is zero or at least has not too large a positive value, then the dust which is known to exist at high galactic latitudes (e.g., from IRAS cirrus observations) would backscatter sufficient light to account for the high latitude observations longward of 1216 Å.
The state of our knowledge of the value of g in 1991 can be gleaned from the excellent summary by Bowyer (1991, his Table 2). Values are widely divergent. The new observation by Witt et al. is of the nebula NGC 7023. The authors show an ultraviolet photograph, taken using UIT on the Astro mission, that shows the scattered light. Their analysis produces a fairly model-independent measurement of g = 0.75, which corresponds to very strong forward scattering. Their value for the albedo at 1400 Å is 0.65. In the light of all the controversy there has been over the value of g, it is important to note that Witt et al. indicate that their conclusion that guv > gvis is based on general radiative transfer principles and on the observational data alone. The value therefore should be quite secure. Let us now develop a simple model to use these values to predict what we should see at the highest galactic latitudes.
There is considerable dust at high galactic latitudes; for example
Hauser et al. (1984)
report, from their study of IRAS cirrus observations, that AV
= 0.1 mag at high latitudes.
Stark et al. 1992
show 2 x 1020 cm-2 as a typical column density
of neutral hydrogen at the highest galactic latitudes. Use of
EB-V = NHI/5 x 1021
(Knapp and Kerr 1974)
then gives AV = 0.12. If E1500-V / EB-V
= 5.3
(Bless and Savage 1972,
for  Oph), we then
get
Oph), we then
get  1500 Å = 0.921
A
1500 Å = 0.921
A = 0.3. We
adopt AV = 0.1
and
= 0.3. We
adopt AV = 0.1
and  1500 Å =
0.255. Also, there are many bright OB stars in or near the galactic
plane. For our simple model for the scattered light of these stars, we
integrate the
Henyey-Greenstein (1941)
scattering function
1500 Å =
0.255. Also, there are many bright OB stars in or near the galactic
plane. For our simple model for the scattered light of these stars, we
integrate the
Henyey-Greenstein (1941)
scattering function

| (4) |
over the back-scattering directions,
 / 2 to
/ 2 to
 , obtaining
, obtaining
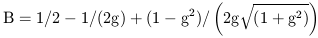
| (5) |
for B, the fraction of the scattered light that is backscattered. Our
model is, then, that
at high latitudes we expect a scattered intensity S = B G a
 , where G is the local
far-ultraviolet interstellar radiation field (~ 10,000 units:
Henry, Anderson, and
Fastie 1980),
a is the grain albedo (~ 0.65 at 1500 Å:
Witt et al. 1992),
and
, where G is the local
far-ultraviolet interstellar radiation field (~ 10,000 units:
Henry, Anderson, and
Fastie 1980),
a is the grain albedo (~ 0.65 at 1500 Å:
Witt et al. 1992),
and  = 0.255 is the
far-ultraviolet optical thickness of the high galactic latitude
scattering layer.
= 0.255 is the
far-ultraviolet optical thickness of the high galactic latitude
scattering layer.
For detailed study of scattered light at any particular region of the sky, one
unquestionably wants to use a detailed model. However, such models are
often complex and
not generally available. The only competing simple model is that of
Jura (1979),
which predicts the scattered light as a function of five variables: the
source function in the disk
(roughly our G),  0 (~ 0.85,
Joubert et al. 1983)
the optical thickness of the galaxy in
the ultraviolet, a, g, and the galactic latitude b. Use of
Jura's model is illustrated nicely in
Joubert et al. 1983.
We prefer our model: because of its simplicity (no evaluation of
an exponential integral is required); because it is valid for all values
of g (Jura's model
fails for large values of g); and because it does not give a
galactic latitude dependence:
the source function shows asymmetry in galactic longitude that is very
strong
(Henry 1977),
equaling that in latitude (just more than 78% of the source function originates
at |b|, < 21° while 78% of the source function originates at
180° < 1 < 360°).
0 (~ 0.85,
Joubert et al. 1983)
the optical thickness of the galaxy in
the ultraviolet, a, g, and the galactic latitude b. Use of
Jura's model is illustrated nicely in
Joubert et al. 1983.
We prefer our model: because of its simplicity (no evaluation of
an exponential integral is required); because it is valid for all values
of g (Jura's model
fails for large values of g); and because it does not give a
galactic latitude dependence:
the source function shows asymmetry in galactic longitude that is very
strong
(Henry 1977),
equaling that in latitude (just more than 78% of the source function originates
at |b|, < 21° while 78% of the source function originates at
180° < 1 < 360°).
A crude estimate using our model is, however, very revealing. In Table 1 we present (as a function of the Henyey-Greenstein scattering parameter g) the predicted high galactic latitude flux, from our model and from that of Jura (for b = 90°), using the values that were specified above for the necessary parameters.
| g | B | S(present) | S(Jura) | S(Onaka & Kodaira) |
| 0.98 | 0.004 | 7 | (-53) | |
| 0.90 | 0.023 | 38 | (7) | 41 |
| 0.80 | 0.051 | 84 | 81 | 90 |
| 0.75 | 0.067 | 110 | 118 | |
| 0.70 | 0.084 | 139 | 155 | 151 |
| 0.60 | 0.124 | 205 | 229 | 222 |
| 0.40 | 0.225 | 372 | 378 | 394 |
| 0.30 | 0.286 | 473 | 452 | 494 |
| 0.00 | 0.500 | 828 | 675 | 741 |
| -0.90 | 0.977 | 1619 | 1342 | |
The observed level of cosmic background reported at moderate and high latitudes by large numbers of observers is about 300 units (Henry 1991, and also Figure 1). A glance at Table 1 shows that if the value of g in the ultraviolet is, say, 0.7 or greater, then the cosmic high-latitude background is not scattered starlight and is presumably extragalactic.
The final column in Table 1 is the prediction at
high galactic latitudes of the sophisticated model of
Onaka and Kodaira
(1991),
which takes into account the variation
with galactic longitude of the galactic source function. We have used
a = 0.65 and
 = 0.255 in this application of the
Onaka and Kodaira model. Note the excellent
agreement among all these closely-related models.
= 0.255 in this application of the
Onaka and Kodaira model. Note the excellent
agreement among all these closely-related models.
That there is a very significant variation with galactic longitude of the source function for scattered light is of the greatest importance for interpretation of the diffuse galactic light. If one simply considers the distribution of the TD-1 stars (Figure 3), it is easy to mistakenly conclude that the source function is dependent on galactic latitude, and is independent of galactic longitude. That both of these conclusions are wrong, is demonstrated in Figure 4, which shows the integrated 1565 Å emission from the same stars that appear in Figure 3. There are profound effects due to absorption by the interstellar medium: also, the presence of Gould's Belt, which is tipped 19° with respect to the galactic plane, is very apparent. The model of Onaka and Kodaira, which takes some of these effects explicitly into account, will be very useful to us below.
If the background at high latitudes is not the back-scattered light of galactic plane stars, our rather exotic extragalactic model must perhaps be taken seriously. Before we do so, however, we must ask why Bowyer (1991) came to an opposite conclusion, concluding that most of the light, even at the highest galactic latitudes, is galactic in origin. Bowyer relied mostly on the result of Hurwitz, Bowyer, and Martin (1991) in reaching this conclusion. The result of Hurwitz, Bowyer, and Martin was that in the far ultraviolet, the interstellar grains have albedo 0.1 < a < 0.3 and Henyey-Greenstein scattering parameter is 0 < g < 0.4, which numbers differ drastically from the values quoted above, and which if correct would give a scattered starlight signal at high galactic latitudes of as much as 340 units. As long as there is any possibility that Hurwitz et al. are correct, our extragalactic model must be rejected.

|
Figure 3. The ultraviolet (1656 Å) stars from the TD-1 catalog. The north galactic pole is at the top, and the galactic center at the center. Galactic longitude increases to the left. The TD-1 stars are concentrated to the galactic plane, and they are reasonably evenly distributed in galactic longitude, when simply number of stars (shown) is considered. When the flux from these same stars is considered, instead, the result is dramatically different, as is shown in Figure 4 and 5. |
Unfortunately it is easy to show that the Hurwitz et al. analysis is suspect. It is based on the Berkeley UVX measurements. The locations of the nine UVX pointings are shown in Figure 5, superimposed on an unsaturated map of the source function for scattered light, the TD-1 stars (that is, Figure 5 is simply an unsaturated version of Figure 4, shifted in longitude). The Hurwitz et al. determination of the albedo relies mostly on their analysis of the signal seen during scan number 6 (see Figure 5), which was a scan from moderate galactic latitude to low galactic latitude in which the signal was interpreted as being saturated. We have organized Figure 5 so that this critical scan (at l = 135°) is centered in the figure. The potential flaw in their analysis is their assumed source function: they assumed that the interstellar radiation field arises from a smooth galactic-plane-parallel distribution of emitting (and absorbing) media. That this is not the case is dramatically apparent from Figures 4 and 5. The Hurwitz et al. model was scaled to match the TD-1 results for the sky-averaged interstellar radiation field at 1550 Å in the galactic plane. Now in fact 78% of the source function occurs in the hemisphere 180°-360°, far removed from scan 6. Thus, the light illuminating the dust of scan 6 was coming from quite different angles than assumed in their model. In particular, if g were large and positive (as the Witt et al. result suggests) then the dust in the direction of scan 6 could not be expected to backscatter significant amounts of light regardless of the value of the albedo. The Hurwitz et al. determination of g follows directly from their determination of the albedo a: they infer g from the high galactic latitude UVX observations, after fixing a. If a is low, then of course scattering must be isotropic if it is to provide the observed high latitude flux. If instead, the albedo is high as the analysis of Witt et al. 1992 suggests, then it follows from their data (and from our own UVX data, Murthy et al. 1990) that g must be large, or too high a flux would be seen at high latitudes.
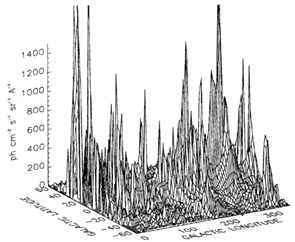
We emphasize that the asymmetry of the source function that is shown in Figure 5 is not in the least controversial (see, e.g., Gondhalekar et al. 1980). It has been clear for quite some time that to extract accurate values of a and g from mapping of the scattered light at moderate and high galactic latitudes will require rather sophisticated models. A beginning for such a model was used by Murthy, Henry, and Holberg (1991) in interpreting Voyager observations of the diffuse background at 1100 Å. In Figure 6 we show a preliminary version of their model, to re-emphasis our point that a sophisticated model is required. In particular, for g large (and the observation of Witt et al. suggests that g is indeed large), models that take into account individual stars clearly will be necessary.
[Since the above was written, we have performed a reanalysis of the UVX data (Henry and Murthy 1993) in which we show that these data are in fact quite compatible with values of the albedo ~ 0.65, and of the scattering asymmetry parameter g ~ 0.75, if an extragalactic component of 300 ±l 100 units exists.]

|
Figure 5. Integrated emission from the TD-1 stars. Dark regions in the figure are bright regions in the sky. The figure is linear and is just saturated at the darkest point. Also shown (numbered) are the regions observed by Johns Hopkins and Berkeley during the UVX mission. Targets are identified by number as specified in Murthy et al. (1989). Target 4 was a failed attempt to observe comet Halley, and produced no data useful for the present analysis. The north galactic pole is at the top, while the center of this Aitoff all-sky image is at galactic longitude l = 135° so as to demonstrate clearly how poorly illuminated the dust is at the location of UVX scan number six, called GRADIENT by Murthy et al. (1989). |
|
Figure 6. The model of ultraviolet scattered light of Murthy, Henry, and Holberg (1991) for the case a = 0.1, g = 0.9. The model scales linearly with a. The very sharp spikes are diffuse halos around stars that are predicted by this model. The figure shows only the scattered light; the source (the stars) is not shown (the source appears in Figures 3 and 4). |
A number of works (Bowyer 1991; Henry 1991) report correlations between purported measurements of the diffuse ultraviolet background and either galactic latitude or hydrogen column density. On this front, there has been a certain amount of progress since 1991. Wright (1992) has criticized the approach, often used, of noting that such correlations when extrapolated to zero column density always leave an unexplained residual. He points out that ionized and molecular hydrogen are also present and should have associated dust. Wright's reanalysis of the data of Fix et al. (1989) yields a = 0.42 ± 0.06, g = 0.44 ± 0.18, and an extragalactic component of no larger than 500 units. However, Witt and Petersohn (1994) have reconsidered Wright's analysis, and they find that the Fix et al. data show, instead, that the albedo a ~ 0.5, g ~ 0.9, and the extragalactic component is 300 ± 80 units.
Onaka and Kodaira (1991) have now reported in detail their rocket study of diffuse far-ultraviolet radiation at high galactic latitudes. They find, using their model that contains a dependence on galactic longitude of the source function for the scattered starlight, that
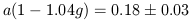
| (6) |
which agrees at the 2 level
with the a and g values of
Witt et al. (1991),
and which also
agrees within 2
level
with the a and g values of
Witt et al. (1991),
and which also
agrees within 2 with the values
of
Wright (1991).
They find that their regression line
of intensity against hydrogen column density intercepts the ordinate at
200 to 300 units, a somewhat lower value than appears in
Figure 1. A plot of their data
against csc b (as recommended by
Wright 1991)
yields an "extragalactic" component of about 400 units.
with the values
of
Wright (1991).
They find that their regression line
of intensity against hydrogen column density intercepts the ordinate at
200 to 300 units, a somewhat lower value than appears in
Figure 1. A plot of their data
against csc b (as recommended by
Wright 1991)
yields an "extragalactic" component of about 400 units.
Finally, Pérault et al. (1991) have re-examined the D2B-AURA measurements of the diffuse ultraviolet background of Joubert et al. (1983). The reexamination strongly justifies the skeptical attitude that was taken toward these data by Henry (1991). In the new analysis, the strong galactic latitude dependence of the "diffuse" flux is found to be dominated by direct starlight and starlight diffused in the instrument. They estimate that 1/2 to 2/3 of the ultraviolet flux is due to factors other than single scattering off dust. Pérault et al. believe that the major additional factor is light scattering in the instrument.
We now turn to the Voyager observations, and in particular, to the question of the reality of the jump in the high latitude background at 1216 Å.