7.2. Vacuum polarization and the value of

Zeldovich (1968), having demonstrated that the energy density of the vacuum was infinite at the one-loop level, suggested that after the removal of divergences, the `regularized' vacuum polarization contributed by a fundamental particle of mass m would be described by the expression

(81)

One can arrive at this result by means of the following argument: the vacuum consists of virtual particle-antiparticle pairs of mass m and separation = /mc. Although the regularized self-energy of these pairs is zero, their gravitational interaction is finite and results in the vacuum energy density _{vac vac} c² ~ (G m² / ) / ³ = Gm⁶ c⁴ / ⁴ corresponding to (81). (In terms of Feynman diagrams this corresponds to the energy associated with the two-loop vacuum graph shown in figure 13.) Substituting m m_e(m_p) we find that the electron (proton) mass gives too small (large) a value for . On the other hand, the pion mass gives just the right value [106] ⁽¹³⁾

(83)

Finally, a small value of can be derived from dimensionless fundamental constants of nature using purely numerological arguments. For instance, the fine structure constant e² / c 1/137 when combined with the Planck scale _P, suggests the relation [187]

(84)

Or, when expressed in terms of = (8 G / 3 H₀²) we get h² = 0.335, in excellent agreement with observations. In principle, could be some other fundamental constant, such as the `string constant' associated with superstring theory, which might enter into exponentially small expressions for of this type.

Figure 13. This figure shows the one-loop (a) and two-loop (b) vacuum diagrams which contribute towards the vacuum energy density discussed in sections 5 & 7.2 respectively.

¹³ The large difference between obtained using (81) for the proton and its observed value prompted Zeldovich to suggest that Fermi's weak interaction constant G_F might play a role in determining the vacuum energy, so that

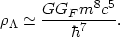
(82)

Although this leads to some improvement, for the proton is still several orders of magnnitude larger than its observed value. Back.