2.5. Petrosian index and magnitude

The Petrosian (1976, his Equation 7) function (R) is given as

(26) (27)

It is the average intensity within some projected radius R divided by the intensity at that radius. The logarithmic expression is written as

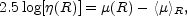
(28)

and is shown in Figure (6) for a range of profile shapes n.

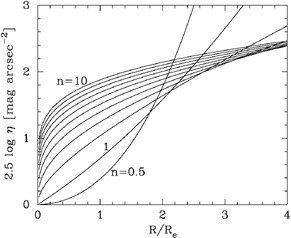Figure 6. The logarithm of the Petrosian function (R) (Equation 28) is shown as a function of normalised radius R / Re for Sérsic profiles having n = 0.5, 1, 2, 3,... 10.

This is a particular clever quantity because if every galaxy had the same stellar distribution, such as an R^1/4 profile, then a radius where the -function equalled some pre-defined, constant value would correspond to the same number of R_e for every galaxy. Moreover, such measurements are unaffected by such things as exposure-depth, galactic dust, and cosmological redshift dimming because they affect both surface brightness terms in Equation (28) equally. Even though it is possible to measure the Petrosian radius without ever assuming or specifying an underlying light-profile model, the actual form of the stellar distribution is implicitly incorporated into the Petrosian function and so cannot be ignored (as Figure 6 reveals).

It turns out the Petrosian function is equal to

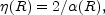
(29)

where (R) is given in Equation (24; Djorgovski & Spinrad 1981; Djorgovski, Spinrad & Marr 1984; Sandage & Perelmuter 1990, their Section IIa; Kjærgaard, Jorgensen, & Moles 1993). Thus

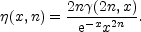
(30)

The flux within twice the radius R_P when 1 / (R_P) = 0.2 is often used to estimate an object's flux (e.g., Bershady, Jangren, & Conselice 2000; Blanton et al. 2001), as is the flux within 3R_P when 1 / (R_P) = 0.5 (e.g., Conselice, Gallagher, & Wyse 2002; Conselice et al. 2003). How well this works of course depends on the shape of the light-profile, and Figure (7) shows these approximations to the total luminosity as a function of the Sérsic index n. In the case of 2R_P when 1 / (R_P) = 0.2, one can see that profiles with n = 10 will have their luminosities under-estimated by 44.7% and those with n = 4 by only 17.1%. The situation is considerably worse when using 3R_P and 1 / (R_P) = 0.5. A prescription to correct for the missing light, beyond one's chosen aperture, is detailed in Graham et al. (2005).

Figure 7. Flux ratio, as a function of light-profile shape n, between the total luminosity Ltot and the Petrosian luminosity LPet inside (i) twice the radius RP where 1 / (RP) = 0.2 (solid curve) and (ii) thrice the radius RP where 1 / (RP) = 0.5 (dotted curve).