


11.2. Orbits in a Barred Galaxy
Knowledge of the stellar orbits in a barred galaxy can help us to
understand the dynamical properties of bars, their extension, and
pattern velocity. It is also fundamental to understanding resonances,
the behavior of the gas component, and therefore to explaining the
existence of rings.
Bars are three-dimensional components, and they can be modeled as
triaxial ellipsoids. Although the disks of spiral galaxies are very thin
components, with axis ratios around 10, bars can be thicker because of
vertical resonances (e.g.
Combes et al. 1990b),
and reveal box or
peanut shapes when seen edge-on. In a first approach, we will describe the
stellar orbits as if they were confined in the galaxy plane. This much
simpler approach gives already most of the characteristics of bar
dynamics and is sufficient to understand the gas behavior, since the
gas disk is much thinner.
First, let us recall the characteristics of orbits in an axisymmetric
potential  (r) in the plane
z = 0. A circular orbit has an angular
velocity
(r) in the plane
z = 0. A circular orbit has an angular
velocity  2 =
1 / r
d
2 =
1 / r
d  / dr. In linearizing the
potential in the neighborhood of a circular orbit, the motion of any
particle can be expressed in first order by an epicyclic oscillation,
of frequency
/ dr. In linearizing the
potential in the neighborhood of a circular orbit, the motion of any
particle can be expressed in first order by an epicyclic oscillation,
of frequency  ,
,
 2 =
d2
2 =
d2  /
dr 2 +
3
/
dr 2 +
3  2 = r
d
2 = r
d 2 / dr + 4
2 / dr + 4 2 6
2 6
The general orbit is therefore the combination of a circle and an
epicycle, or a rosette, since there is no rational relation between
the two periods.
The bar creates a bisymmetric gravitational potential, with a predominant
Fourier component m = 2, which rotates in the galaxy with the
pattern speed
 b. To be left
with a potential independent of time, where the
energy of particles is conserved, we must consider the orbits in the
rotating frame. The equivalent potential in this frame is then:
b. To be left
with a potential independent of time, where the
energy of particles is conserved, we must consider the orbits in the
rotating frame. The equivalent potential in this frame is then:
 eq =
eq =  (r,
(r,  ) - 1/ 2
) - 1/ 2  b2
r2 7
b2
r2 7
in cylindrical coordinates (r,  , z), for z = 0.
, z), for z = 0.
The energy of a particle (per unit mass) in this frame is
EJ = 1/ 2 v2 +  - 1/ 2
- 1/ 2  b2
r2. 8
b2
r2. 8
It is an integral called the Jacobi integral. It is expressed as a
function of the energy in the fixed frame E, as
EJ = E -  b Lz , where
Lz is the angular momentum, which is not an integral,
since the potential is non-axisymmetric.
b Lz , where
Lz is the angular momentum, which is not an integral,
since the potential is non-axisymmetric.
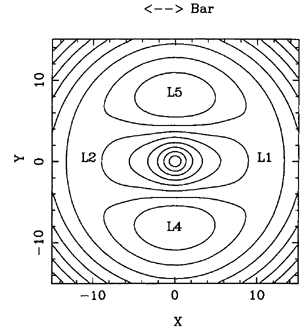
Figure 58. Equipotentials in the rotating
frame of the bar. This picture already provides a good hint of some of
the periodic orbit shapes.
|
The equipotentials in the rotating frame are given in
Figure 58. There exist 5 stationary points,
known as Lagrangian
points, L1 to L5, where the
derivative of  eq vanishes.
L3 is the central minimum, L4 and
L5 are maxima, and
L1 and
L2 are saddle points. Only L4 and
L5 are stable, i.e., a
particle can oscillate around them with the epicyclic frequency. The
four points, L1, L2,
L4, and L5 correspond to the
corotation resonance with the bar. Although they are not located at
the same galactocentric distance, they delineate an annular zone,
called the corotation zone.
eq vanishes.
L3 is the central minimum, L4 and
L5 are maxima, and
L1 and
L2 are saddle points. Only L4 and
L5 are stable, i.e., a
particle can oscillate around them with the epicyclic frequency. The
four points, L1, L2,
L4, and L5 correspond to the
corotation resonance with the bar. Although they are not located at
the same galactocentric distance, they delineate an annular zone,
called the corotation zone.
Lindblad Resonances
In the rotating frame, the effective angular velocity of a particle is
 ' =
' =  -
-  b. There exists
then regions in the galaxy
where
b. There exists
then regions in the galaxy
where  ' =
' =  / m, i.e. where the epicyclic
orbits close
themselves after m lobes. The corresponding stars are aligned with the
perturbation and closely follow it; they interact with it always with
the same sign, and resonate with it. These zones are the Lindblad
resonances, sketched with dashed circles in
Figure 59a.
According to the relative values of
/ m, i.e. where the epicyclic
orbits close
themselves after m lobes. The corresponding stars are aligned with the
perturbation and closely follow it; they interact with it always with
the same sign, and resonate with it. These zones are the Lindblad
resonances, sketched with dashed circles in
Figure 59a.
According to the relative values of  and
and  in a
realistic disk galaxy (Figure 59b), and
because the bar is a
bisymmetric perturbation, the most important resonances are those for
m = 2. An obvious resonance is corotation, for which
in a
realistic disk galaxy (Figure 59b), and
because the bar is a
bisymmetric perturbation, the most important resonances are those for
m = 2. An obvious resonance is corotation, for which  =
=
 b (
b ( ' = 0).
' = 0).
Figure 59. Lindblad resonances: a) resonant
orbits in the rotating frame of the bar; b) rotation curve (left scale,
km/s) and frequencies (right, km/s/kpc) used to model these orbits. The
pattern speed of the bar is indicated.

|
Periodic Orbits
Periodic orbits in the bar rotating frame are orbits that close on
themselves after one or more turns. Periodic orbits are the building
blocks which determine the stellar distribution function, since they
define families of trapped orbits around them. Trapped orbits are
non-periodic, but oscillate about one periodic orbit, with a similar
shape. The various families are best identified in surface of section
diagrams, first used by Poincaré as early as 1899. This method
consists of representing orbits of the 4-D phase-space (x, y, xdot,
ydot) only by their intersection points with any plane of phase
space, for instance xdot = 0, and projecting these points in a 2-D
space (for instance (y, ydot)). Periodic orbits appear then as single
points, while trapped orbits are represented by invariant curves. The
fact that points do not spread in space all over a given region, but
follow an invariant curve, is the consequence of the existence of
another integral besides Jacobi's. There can, however, exist irregular
orbits, for which the intersection points are spread all over a sea in
a stochastic manner. These ergodic orbits occur essentially when the
potential presents strong asymmetries. For realistic galaxy potentials,
ergodic orbits concern mainly regions outside corotation.
The periodic orbits are numerous (see the recent review by
Contopoulos &
Grosbøl 1989),
and we will describe here the most important ones for
the bar support, and for the ring formation mechanism:
- the x1 family (see
Figure 60) is the main family
supporting the bar. Orbits are elongated parallel to the bar, within
corotation. They can look like simple ellipses, and with energy
increasing, they can form a cusp, and even two loops at the extremities.
Figure 60. Shape of the main families of
periodic orbits in a barred galaxy, (see e.g.
Contopoulos &
Papayannopoulos 1980).
Some x1
orbits parallel to the bar are represented, together with
some x2 perpendicular orbits, inside CR.

|
- the x2 family exists only between the two inner
Lindblad resonances (ILR), when they exist. They are more round, and
elongated perpendicular to the bar. In the same category of 2 / 1 orbits
(which close after one turn and two epicycles), there are also the
x4
retrograde orbits, and the x3 unstable orbits, but
with less impact
for galaxies. The presence or not of nuclear rings appears to be
related to the existence of an ILR, as we have discussed in sections
9.7 and 9.8, and
which will be developed later. Even when there exist
two ILR's in the axisymmetric sense, the existence of the
x2 family
is not certain. When the bar is strong enough, the x2
orbits disappear. The bar strength necessary to eliminate the
x2 family
depends on the pattern speed
 b: the lower this speed, the
stronger the bar must be.
b: the lower this speed, the
stronger the bar must be.
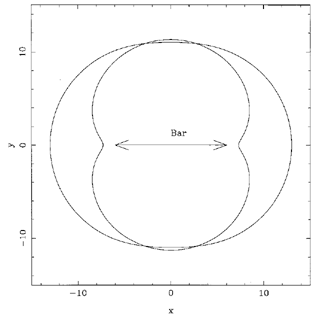
- Outside corotation, the 2 / 1 orbits that are run in the retrograde sense
in the rotating frame are perpendicular to the bar inside
the outer Lindblad resonance (OLR), and parallel to the bar slightly
outside (Figure 61). These orbits are also
discussed by
Kalnajs (1991).
Their shape reveals a characteristic figure-eight,
that is very similar to the dimpled shape of some outer rings in barred
galaxies (see section 4.1 and
Figure 18).
When the bar is strong, most orbits perpendicular to the bar between CR
and OLR become unstable. That is why this region is often depopulated
and, in real galaxies, never includes recent star formation.
Figure 61. Shape of the two main families
of periodic orbits near the outer Lindblad resonance (OLR). The orbit
inside OLR is perpendicular to the bar, while the orbits outside OLR are
parallel. The first one displays the characteristic dimpling that
corresponds to observations of R1 outer rings.
|
- When getting towards corotation (CR) all higher order resonances
 ' =
' =  / m with m
/ m with m
 3 are encountered. Spanning this
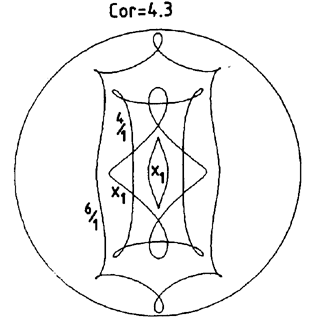
region, on the inner or outer side of CR, the periodic families 6 / 1 and
4 / 1 are displayed in Figure 62. The 4 / 1
resonant family is likely to play a role in the formation of inner rings.
3 are encountered. Spanning this
region, on the inner or outer side of CR, the periodic families 6 / 1 and
4 / 1 are displayed in Figure 62. The 4 / 1
resonant family is likely to play a role in the formation of inner rings.
Figure 62. The 4 / 1 and 6 / 1 periodic orbits,
from
Contopoulos &
Grosbøl (1989).
The bar is vertical, and the
circle indicates corotation.
|
- Finally, circulating around the Lagrangian points
L4 and L5, one
can distinguish the banana orbits or long period orbits (LPO), and the
short period orbits (SPO).
Consequences for the Bar Dynamics
The main family of orbits building the bar is the x1 family,
elongated along it. We can note however that the stable direct periodic
orbits have an orientation either parallel or perpendicular to the bar,
and that their orientation rotates by 90° at each Lindblad
resonance crossing. The x2 family, when it exists
between the two
ILR's, tends to weaken the bar, if the concerned region is large enough.
This suggests that a self-consistent bar can barely have one ILR; this
is the same conclusion reached from the swing amplification and
wave reflection mechanism to form the bar: the presence of ILR's tends
to destroy the bar.
The existence of a small zone between the two ILR's where the orbits are
not supporting the bar helps to understand the decoupling of a second
pattern, rotating faster than the main bar, in the nuclear parts of
some barred spiral galaxies. Examples of this decoupling into two
patterns can be found in the ``nuclear barred'' galaxies (e.g.
de Vaucouleurs 1975a;
Jarvis et al. 1988;
Shaw et al. 1993;
Buta & Crocker 1993).
The nuclear bar often occurs inside the nuclear ring,
corresponding to the ILR (see section 16).
Beyond corotation, the stable periodic orbits are also perpendicular to
the bar, which suggests that a self-consistent bar cannot extend far
beyond its own corotation. This has been verified in N-body simulations
of barred galaxies (e.g.,
Sellwood 1981;
Combes & Sanders 1981;
Sparke & Sellwood
1987):
the longest bars end just slightly before their corotation.
Approaching the corotation region, higher order resonances occur, and
the periodic orbits become more and more complex and squarish. The
observed shape of face-on early-type galaxy bars is indeed boxy, as was
shown for NGC 936 by
Kormendy (1983),
which compares very well
with N-body models (e.g.
Miller & Smith 1979).
This squarish shape might
measure the importance of the 4 / 1 resonance, which will in turn have a
strong consequence for inner rings encircling the bars.



 (r) in the plane
z = 0. A circular orbit has an angular
velocity
(r) in the plane
z = 0. A circular orbit has an angular
velocity  2 =
1 / r
d
2 =
1 / r
d  / dr. In linearizing the
potential in the neighborhood of a circular orbit, the motion of any
particle can be expressed in first order by an epicyclic oscillation,
of frequency
/ dr. In linearizing the
potential in the neighborhood of a circular orbit, the motion of any
particle can be expressed in first order by an epicyclic oscillation,
of frequency  ,
,



 ) - 1/ 2
) - 1/ 2 



 3 are encountered. Spanning this
region, on the inner or outer side of CR, the periodic families 6 / 1 and
4 / 1 are displayed in
3 are encountered. Spanning this
region, on the inner or outer side of CR, the periodic families 6 / 1 and
4 / 1 are displayed in 