| 
|



12.2. Formation of Rings
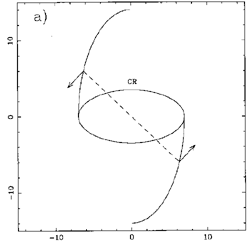
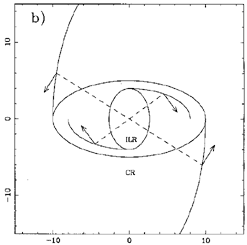
The most successful theory is that rings are formed by gas accumulation at Lindblad resonances, under the continuous action of gravity torques from the bar pattern. This long-term behavior has been revealed essentially by the sticky particle simulations. Since the potential is non-axisymmetric, tangential forces are exerted on the gas. If the gas is moving on circular orbits, these forces will cancel out by symmetry, and no net torque will be produced. This is also the case for orbits aligned parallel or perpendicular to the bar. On the contrary, when the gas is distributed in a spiral shape, orbits are inclined with respect to the symmetry axis of the bar. This results in a net torque on the gas, which is schematically represented in Figure 71. The torque changes sign at each resonance, where the spiral turns by 90°. Between the ILR and corotation, the torque is negative, while between CR and OLR, the torque is positive. These torques tend to depopulate the corotation region, and to accumulate gas towards the Lindblad resonances, in the shape of rings. Indeed, these rings then are aligned with the symmetry axis of the bar, and no net torque is acting on them.

| 
|
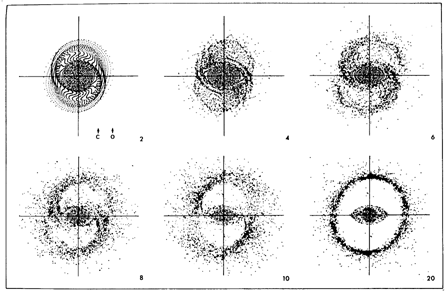
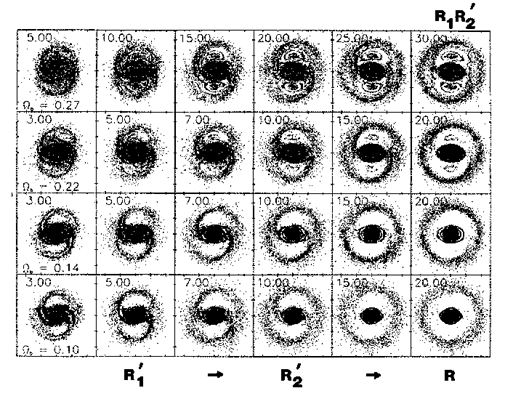
Schwarz (1981, 1984b) was the first to demonstrate the efficiency of these gravity torques to form rings, via numerical simulations. He developed a sticky particle code, where gas clouds are test particles in a fixed barred potential, moving like free particles, except when their trajectories cross and inelastic collisions are introduced. The reduced viscosity of the code allows us to see gas being driven outwards, from CR to OLR, instead of being dominated by viscous torques, that would have driven all the gas towards the center, irrespective of its location in the galaxy. Figure 72 shows in a very convincing way the formation of outer rings. The time-scale for this formation is quite long, being proportional to the rotation period in the concerned radii, which at OLR is of the order of a few times 109 yrs. Of course, this time-scale is longer for a weaker bar, with weaker torques.
|
Schwarz (1981) showed that two kinds of outer rings can be obtained, according to the initial distribution of the gas; both are shown in Figure 73. If the gas is more centrally concentrated, the outer ring is perpendicular to the bar, and takes the figure-eight shape characteristic of the 2 / 1 resonant periodic orbits slightly inside OLR. If the gas distribution is initially more extended, then the outer ring is parallel to the bar, and takes the shape of the other 2 / 1 resonant orbits slightly outside OLR. These two types of outer rings exist and have been called R'1 and R'2, respectively, by Buta (1986a) and Buta & Crocker (1991); they were illustrated and discussed in section 4.1. It is noteworthy that Schwarz found the parallel-aligned variety of outer ring to be more robust than the perpendicular-aligned variety, stating that it was always favored when there was even a little gas outside the OLR. However, as was shown by Buta (1995), in real barred galaxies the R'1 variety is more commonly observed than the R'2 variety.
|
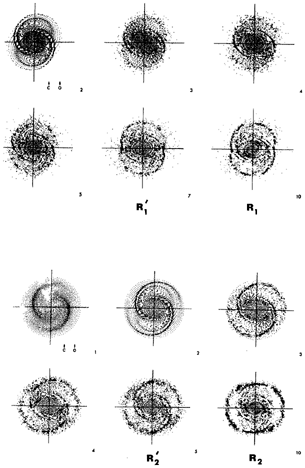
The formation of outer rings and pseudorings has also been investigated by Byrd et al. (1994), who extended Schwarz's simulations by using a larger number of particles, an improved collision scheme which tracked individual particles to determine when they collide, and different rotation curves. They found that outer rings (mainly those of type R'2) are more easily produced with fast bars (since there is then enough matter at OLR). Besides also verifying Schwarz's results, this study identified a possible evolutionary sequence whereby outer rings begin as R'1 pseudorings, evolve into R'2 pseudorings, and then evolve into detached closed outer rings (see Figure 74). This study also helped to eludicate the possible origin of the R1R'2 morphology which was discussed in section 4.1. This combination morphology of the two ring types was not found in Schwarz's simulations, but traces of it are seen in Byrd et al.'s highest pattern speed simulation (see upper left frame of Figure 74). The general lack of formation of this type of structure in simulations might only be due to the non-introduction of star formation out of the gas in the models. Indeed, the ring formation only involves gas. The stellar component is not following the accumulation of gas at resonances, since the stellar orbits are parallel to the symmetry axis of the bar. But new stars will be formed out of the gas in rings, a process that will grow more effective as the gas density there is enhanced. When the gas is then locked up into stars, it will no longer be as sensitive to the gravity torques as in the simulations, and will stay in the rings formed. The remaining gas will then loose a little energy by dissipation, and falls on the next orbit in energy, which is inside OLR forming the R1 ring.
|
In support of these ideas, Byrd et al. (1994) pointed out the existence of a subtle dichotomy in the R1R'2 galaxy IC 1438. In this galaxy, the R1 component is most prominent in a near-IR image, while the R'2 component is most prominent in a blue light image or a B - I color index map. The dichotomy suggests that the R1 component formed first and left behind a stellar remnant. Such an arrangement seems to support the evolutionary sequence shown in Figure 74, and the need for allowing for star formation to explain the R1R'2 morphology in some cases. Crocker et al. (1996) also found evidence in support of the R1 -> R2 sequence in the distributions of HII regions in several early-type ringed galaxies with strong stellar R1 components (see section 9.5).
With regard to the periodic OLR orbits that lead to the R1 and R2 rings, Kalnajs (1991) has proposed that the Hyades and Sirius star streams may be connected with crossing periodic orbits near the OLR in our Galaxy. The Sirius stream would be outside the OLR while the Hyades stream would be inside. This is interesting, and not unlikely given the location of the Sun at a radius comparable to some outer rings in galaxies.
A sticky-particle simulation which shows an especially well-defined inner ring was illustrated by Simkin et al. (1980; see Figure 75). Schwarz (1984a, b) proposed that the inner ring in this and his other simulations corresponds to gas accumulated at the inner 4 / 1 ultraharmonic resonance (UHR) just slightly inside corotation. In its motion towards the ILR due to the bar torque, some gas can stop at the UHR, where the orbits are parallel to the bar. The gas gathers into the largest periodic orbit near UHR which does not cusp and which does not intersect another orbit. The symmetry of the orbit then shields it from the torques. Formation of a UHR ring depends on the bar field being strong enough near UHR to cause spiral shocks. When the bar field near UHR is weak, the periodic orbits at UHR do not intersect, and no ring forms there.

|
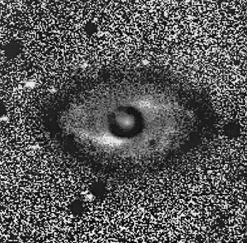
The simulated inner rings tend to be rather oval and sharp-cornered with a rough diamond shape and a pointy major axis. The UHR rings begin as pseudorings and develop a four-part structure before evolving into a pointy oval (as in Figure 75). Evidence that the UHR is the right interpretation for real inner rings and pseudorings comes from the shape of some of these rings, which are in general elongated along the bar (section 9.3), and which are sometimes pointy-ended as in NGC 6782 (see Figure 76) or sectioned into four parts as in NGC 1433 (Buta 1986b, see Figure 3). From Figure 75, the inner pseudoring in NGC 1433 could be in an earlier stage of development than the pointy oval inner ring in NGC 6782 or the inner ring in NGC 3081 (Figure 49). We have already pointed out, however, that the inner rings in these three galaxies are extreme in shape. Schwarz (1985) investigated a way of making the inner rings in his simulations come out rounder than in Figure 75. By adding a lens-like component to the background, he was able to achieve rounder, less pointy inner rings.
|
The ratio of radii of outer and inner rings in most barred galaxies that exhibit both types agrees well for R(OLR) / R(UHR) for flat rotation curves: the expected ratio is 2.6 compared to the observed average ratio of 2.2 (Athanassoula et al. 1982; Buta 1995, see Figure 27). We can see this characteristic ratio in the simulation in Figure 75.
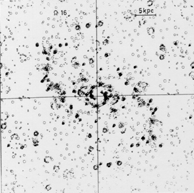
Nuclear rings are even more easily formed, since the time-scale is quite short: a few 107 to 108 yrs (see Figure 77). They require the existence of at least one ILR. But various shapes can be obtained, according to the assumed pattern speed of the bar: the nuclear ring can be parallel to the bar, tilted in the leading direction, or even perpendicular to the bar (Schwarz 1984b; Combes & Gerin 1985; Byrd et al. 1994). Its ellipticity can take a wide range of values. In the long run, the inner rings are depleted to the benefit of nuclear rings. The persistence of inner rings in observed galaxies suggests that fresh gas is continuously provided to the galaxy, either from the gas released from high mass star formation, or by continuous infall of external gas.
| 
|

|
When there exist two ILR's, the gas accumulates at the inner ILR (Combes & Gerin 1985; Piner et al. 1995), which can be very close to the center (inside 1 kpc, typically). This can be explained either by the gas dissipation, since viscous torques are becoming less negligible towards the center, or by gravity torques. In fact, the transient spiral that forms between the two ILR's is a mixture of leading and trailing waves, and close to the inner ILR, the leading one dominates. The gas distribution, which is very concentrated in the center, favors the leading wave, and the dominant torque is negative.
The nuclear rings are so small that they represent a huge accumulation
of gas, with large surface densities. This leads to starbursts that are
frequently observed as hot spots of giant HII regions, or non-thermal
radio emission: typical examples are NGC 1097
(Hummel et al. 1984;
Gerin et al. 1988), NGC 4314
(Wakamatsu & Nishida
1980;
Garcia-Barreto et
al. 1991),
and others discussed in sections
9.5 and 9.7.
Some nuclear rings are so small (of the order of 100 pc scale,
e.g. M82,
Lo et al. 1987)
that viscous torques, or even dynamical
friction of giant molecular clouds (of mass up to 107
M )
on the bulge could produce large gas flows towards the center, and fuel
an active nucleus. A correlation has been found between the existence
of bars and activity in the nuclei (section
8), and in particular the
presence of a nuclear ring (e.g., the jets in NGC 1097,
Wolstencroft et
al. 1984).
The presence of two ILR's, in shifting the gas response with
respect to the stellar bar, enhances the negative gravity torques on
the gas, that is now leading the bar. This considerably enhances the
nuclear gas flows
(Combes 1994).
When the mass concentration is high
enough in the center, another pattern can develop inside the nuclear
ring, as will be described in section 16.
)
on the bulge could produce large gas flows towards the center, and fuel
an active nucleus. A correlation has been found between the existence
of bars and activity in the nuclei (section
8), and in particular the
presence of a nuclear ring (e.g., the jets in NGC 1097,
Wolstencroft et
al. 1984).
The presence of two ILR's, in shifting the gas response with
respect to the stellar bar, enhances the negative gravity torques on
the gas, that is now leading the bar. This considerably enhances the
nuclear gas flows
(Combes 1994).
When the mass concentration is high
enough in the center, another pattern can develop inside the nuclear
ring, as will be described in section 16.