4.2. Measuring the Hubble Constant
As stated previously, the expansion of the universe follows the Hubble
law given by Eq. (6). Observationally, we measure the recession
velocity as a redshift, z, in the light from the supernova
(vr = cz). Since every type Ia supernovae has
about the same absolute
magnitude, Eq. (26), the apparent magnitude provides an indirect measure
of its distance. Therefore, for nearby supernovae (z
0.3) the
Hubble Law is equivalent to a relationship between the redshift and the
magnitude. Inserting (26) into (24), using (6), and applying to the
current epoch, yields the redshift-magnitude relation
 |
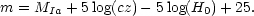(27) |
Defining the z = 0 intercept as
 |
(28) |
we can write equation (27) as
 |
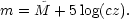(29) |
As shown in Fig. 1, low-redshift data can be
used to find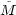 
and
Eq. (28) to solve for the Hubble constant. Studies on type Ia supernova
[12]
consistently suggest a value for the Hubble constant of
about 63 km s-1 Mpc-1.
 |
Figure 1. Using low-redshift supernovae to
determine the Hubble
constant. The data points are from Ref. 12. The inset graph shows a
close-up view of the data and the best-fit line. The best-fit line
determines the intercept
|
The result for H0, found from low-redshift supernovae, tends to set the lower bound when compared with other methods for obtaining H0. For example, if the distances to enough galaxies can be accurately found, then the Hubble law can be used directly to obtain a value of H0. This has partly been the goal of the Hubble Space Telescope Key Project. [13] This project has shown that a careful consideration of the type Ia supernova results in combination with the other methods for obtaining H0 produces what has become a widely accepted value for the Hubble constant
 |
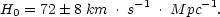(30) |
The value given in Eq. (30) is the one that we shall adopt in this paper.