4. THE NATURE OF DARK MATTER

By the end of 1970s most objections against the dark matter hypothesis were rejected. In particular, luminous populations of galaxies have found to have lower mass-to-light ratios than expected previously, thus the presence of extra dark matter both in galaxies and clusters has been confirmed. However, there remained three problems:

• It was not clear how to explain the Big Bang nucleosynthesis constraint on the low density of matter, and the smoothness of the Hubble flow.

• If the massive halo (corona) is not stellar nor gaseous, of what stuff is it made of?

• And a more general question: in Nature everything has its purpose. If 90% of matter is dark, then there must be a reason for its presence. What is the role of dark matter in the history of the Universe?

First we shall discuss baryons as dark matter candidates.

4.1. Nucleosynthesis constraints on the amount of baryonic matter

According to the Big Bang model, the Universe began in an extremely hot and dense state. For the first second it was so hot that atomic nuclei could not form - space was filled with a hot soup of protons, neutrons, electrons, photons and other short-lived particles. Occasionally a proton and a neutron collided and sticked together to form a nucleus of deuterium (a heavy isotope of hydrogen), but at such high temperatures they were broken immediately by high-energy photons (Schramm & Turner 1998).

When the Universe cooled off, these high-energy photons became rare enough that it became possible for deuterium to survive. These deuterium nuclei could keep sticking to more protons and neutrons, forming nuclei of helium-3, helium-4, lithium, and beryllium. This process of element-formation is called "nucleosynthesis". The denser proton and neutron "gas" is at this time, the more of these light elements will be formed. As the Universe expands, however, the density of protons and neutrons decreases and the process slows down. Neutrons are unstable (with a lifetime of about 15 minutes) unless they are bound up inside a nucleus. After a few minutes, therefore, the free neutrons will be gone and nucleosynthesis will stop. There is only a small window of time in which nucleosynthesis can take place, and the relationship between the expansion rate of the Universe (related to the total matter density) and the density of protons and neutrons (the baryonic matter density) determines how much of each of these light elements are formed in the early Universe.

According to nucleosynthesis data baryonic matter makes up 0.04 of the critical cosmological density (Fig. 8). Only a small fraction, less than 10%, of the baryonic matter is condensed to visible stars, planets and other compact objects. Most of the baryonic matter is in the intergalactic matter, it is concentrated also in hot X-ray coronas of galaxies and clusters.

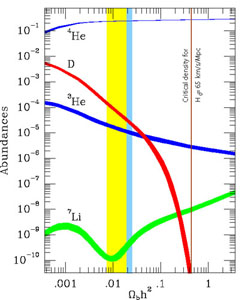Figure 8. The big-bang production of the light elements. The abundance of chemical elements is given as a function of the density of baryons, expressed in units of b h2 (horizontal axis). Predicted abundances are in agreement with measured primeval abundances in a narrow range of baryon density (Schramm & Turner 1998).

4.2. Baryonic Dark Matter

Models of the galaxy evolution are based on stellar evolution tracks, star formation rates (as a function of time), and the initial mass function (IMF). For IMF the Salpeter (1955) law is usually used:

where m is the mass of the forming star, and a and n are parameters. This law cannot be used for stars of arbitrary mass, because in this case the total mass of forming stars may be infinite. Thus we assume that this law is valid in the mass interval m₀ to m_u (the lower and upper limit of the forming stars, respectively).

Early models of physical evolution of galaxies were constructed by Tinsley (1968) and Einasto (1972). These models show that the mass-to-light ratio M_i / L_i of the population i depends critically on the lower limit of the IMF, m₀. It is natural to expect that in similar physical conditions (the metalicity of the gas in star formation regions) the lower mass limit of forming stars has similar values (Fig. 9). An independent check of the correctness of the lower limit is provided by homogeneous stellar populations, such as star clusters. Here we can assume that all stars were formed simultaneously, the age of the cluster can be estimated from the HR diagram, and the mass derived from the kinematics of stars in the cluster. Such data are available for old metal-poor globular clusters, for relatively young medium-metal-rich open clusters as well as for metal-rich cores of galaxies. This check suggests that in the first approximation for all populations similar lower mass limits (m₀ = 0.05 ... 0.1 M) can be used; in contracting gas clouds above this limit the hydrogen starts burning, below not. Using this mass lower limits we get for old metal-poor halo populations M_i / L_i 1, and for extremely metal-rich populations in central regions of galaxies M_i / L_i =10 ... 30, as suggested by the central velocity dispersion in luminous elliptical galaxies. For intermediate populations (bulges and disks) one gets M_i / L_i = 3 ... 10, see Fig. 9. Modern data yield slightly lower values, due to more accurate measurements of velocity dispersions in the central regions of galaxies, as suggested in pioneering studies by Faber & Jackson (1976), Faber et al. (1977), and more accurate input data for evolution models.

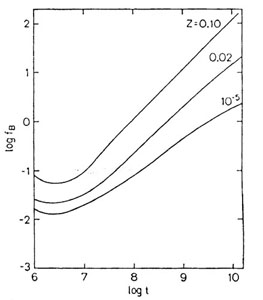Figure 9. The evolution of mass-to-light ratios fB of galactic populations of different metal abundance Z (Einasto 1972). The age of population t is expressed in years. An identical lower limit of IMF 0.1 M was accepted.

For this reason it is very difficult to explain the physical and kinematical properties of a stellar dark halo. Dark halo stars form an extended population around galaxies, and must have a much higher velocity dispersion than the stars belonging to the ordinary halo. No fast-moving stars as possible candidates for stellar dark halos were found (Jaaniste & Saar 1975). If the hypothetical population is of stellar origin, it must be formed much earlier than all known populations, because known stellar populations of different age and metalicity form a continuous sequence of kinematical and physical properties, and there is no place where to include this new population into this sequence. And, finally, it is known that star formation is not an efficient process - usually in a contracting gas cloud only about 1 %  of the mass is converted to stars. Thus we have a problem how to convert, in an early stage of the evolution of the Universe, a large fraction of the primordial gas into this population of dark stars. Numerical simulations suggest, that in th early universe only a very small fraction of gas condenses to stars which ionize the remaining gas and stop for a certain period further star formation (Cen 2003, Gao et al. 2005b).

Stellar origin of dark matter in clusters was discussed by Napier & Guthrie (1975); they find that this is possible if the initial mass function of stars is strongly biased toward very low-mass stars. Thorstensen & Partridge (1975) discussed the suggestion made by Truran & Cameron (1971) that there may have been a pre-galactic generation of stars (called now population III), all of them more massive than the Sun, which are now present as collapsed objects. They conclude that the total mass of this population is negligible, thus collapsed stars cannot make up the dark matter.

Recently weak stellar halos have been detected around several nearby spiral galaxies at very large galactocentric distances. For instance, a very weak stellar halo is found in M31 up to distance of 165 kpc (Gilbert et al. 2006, Kalirai et al. 2006). The stars of this halo have very low metalicity, but have anomalously red colour. The total luminosity and mass of these extended halos is, however, very small, thus these halos cannot be identified with the dark halo.

Gaseous coronas of galaxies and clusters were discussed in 1970s by Field (1972), Silk (1974), Tarter & Silk (1974), Komberg & Novikov (1975) and others. The general conclusion from these studies was that gaseous coronas of galaxies and clusters cannot consist of neutral gas since the intergalactic hot gas would ionise the coronal gas. On the other hand, a corona consisting of hot ionised gas would be observable. Modern data show that part of the coronal matter around galaxies and in groups and clusters of galaxies consists indeed of the X-ray emitting hot gas, but the amount of this gas is not sufficient to explain the flat rotation curves of galaxies (Turner 2003).

The result of these early discussions of the nature of dark halos were inconclusive - no appropriate candidate was found. For many astronomers this was an argument against the presence of dark halos.

4.3. Non-baryonic Dark Matter and fluctuations of the CMB radiation

Already in 1970s suggestions were made that some sort of non-baryonic elementary particles, such as massive neutrinos, magnetic monopoles, axions, photinos, etc., may serve as candidates for dark matter particles. There were several reasons to search for non-baryonic particles as a dark matter candidate. First of all, no baryonic matter candidate did fit the observational data. Second, the total amount of dark matter is of the order of 0.2-0.3 in units of the critical cosmological density, while the nucleosynthesis constraints suggest that the amount of baryonic matter cannot be higher than about 0.04 of the critical density.

A third very important observation was made which caused doubts to the baryonic matter as the dark matter candidate. In 1964 Cosmic Microwave Background (CMB) radiation was detected. This discovery was a powerful confirmation of the Big Bang theory. Initially the Universe was very hot and all density and temperature fluctuations of the primordial soup were damped by very intense radiation; the gas was ionized. But as the Universe expanded, the gas cooled and at a certain epoch called recombination the gas became neutral. From this time on density fluctuations in the gas had a chance to grow by gravitational instability. Matter is attracted to the regions were the density is higher and it flows away from low-density regions. But gravitational clustering is a very slow process. Model calculations show that in order to have time to build up all observed structures as galaxies, clusters, and superclusters, the amplitude of initial density fluctuations at the epoch of recombination must be of the order of 10^-3 of the density itself. These calculations also showed that density fluctuations are of the same order as temperature fluctuations. Thus astronomers started to search for temperature fluctuations of the CMB radiation. None were found. As the accuracy of measurement increased, lower and lower upper limits for the amplitude of CMB fluctuations were obtained. In late 1970s it was clear that the upper limits are much lower than the theoretically predicted limit 10^-3.

Then astronomers recalled the possible existence of non-baryonic particles, such as heavy neutrinos. This suggestion was made independently by several astronomers (Cowsik & McClelland 1973, Szalay & Marx 1976, Tremaine & Gunn 1979, Doroshkevich et al. 1980b, Chernin 1981, Bond et al. 1983) and others. They found that, if dark matter consists of heavy neutrinos, then this helps to explain the paradox of small temperature fluctuations of the cosmic microwave background radiation. This problem was discussed in a conference in Tallinn in April 1981. Recent experiments by a Moscow physicist Lyubimov were announced, which suggested that neutrinos have masses. If so, then the growth of perturbations in a neutrino-dominated medium can start much earlier than in a baryonic medium, and at the time of recombination perturbations may have amplitudes large enough for structure formation. The Lyubimov results were never confirmed, but it gave cosmologists an impulse to take non-baryonic dark matter seriously. In the conference banquet Zeldovich gave an enthusiastic speech: "Observers work hard in sleepless nights to collect data; theorists interpret observations, are often in error, correct their errors and try again; and there are only very rare moments of clarification. Today it is one of such rare moments when we have a holy feeling of understanding the secrets of Nature." Non-baryonic dark matter is needed to start structure formation early enough. This example illustrates well the attitude of theorists to new observational discoveries - the Eddington's test: "No experimental result should be believed until confirmed by theory" (cited by Mike Turner 2000). Dark matter condenses at early epoch and forms potential wells, the baryonic matter flows into these wells and forms galaxies (White & Rees 1978).

The search of dark matter can also be illustrated with the words of Sherlock Holmes "When you have eliminated the impossible, whatever remains, however improbable, must be the truth" (cited by Binney & Tremaine 1987). The non-baryonic nature of dark matter explains the role of dark matter in the evolution of the Universe, and the discrepancy between the total cosmological density of matter and the density of baryonic matter, as found from the nucleosynthesis constraint. Later studies have demonstrated that neutrinos are not the best candidates for the non-baryonic dark matter, see below.

4.4. Alternatives to Dark Matter

The presence of large amounts of matter of unknown origin has given rise to speculations on the validity of the Newton law of gravity at very large distances. One of such attempts is the Modified Newton Dynamics (MOND), suggested by Milgrom & Bekenstein (1987), for a discussion see Sanders (1990). Indeed, MOND is able to represent a number of observational data without assuming the presence of some hidden matter. However, there exist several arguments which make this model unrealistic.

First of all, in the absence of large amounts of non-baryonic matter during the radiation domination era of the evolution of the Universe it would be impossible to get for the relative amplitude of density fluctuations a value of the order of 10^-3, needed to form all observed structures.

Second, there exist numerous direct observations of the distribution of mass, visible galaxies and the hot X-ray gas, which cannot be explained in the MOND framework. One of such examples is the "bullet" cluster 1E 0657-558 (Clowe et al. 2004, Markevitch et al. 2004, Clowe et al. 2006a), shown in Fig. 10. This is a pair of galaxy clusters, where the smaller cluster (bullet) has passed the primary cluster almost tangentially to the line of sight. The hot X-ray gas has been separated by ram pressure-stripping during the passage. Weak gravitation lensing yields the distribution of mass in the cluster pair. Lensing observations show that the distribution of matter is identical with the distribution of galaxies. The dominant population of the baryonic mass is in X-ray gas which is well separated from the distribution of mass. This separation is only possible if the mass is in the collisionless component, i.e. in the non-baryonic dark matter halo, not in the baryonic X-ray gas.

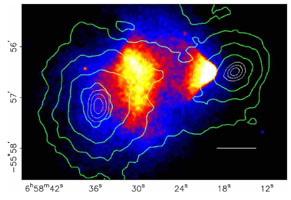Figure 10. Images of the merging 'bullet' cluster 1E0657-558. The left panel shows a direct image of the cluster obtained with the 6.5-m Magellan telescope in the Las Campanas Observatory, the right panel is a X-ray satellite Chandra image of the cluster. Shock waves of the gas are visible, the gas of the smaller 'bullet' cluster (right) lags behind the cluster galaxies. In both panels green contours are equidensity levels of the gravitational potential of the cluster, found using weak gravitational lensing of distant galaxies. The white bar has 200 kpc/h length at the distance of the cluster. Note that contours of the gravitational potential coincide with the location of visible galaxies, but not with the location of the X-ray gas (the dominant baryonic component of clusters) (Clowe et al. 2006a) (reproduced by permission of the AAS and authors).