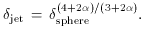4.4 Brightness Temperature Calculations and SSC Models

The smooth nonthermal radio-through-infrared continuum emission in radio-loud AGN is probably synchrotron radiation, i.e., emission from relativistic electrons moving in a magnetic field. Some of the synchrotron photons will be inverse Compton scattered to higher energies by the relativistic electrons, which is known as the synchrotron self-Compton (SSC) process. For some radio-loud AGN the synchrotron radiation density inferred from the observed radio power and angular size predicts SSC X-rays well in excess of the observed X-ray flux (Marscher et al. 1979; Ghisellini et al. 1993), which is called the ``Compton catastrophe'' (Hoyle et al. 1966). A related (but not equivalent) statement is that extremely rapid radio variability in some blazars (Quirrenbach et al. 1992) implies brightness temperatures, T_B = Ic² / (2k²), where k is Boltzman's constant, larger than the 10¹² K limit (Kellermann and Pauliny-Toth 1969; see also Singal and Gopal-Krishna 1985 and Readhead 1994, who suggest a limit T_B 10¹¹ K appropriate to the equipartition of magnetic field and relativistic electron energy densities).

It follows that the true synchrotron photon density must be lower than observers infer by assuming isotropy. The strong anisotropy and shortened time scales caused by relativistic beaming can account naturally for the Compton catastrophe (or non-catastrophe, as it happens). A lower limit to the Doppler factor, , which characterizes these effects (Appendix B) can be estimated from the ratio of predicted to observed SSC flux (Jones et al. 1974; Marscher et al. 1979). In the case of a spherical emission region of observed angular diameter _d, moving with Doppler factor _sphere, the limit is (Ghisellini et al. 1993):

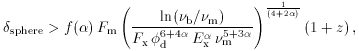(2)

where _d is in milliarcseconds, _m is the observed self-absorption frequency of the synchrotron spectrum in GHz, F_m is the observed radio flux at _m in Jy, E_x and F_x are the observed X-ray energy and flux in keV and Jy respectively, and _b is the observed synchrotron high frequency cut-off. The function f(), where is the spectral index of the optically thin synchrotron emission, depends only weakly on the various assumptions used by different authors (see discussion in Urry 1984) and has the approximate value f() 0.08 + 0.14 (Ghisellini 1987). If the radio source is a continuous jet, which is perhaps more realistic (Appendix B, case p = 2 + ), then (Ghisellini et al. 199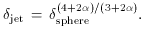3):

(3)

For a continuous jet compared to a single blob, therefore, the same observed quantities imply a higher Doppler beaming factor (for > 1).

The limit in Eq. (2) has been calculated for many radio-loud AGN (Marscher et al. 1979; Madejski and Schwartz 1983; Madau et al. 1987), with the result that has lower limits both larger and smaller than unity, depending on the AGN. One complication is that the angular size (_d) is a function of observation frequency and so is to some extent arbitrary. A self-consistent approach is to use, in Eq. (2), the observing frequency as _m and the flux and angular size (preferably measured with VLBI) at that frequency as F_m and _d, respectively.

For ~ 100 radio sources for which the VLBI size of the radio-emitting core is published, Eq. (2) gives > 1 for a large fraction of BL Lacs and essentially all FSRQ (Ghisellini et al. 1993). That is, blazars for which appropriate data exist do appear to have relativistically beamed emission. Similarly, using variability time scales to infer Doppler factors from the condition T_{B, max} < 10¹² K gives > 1 for a number of blazars (Teräsranta and Valtaoja 1994). The latter values for BL Lacs are somewhat low compared to the SSC calculation, but if the equipartition brightness temperature is more appropriate (Singal and Gopal-Krishna 1985; Readhead 1994), the derived Doppler factors increase by a factor of 2-3, since = [T_{B, obs} / T_{B, max}]^1/3.