4.2. A worked example

The easiest way to see what is going on is to work through a specific example, the m²² / 2 potential which we already saw in Section 3.7. We'll see that we don't even have to solve the evolution equations to get our predictions.

1. Inflation ends when = 1, so _end m_Pl / (4).
2. We're interested in 60 e-foldings before this, which from Eq. (37) gives ₆₀ 3m_Pl.
3. Substitute this in:

   

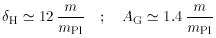
4. Reproducing the COBE result requires [19] _H 2 x 10^-5 (provided A_G << _H), so we need m 10^-6m_Pl.

Because the required value of m is so small, that means it is easy to get sufficient inflation to solve the cosmological problems if one only requires the classicality condition V < m_Pl⁴. Since that condition implies only that < m_Pl² / m 10⁶m_Pl, and as N_tot 2² / m_Pl², we can get up to about 10¹³ e-foldings in principle. This compares extremely favourably with the 70 or so actually required.