3.3.8. Core Profiles

This review of elliptical-ga1axy brightness profiles has so far been based on arbitrary analytic fitting functions and departures from them. The reason for this is that we have no secure model of the profile shape in the absence of an understanding of the velocity anisotropies. This is less true in galaxy cores. It is not implausible that violent relaxation may have produced cores that are isothermal (e.g., King 1966, 1978). However, this may be an oversimplification (1) if there exist large nuclear mass concentrations such as black holes, or (2) if the dynamics of galaxy formation were sufficiently complicated. For example, cores could be triaxial (Schwarzschild 1980a; Binney 1980a, 1981b, 1982a), as observed in the nucleus of M31 (see below). There has been no systematic study of core shapes or velocity anisotropies. I wish to note this important problem, but will not discuss it further. I will review recent work on the brightness profiles of cores, which shows that only some of them are isothermal. The rest have non-zero brightness gradients near the center, an effect which may indicate the presence of a nuclear "black hole", but which can also be interpreted in other ways. As emphasized by Schweizer (1979, 1981a) and others, atmospheric seeing has a major impact on our ability to detect and interpret interesting features in galaxy cores. This problem will also be discussed in detail. Previous reviews on cores have been published by Faber (1980) and by Illingworth (1980).

Examples of isothermals fitted to galaxy profiles are given in Figure 19. The upper two galaxies are among the very few (Schweizer 1979) in which the cores are large enough that seeing is not a major factor. NGC 4472 is well described by an isothermal. NGC 4636 is not isothermal unless there are large errors in the photometry. (The King 1978 data are the best photographic photometry of cores in the literature, but they need to be verified using, e.g., a CCD.) Many galaxies with smaller cores are well described by seeing-convolved isothermals; Figure 19 gives two examples. If this simplest interpretation of the profiles is correct, then seeing corrections to the parameters are small enough to be practical (see also Fig. 21). However, in many galaxies an isothermal is a good fit only in an unphysically small radius range (i.e., r r_c). The approximate core radius may still be a useful parameter for these galaxies, but the deviations are physically interesting and need to be explained. These deviations are largest in NGC 4636 and in M87 (Young et al. 1978a) and progressively smaller in NGC 4589, 4406, 4621, 4697, 4382, 5846 and 7626. (The last three galaxies are not ranked; cf. King 1978 for a similar ranking. The "isothermal" galaxies are NGC 2300, 3379, 4261, 4365, 4374, 4472, 4552, 4649 and 6703.) If there are signs of these deviations in half of the galaxies studied, how compelling is an isothermal model? In particular (Schweizer 1979), how much extra light can be hidden in the nucleus of even an apparently isothermal galaxy? This question motivates a detailed examination of seeing effects, following Schweizer (1979, 1981a; see also de Vaucouleurs 1979, and references therein). The results are still somewhat ambiguous and model-dependent. However, it is clear that interesting amounts of light can be hidden in the nuclei of most galaxies, even many which appear isothermal.

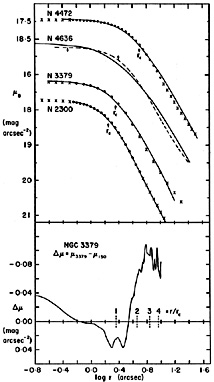Figure 19. Fits of seeing-convolved isothermal core models to profiles (solid lines) of four elliptical galaxies. The profiles of NGC 4472, 4636 and 2300 are from King (1978); that of NGC 3379 is from Kormendy (1977a, see de Vaucouleurs and Capaccioli 1979, Appendix I). Model points shown as filled circles were used in the least-squares fits; points not used are shown as crosses. The model profiles are isothermals (log rt / rc = ) except for NGC 2300, for which a King (1966) model with log(rt / rc) = 2.25 is used. Here rc = core radius; rt = limiting radius. Except for NGC 4636, model profiles are convolved with a Gaussian seeing profile with the observed *. The dashed line shown for NGC 4636 is an unconvolved isothermal; seeing corrections have the sense indicated by the arrows and would make the fit even worse. The parameters of the fits follow.
NGC 4472:	rc = 5.0";	rc, app = 5.5";	* = 0.85".
NGC 4636:	rc = 3.0";	rc, app = 3.2";	* = 0.58".
NGC 3379:	rc = 2.3";	rc, app = 2.9";	* = 0.88".
NGC 2300:	rc = 1.8";	rc, app = 2.1";	* = 0.67".
Here rc, app is the apparent core radius ignoring seeing and rc the true core radius of the fit illustrated. The lower panel shows the deviations of the fit in more detail for NGC 3379. At the center the galaxy is brighter than the model by 0.04 mag arcsec-2. Thus there is no significant evidence for peculiarities such as a central mass concentration or black hole, as suggested by de Vaucouleurs and Capaccioli (1979). A preferable interpretation seems to be that the center is well described by an isothermal, the profile from 17-21 B mag arcsec-2 by an r1/4 law with normal parameters, and the outer profile by the usual tidal distension for a T3 galaxy (Fig. 14).

The nearest, best-studied nucleus is that of M31. In Stratoscope II observations (Light, Danielson and Schwarzschild 1974) this is a clearly resolved feature rising above the core profile of the bulge (r_c 19", central surface brightness µ₀ ~ 17.0 B mag arcsec^-2). The diameter of the nucleus at half of central intensity is 1.0" × 1.6", the central surface brightness is 13.7 ± 0.3 B mag arcsec^-2. These values are corrected for an instrumental point-spread function with a dispersion _* 0.1". The total apparent B magnitude is 13.6 ± 0.3. Other parameters will be given below in Table 3. There are a number of reasons for believing that the nucleus and bulge of M31 are dynamically distinct entities (e.g., Schwarzschild 1980a; Tremaine and Ostriker 1982). (1) Schwarzschild (1980a) and Light et al. (1974) note that the brightness distribution is suggestive of separate components. For example, there is an isophote twist of ~ 20° between the nucleus and bulge, indicating that these features are differently triaxial. (2) The rotation curve of M31 has a sharp peak of amplitude V ~ 100 km s^-1 at r 2". At larger radii V decreases to ~ 20 km s^-1, before beginning to rise again in the bulge (Lallemand, Duchesne and Walker 1960; Morton and Thuan 1973; Walker 1974; Peterson 1978a; McElroy 1981). This suggests the presence of two mass components of very different characteristic radii. (3) There are indications of spectral differences between the bulge and the nucleus; these would imply population or metallicity differences (e.g., Cohen 1979; Faber and French 1980, but see Persson et al. 1980). (4) Whitmore (1980) suggests that the velocity dispersion _n of the nucleus may be larger than the central dispersion ₀ of the bulge: ₀ / _n = 0.83 ± 0.12. (5) Tremaine and Ostriker (1982) have shown that the dynamical effects of the bulge and the nucleus on each other are small. They derive dynamical models for the nucleus, and discuss constraints on its origin in some detail. Although the precise nature of the nucleus is not yet understood, it is unlikely to be the direct response of a stellar system to a nuclear "black hole". Tremaine and Ostriker conclude that the nucleus is a system of relatively ordinary stars on almost radial orbits.

Are there similar nuclei in elliptical galaxies? Schweizer (1979) argues that this is probable, basically by analogy with M31 and M32. This analogy is not very secure, because M31 and M32 are much less luminous than typical ellipticals studied to date (the absolute magnitude of the bulge of M31 is only M_B ~ -18.6, Kormendy and Illingworth 1982a, and M32 is much fainter still). Also, observed differences between bulges and bright ellipticals are suggestive of dynamical differences (sections 3.4.1; 4.2.6). However, Schweizer (1979) notes quite correctly that if M31 were placed at the typical distance of ellipticals studied to date, i.e., in the Virgo cluster, the nucleus would not be detected in ground-based measurements.

To determine how much nuclear light could be hidden in King's (1978) photometry, Schweizer adopts the computational convenience of r^1/4 laws. These model a particular amount of nuclear cusp in an arbitrary but plausible way, since they just extrapolate the overall profile to the center. (In fact, the nucleus and bulge of M31 have a combined profile which is approximately as bright at the center as the r^1/4 law of the bulge.) Schweizer shows that when r^1/4 laws are convolved with realistic point-spread functions, artificial cores are generated whose apparent radii r_{c, app} depend on the effective radii and the stellar seeing _*. As Figure 20 shows, only a few of King's galaxies have observed core radii which are much larger than these false core radii. That is, M87, NGC 4649 and NGC 4472 are well resolved, and NGC 4365 and 4636 are reasonably well resolved. Little extra light can be hidden in the nuclei of these galaxies. And, indeed, M87 did not successfully hide its core peculiarities from Young et al. (1978a), nor does NGC 4636 appear isothermal in Figure 19. On the other hand, most galaxies in Figure 20 fall close to the lines describing the false cores. This means that their cores are not resolved unambiguously. These galaxies could have isothermal cores, as illustrated in Figure 19. However they could instead be hiding nuclear brightness cusps as strong as those in r^1/4 laws. Furthermore, in at least one case where an isothermal is a poor fit, a convolved r^1/4 law gives an excellent fit to the central profile (NGC 4406, see Fig. 6 of Schweizer 1979). Galaxies such as NGC 4406 and 4636 are good candidates in which to pursue the search for nuclei.

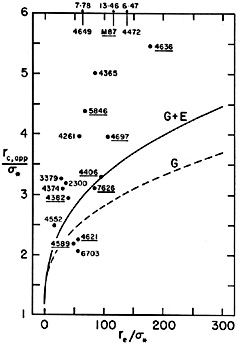Figure 20. Resolution of E-galaxy cores, here interpreted as the degree to which we can distinguish isothermal cores from artificial cores produced by seeing. The lines shown are for a convenient model profile which has a significant nuclear light cusp, i.e., an r1/4 law. Circularly symmetric r1/4 laws with effective radii re have been convolved with two point-spread functions, a pure Gaussian (G) and a Gaussian core plus exponential wings which begin at 2.25 * and have scale length 0.81" (G + E). The dispersion of the Gaussians is *. Seeing-convolved r1/4 laws have apparent cores whose radii rc, app are larger for larger effective radii, as shown by the lines. The points refer to galaxies measured by King (1978) and Kormendy (1977a), and are labeled with NGC numbers. Those that are underlined identify apparently non-isothermal cores. Galaxies which are not located well above the curves could be hiding nuclear brightness cusps comparable to those of the best-fitting r1/4 laws. This figure is adapted from Fig. 4 of Schweizer (1979).

Given the fact that galaxies near the G + E line in Figure 20 could be hiding nuclei, Schweizer assumes (by analogy with M31 and M32) that they are generally hiding brightness cusps which are strong enough to render the measured core parameters meaningless. I prefer the more conservative assumption that most cores are nearly isothermal, by analogy with the well-resolved cases NGC 4649, 4472 and 4365. Even M87 and NGC 4636 depart from isothermality by amounts which are small enough that useful approximate values of r_c can still be derived. If cores are isothermal then we can derive corrections for seeing as given in Figure 21. These corrections are manageably small if r_{c, app} 2.5_*. I must emphasize that it is an assumption that an isothermal and not an r^1/4 law is generally valid near the center. Distinguishing conclusively between these alternatives will require better resolution, perhaps even observations with Space Telescope.

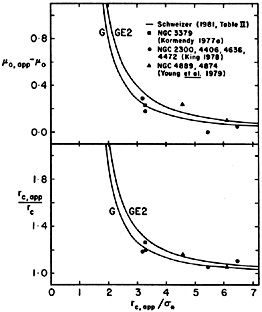Figure 21. Seeing corrections to convert apparent isothermal core radii rc, app and central surface brightnesses µ0, app to true values rc and µ0. The curves labeled G and GE2 are calculated by convolving isothermals with two point-spread functions, a Gaussian of dispersion * (G) and a Gaussian with exponential wings of scale length 0.5" beginning at 2 *. Values of rc, app and µ0, app are obtained by fitting unconvolved isothermals to the convolved ones. The resulting curves are taken from Schweizer (1981a). The points are for various galaxies whose observed profiles have been fitted with both unconvolved and convolved isothermals (e.g., Fig. 19). The agreement with the curves is good. The curve labeled GE2 provides the best simple estimate of seeing corrections for galaxies with isothermal cores.

Meanwhile, we can examine the internal physical consistency of the assumption that cores are generally isothermal. For example, Faber (1980) has shown that more luminous galaxies have larger cores. In fact, the well-resolved galaxies in Figure 20 are the most luminous ones studied; this is one reason for suspecting that fainter ellipticals just have smaller cores. However, consistency is not proof: the false core radii given by seeing-convolved r^1/4 laws also correlate with luminosity (Faber 1980). The reason is that r_e determines r_{c, app} (Fig. 20), and r_e depends on luminosity (equation 7). Another interesting correlation is that between central velocity dispersion and core radius (Fig. 22). This is a physically reasonable result which, when combined with the Faber-Jackson (1976) relation and the virial theorem, yields the plausible conclusion that the central mass-to-light ratio M₀/L₀ L^0.26 (see section 4.2.3). However, this relation could also, in principle, be spurious, through real relations between , L and r_e, and the seeing-induced dependence of r_{c, app} on r_e. Still, the r_c - L and r_c - correlations are both satisfied by galaxies which are well enough resolved so that seeing effects are small. This fact, and the physical consistency in the derived correlations provide some additional support for the assumption that most ellipticals have nearly isothermal cores. None of this changes the fact that many ellipticals may harbor nuclei in addition to cores, and that some cores are clearly not isothermal.

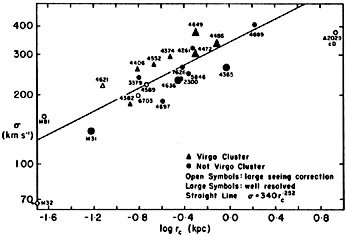Figure 22. Central velocity dispersion versus core radius for ellipticals and for the bulges of M31 and M81. The core radii have been corrected for seeing effects as in Figure 21. Seeing corrections are large enough to be unreliable in galaxies with open symbols (rc, app / * < 2.5); they are small enough to be relatively unimportant in galaxies with large symbols (rc, app / * > 5). There is a good correlation between and rc; a weighted least-squares fit with A2029 cD omitted gives the relation in the key. Distances are calculated using a Hubble constant of 50 km s-1 Mpc-1. Note that corrections for the Virgocentric flow field would decrease the scatter since the non-Virgo galaxies would be moved closer to us. Sources of photometry are as follows: M81, Illingworth (1981); M31, Johnson (1961), Kinman (1965), Light et al. (1974); NGC 3379, Kormendy (1977a); A2029 cD, Dressler (1979); other galaxies, King (1978).

To conclude the discussion of isothermal cores, I list in Table 3 some benchmark core parameters for the densest nucleus studied to date and for a typical elliptical. The total light L and mass M in the core region are much larger in the elliptical. However, the central density is much larger in the nucleus of M31, because it is both small and bright. This high central density results in a short relaxation time; Tremaine and Ostriker (1982) derive a value of 3 × 10⁹ yr, and discuss the possibility that a core collapse has occurred. In contrast, two-body relaxation is unlikely to be important in the cores of ellipticals. In particular, this does not seem to be a natural way to produce nuclear black holes.

Table 3. PHYSICAL PARAMETERS OF ISOTHERMAL CORES

Parameter	M31 Nucleus	NGC 2300
	(densest observed)	(typical elliptical)
rc	1.4 pc	370 pc
µ0	12.3 V mag arcsec-2	16.1 V mag arcsec-2
L0	4 × 105 L pc-2	1.4 × 104 L pc-2
	181 ± 12 km s-1	235 ± 14 km s-1
0	2.7 × 106 M pc-3	66 M pc-3
M0	7.8 × 106 M pc-2	4.9 × 104 M pc-2
M0 / L0	19	3.6
Lproj(rc)	1.7 × 106 L	4.2 × 109 L
Mproj(rc)	3.3 × 107 M	1.5 × 1010 M
NOTES. - The core radii rc and the central surface brightnesses µ0 and L0 are derived by fitting the function I(r) = I0(1 + r2/rc2)-1 to the photometry of Light et al. (1974) for M31 and of King (1978) for NGC 2300. These values are corrected for Galactic absorption, and for seeing (via Fig. 21). Both cores are only moderately well resolved: rc, app / * is 4.6 for the nucleus of M31 and 3.2 for NGC 2300. Adopted distances are 0.69 Mpc for M31 and 47 Mpc for NGC 2300. The central velocity dispersion of the M31 nucleus is taken from Whitmore (1980); that of NGC 2300 is from Tonry and Davis (1981a). The unprojected central density 0 of an isothermal is given by rc = 3(4 G 0)-1/2 (King 1966). The projected central density M0 is calculated from a tabulation by Peebles of the projected isothermal, for which M0 = 6.050(rc / 3), see Light et al. (1974). This yields also the central mass-to-light ratio M0 / L0. Finally, Lproj(rc) and Mproj(rc) are the total projected luminosity and mass within r = rc of the center of the image. The parameters rc, µ0, L0, and Lproj(rc) are at least operationally well defined by the observations; the other parameters depend critically on the assumption of an isothermal distribution. In particular, Tremaine and Ostriker (1982) point out that the derived masses can be much smaller if the radial velocity dispersion is much larger than the azimuthal dispersion. The present parameters agree reasonably well with those of Sandage (1971), Light et al. (1974) and Tremaine and Ostriker (1982, the high M/L model).

Significant dynamical evidence for a nuclear black hole was first found in M87 (Young et al. 1978a, Sargent et al. 1978, hereafter SYBSLH). This was an obvious galaxy for study because it is a strong radio source with an optical jet, and because its core is so well resolved that peculiarities should be hard to hide. Young et al. (1978a) have studied the core brightness profile; their excellent SIT plus CCD data are shown in Figure 23. There is a central point source, which appears to be partly stellar and partly non-thermal (Dressler 1980c). In addition, there is a core between ~ 2" and 10" radius, but one which maintains a significant brightness gradient even near the center. It is this brightness gradient (and not the nucleus) which suggests to Young and collaborators a model that contains a central point mass, possibly a black hole, of mass ~ 3 × 10⁹ M. A similarly non-isothermal core in the radio galaxy NGC 6251 can also be modeled by assuming the existence of a nuclear black hole (Young et al. 1979). These observations fuel the widespread suspicion that massive black holes might provide a natural engine to power nuclear activity.

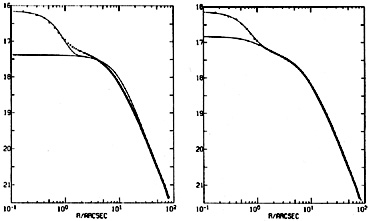Figure 23. Fits of various model profiles to the photometry (plus signs) of M87. These figures are taken from Young et al. (1978a); the photometry has been confirmed by de Vaucouleurs and Nieto (1979). The left-hand panel shows that a King model plus a nuclear point source do not fit the data. Some improvement would result from adopting a smaller fitting range, but the observed profile between 10" and 2" radius would still not turn down sharply enough into a core. The right-hand panel shows that it is possible to fit the data with a nuclear point source plus a King model modified to contain a central black hole of mass ~ 3 × 109 M.

Further support for the black hole model was provided by the observation of SYBSLH (see Figure 24) that velocity dispersions increase rapidly toward the center of M87. This increase was well fitted by a King model with mass-to-light ratio 6.5 and a nuclear point mass of ~ 5 × 10⁹ M. It was comforting to note that no such evidence for a central point mass was seen in the comparison galaxy NGC 3379, which has an isothermal core. However, more recent work has weakened the force of these conclusions. First, Illingworth (1981) has shown that the velocity dispersion gradient in M87 is in fact similar to that in the isothermal cores of NGC 3379 and NGC 4472 (Fig. 35). Also, Dressler (1980c) has remeasured the nuclear dispersion in M87 in well-defined and excellent seeing (0.75" diameter images in a 1" × 1" aperture, compared with 5.4" resolution in SYBSLH). He obtains a dispersion which is much smaller than the inward extrapolation of the SYBSLH model (see Fig. 24). A massive central black hole is still possible if the nuclear star cluster is large enough (i.e., barely unresolved). However, the model is no longer compelling. Finally, Duncan and Wheeler (1980) and Binney and Mamon (1982) note that the interpretation of the data is very model-dependent. If the assumption of an isotropic velocity distribution is relaxed, then it is possible to model the observations without using a black hole (see Binney 1982a for a review). Therefore, it is not yet observationally clear whether nuclear black holes exist.

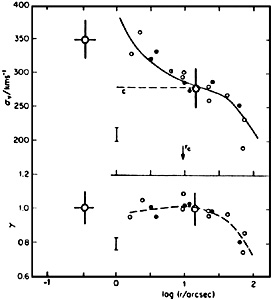Figure 24. Velocity dispersions V and line-strength parameter in M87, from Dressler (1980c). The small circles are from Sargent et al. (1978); open and closed, symbols are for measurements west and east of the nucleus, respectively. The core radius rc ~ 9.6" is marked. The large open circles are measurements by Dressler (1980c). The solid curve is the prediction of the black hole model of Sargent and collaborators, and the dashed curve shows the behavior of a King model without a central black hole.

Another galaxy whose central profile departs from an isothermal is NGC 1316 = Fornax A. In section 3.3.7 I discussed Schweizer's (1981b) conclusion that this galaxy is a merger remnant. Schweizer shows further that the brightness profile at r < 5" deviates above the inward extrapolation of an r^1/4 law fitted at larger radii. This extra light is well described by a King (1966) model with log (r_t / r_c) 2.65. Such a model has a very pronounced core-halo structure. Normal ellipticals have log (r_t / r_c) 2.25, a value which gives a profile like an r^1/4 law (Kormendy 1977c; King 1978). Corrected for seeing, the core radius in NGC 1316 is r_c 0.6" ± 0.2" or 0.10 ± 0.03 kpc (H₀ = 50 km s^-1 Mpc^-1). This is a surprisingly small value for a galaxy with M_V -23.8. For comparison, r_c 0.06 kpc in the bulge of M31 (M_{v, bulge} = -19.6). Typical ellipticals in King (1978) have 0.1 r_c 1.0 kpc (see Fig. 22) and -21.5 M_V - 23.5. Young et al. (1979) find still larger cores in ellipticals comparable in luminosity to NGC 1316: r_c ~ 2.1 kpc in NGC 4874 (M_V ~ -23.9);r_c ~ 1.7 kpc in NGC 4889 (M_V ~ -24.4), and r_c ~ 1.0 kpc in NGC 6251. Schweizer points out that a core as small as that in NGC 1316 would be unresolved in these distant ellipticals. However, as discussed above, many of the observed large cores appear to be real. Then NGC 1316 has an anomalously small core for its luminosity.

The properties of non-isothermal cores, and particularly the above observations, motivate the following question (see also section 4.2.5, and Hausman and Ostriker 1978; cf. Schweizer 1981b). Could a non-isothermal core be the signature of a merger of two galaxies which had very different core radii? That is, did part of the small core survive the merger to produce a "core within a core"? This process would be similar in principle to the possible building of galactic nuclei out of globular clusters through dynamical friction (Tremaine, Ostriker and Spitzer 1975; Tremaine and Ostriker 1982). It is particularly attractive for NGC 1316, which is suggested to be a merger remnant. The survival of small cores which are being eaten is not implausible in view of the high central surface brightnesses µ₀ of small ellipticals. For example, M32 has µ₀ ~ 14.7 B mag arcsec^-2 (Bendinelli et al. 1977), compared with an average <µ₀> = 16.9 B mag arcsec^-2 (dispersion = 0.7 mag arcsec^-2) for King's (1978) ellipticals. The core of M32 is also tightly bound; r_c ~ 8 pc (Walker 1962) compared with core radii of 0.1 - 1.0 kpc for large ellipticals. Finally, the parameters given in Table 3, and especially the high central density of the M31 nucleus, suggest that this nucleus, for example, might be robust enough to survive ingestion by NGC 2300. It would be worth looking further into the possibility that some cores might be non-isothermal because of mergers, especially in view of the insensitivity of overall profiles to merger processes.