Annu. Rev. Astron. Astrophys. 1988. 36: 539-598 Copyright © 1998 by Annual Reviews. All rights reserved

3.4. Observable Quantities

POWER    In the Blandford & Payne model, the ratio of jet and disk luminosities is

(10)

where r_{0 max} and r_{0 min} are the outer and inner disk radii and is an efficiency factor of transformation of binding energy at r_{0 min} into jet power. For the estimated parameters, this ratio is always << 1; i.e. only a small fraction of the accreted mass has to go into the wind. Only for relatively high-mass discharge onto the jet, 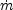_j ~ 0.1 M / year ~ 0.1 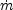_d, can the power in the jet reach L_j ~ 10⁴⁶ erg s^-1; this makes it difficult to explain the energetics of strong sources (see Section 2), especially when taking into account that only a small fraction can be transformed into radiation. Analytic results are confirmed substantially by the numerical simulations we discussed above. Hydromagnetic jets appear to be a very efficient way to extract energy from accretion disks, but perhaps a relativistic treatment is needed to fit the parameters well.

In this respect, unipolar inductor-type models are more promising. Camenzind (1998) showed that the Poynting flux of a rotating axisymmetric Kerr black hole magnetosphere can carry a magnetic luminosity:

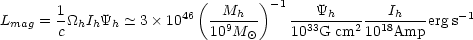(11)

where _h is the rotational velocity at the Kerr horizon and I_h the total current and _h the magnetic flux, respectively, integrated over the horizon.

While the understanding of the jet dynamics has progressed substantially, the problem of powering jets and enabling them to emit nonthermal radiation with luminosities up to ~ 10⁴⁷ erg s^-1 is still far from being solved.

ASYMPTOTIC BULK VELOCITY    In most jets, asymptotic bulk velocity is estimated indirectly through proper motions at VLBI resolution or through advancement velocities of extended structures or required Doppler beaming. This bulk velocity has to be supersonic and, in the inner portion of jets, relativistic, with Lorentz factors up to _bulk ~ 20. In general, the asymptotic velocity of outflows in hydromagnetic models is a few times the Keplerian velocity at the base of the flow, which corresponds to super-Alfvènic and relativistic velocities and allows Lorentz factors that are as large as needed, _bulk ~ 10-20. Kudoh & Shibata (1995) have investigated 1.5-D steady MHD winds from accretion disks, including thermal effects, and they obtained a relation between the jet mass flux and the magnetic energy of the disk. Their calculations confirm the above results for the terminal velocity with a weak dependence on the magnetic energy of the disk, v B^1/3.