


As a good first approximation, one should think of a map of the CMB anisotropy as a picture of the universe at a redshift of zdec = 1089, when the CMB decoupled from the primordial plasma. Thus, the CMB tells us about the universe when it was less than tdec = 379 kyrs old and a much simpler place. In this epoch, the early universe acts as though it is spatially flat, independent of the values of the dark energy and dark matter today.
The variation in temperature from spot to spot across the sky arises from the primordial plasma responding to spatial variations in the gravitational potential. In turn, the gravitational landscape is the manifestation of quantum fluctuations in some primordial field. In the inflationary model, one imagines these fluctuations stretched by at least 1028 so that they are super-horizon size, and then expanded with the expansion of the universe.
Observing the CMB is like looking at a distant surface (2) at the edge of the observable universe. As the universe expands, the pattern in the anisotropy will shift as new regions of the gravitational landscape are sampled. For example, one may imagine that the quadrupole (l = 2) may rotate 90° in one Hubble time (30 mas/century), with higher multipoles changing faster. In a similar vein, the light from the clusters of galaxies that formed in the potential wells that gave rise to cold regions on the decoupling surface has not has enough time to reach us.
The processes of the formation of stars, galaxies, and clusters of galaxies takes place between us and the decoupling surface. As a first approximation, photons from the decoupling surface come to us unimpeded. The lower redshift properties do, though, affect the light from the decoupling surface but in characteristic and definable ways as discussed below.
A full analysis of the CMB involves accurately comparing the measured power spectrum, Figure 2, to models. The simplest model that describes the CMB data is flat and lambda-dominated. The results for this parametrization derived from WMAP alone (Spergel et al. 2003) and the independent GUS analysis are shown in Table 1.
| Description | Parameter | WMAP | GUS | w/2dF |
| Baryon density |
 b
h2 b
h2 |
0.024 ± 0.001 | 0.023 ± 0.002 | 0.023 ± 0.001 |
| Matter density |
 m
h2 m
h2 |
0.14 ± 0.02 | 0.14 ± 0.01 | 0.134 ± 0.006 |
| Hubble parameter | h | 0.72 ± 0.05 | 0.71 ± 0.05 | 0.73 ± 0.03 |
| Amplitude | A | 0.9 ± 0.1 | 0.85 ± 0.06 | 0.8 ± 0.1 |
| Spectral index | ns | 0.99 ± 0.04 | 0.967 ± 0.029 | 0.97 ± 0.03 |
| Optical depth |  |
0.166+0.076-0.071 | ... | 0.148 ± 0.072 |
We can get at the essence of what the CMB is telling us from the
following. Let us focus on the decoupling surface. There is
a natural length scale
in the early universe that is smaller than the horizon size.
It corresponds to the distance over which a density perturbation
(sound wave) in the primordial plasma can propagate in the age of
the universe at the time of decoupling (tdec = 379
kyr). It is called
the acoustic horizon. Once we know the contents of the universe from
the overall characteristics of the power spectrum, we can compute
the size of the acoustic horizon. It is roughly
rs  cs tdec zdec
where cs is the sound speed in the plasma. In the full
expression
(Hu & Sugiyama 1995),
rs depends on only the physical densities
of matter and radiation and not on the Hubble parameter, h.
We may think of rs as a standard yard stick embedded
in the decoupling surface. From a map of the anisotropy, we measure the
angular size,
cs tdec zdec
where cs is the sound speed in the plasma. In the full
expression
(Hu & Sugiyama 1995),
rs depends on only the physical densities
of matter and radiation and not on the Hubble parameter, h.
We may think of rs as a standard yard stick embedded
in the decoupling surface. From a map of the anisotropy, we measure the
angular size,  A,
of the feature corresponding to rs. From WMAP,
A,
of the feature corresponding to rs. From WMAP,
 A =
0.598° ± 0.002. By definition then,
A =
0.598° ± 0.002. By definition then,
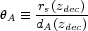 |
(1) |
where dA is the angular size distance to the
decoupling surface. In dA we can trade off the geometry,
 k = 1 -
k = 1 -
 r -
r -
 m -
m -

 ,
with h. Thus to determine the geometry without recourse to appealing
to the simplest model, we must make a prior assumption on h.
The dependence is not strong. If one assumes h > 0.5 then
one finds 0.98 <
,
with h. Thus to determine the geometry without recourse to appealing
to the simplest model, we must make a prior assumption on h.
The dependence is not strong. If one assumes h > 0.5 then
one finds 0.98 <
 tot <
1.08 (95% cl),
where again we have used the WMAP data
for illustration. The progress in our knowledge of
tot <
1.08 (95% cl),
where again we have used the WMAP data
for illustration. The progress in our knowledge of
 tot
as determined by all available data roughly between the past two IAU
symposia (starting with Figure 1,
Bond et al. 2003) is:
tot
as determined by all available data roughly between the past two IAU
symposia (starting with Figure 1,
Bond et al. 2003) is:
| January 2000 |
 tot =
1.06+0.16-0.10 tot =
1.06+0.16-0.10 |
| January 2002 |
 tot =
1.035+0.043-0.046 tot =
1.035+0.043-0.046 |
| January 2003 |
 tot =
1.034+0.040-0.042 tot =
1.034+0.040-0.042 |
| March 2003 (+WMAP) |
 tot =
1.015+0.063-0.015 tot =
1.015+0.063-0.015 |
One way to see what the CMB alone can tell us is to plot the data
in the  m -
m -

 plane for a pure cosmological
constant, or equation of state w = -1. This is shown in
Figure 4 for the WMAP data.
All simple open, flat, and closed
cosmological models satisfying the Friedmann equation can
be plotted here. One picks a point in the space, a single
source of the fluctuations (e.g., adiabatic fluctuations in the metric from
an inflationary epoch), w = -1, and marginalizes over the other
parameters (ns,
plane for a pure cosmological
constant, or equation of state w = -1. This is shown in
Figure 4 for the WMAP data.
All simple open, flat, and closed
cosmological models satisfying the Friedmann equation can
be plotted here. One picks a point in the space, a single
source of the fluctuations (e.g., adiabatic fluctuations in the metric from
an inflationary epoch), w = -1, and marginalizes over the other
parameters (ns,
 b,
b,
 , A) with uniform
priors. The possibilities are labeled by the Hubble parameter that goes
with them.
, A) with uniform
priors. The possibilities are labeled by the Hubble parameter that goes
with them.
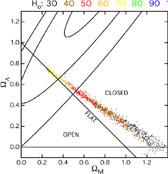 |
Figure 4. Models consistent with the
WMAP CMB data in the
|
There are a number of things the plot pulls together. First,
there is a large degeneracy in the CMB data along the line that
runs above the line for flat universe. This
is called the "geometric degeneracy" and is essentially the observation
noted above that one must pick h to determine
dA to complete the equation
 A =
rs / dA. The degeneracy line clearly
misses a model in which the universe is flat with
A =
rs / dA. The degeneracy line clearly
misses a model in which the universe is flat with
 m = 1
(
m = 1
(
 =
0), the Einstein-deSitter case. If one stretches the data slightly, it
is possible to have a model with
=
0), the Einstein-deSitter case. If one stretches the data slightly, it
is possible to have a model with
 m
m
 1.3
(
1.3
(
 =
0) but the
price one pays is a Hubble parameter near 0.3. This value is in conflict
with a host of other non-CMB observations. In addition, when one
considers the Integrated Sachs-Wolfe (ISW) induced cross-correlation
between cosmic structure, as measured by radio sources, and the CMB
anisotropy, this solution is disfavored at the
3
=
0) but the
price one pays is a Hubble parameter near 0.3. This value is in conflict
with a host of other non-CMB observations. In addition, when one
considers the Integrated Sachs-Wolfe (ISW) induced cross-correlation
between cosmic structure, as measured by radio sources, and the CMB
anisotropy, this solution is disfavored at the
3 level
(Nolta et al. 2003).
Thus, in this minimal picture, there are no models with
level
(Nolta et al. 2003).
Thus, in this minimal picture, there are no models with

 = 0
that fit the data.
= 0
that fit the data.
Once one moves off the x axis, the intersection of the
flat universe line,

 +
+
 m = 1,
and the geometric degeneracy is the next least baroque point, at least
by today's standards of baroqueness.
It is very satisfying that h for the intersection is very close
to the value obtained from the Hubble Key Project
(h = 0.72 ± 0.03(stat) ± 0.07(sys),
Freedman et al. 2001).
Additionally, the values agree with probes of the large scale structure
and the supernovae data. From the plot, it is easy to see why
such a weak prior on h (or
m = 1,
and the geometric degeneracy is the next least baroque point, at least
by today's standards of baroqueness.
It is very satisfying that h for the intersection is very close
to the value obtained from the Hubble Key Project
(h = 0.72 ± 0.03(stat) ± 0.07(sys),
Freedman et al. 2001).
Additionally, the values agree with probes of the large scale structure
and the supernovae data. From the plot, it is easy to see why
such a weak prior on h (or
 m) picks
out a flat universe. A number have noted that all determinations of
m) picks
out a flat universe. A number have noted that all determinations of
 tot are
greater than unity. The plot shows that with the priors we have chosen,
there are more solutions with
tot are
greater than unity. The plot shows that with the priors we have chosen,
there are more solutions with
 tot >
1. This may bias the solution somewhat.
tot >
1. This may bias the solution somewhat.
2 This is the "surface" at which
the CMB decoupled from the primordial electrons and baryons. It is
sometimes called the last scattering surface, but since
 15% of the CMB
photons were really last scattered when the universe was reionized near
z = 20, we prefer decoupling.
Back.
15% of the CMB
photons were really last scattered when the universe was reionized near
z = 20, we prefer decoupling.
Back.