


1.2. Dynamics
Everything discussed so far has been "geometrical", relying only on the form of the Robertson-Walker metric. To make further progress in understanding the evolution of the universe, it is necessary to determine the time dependence of the scale factor a(t). Although the scale factor is not an observable, the expansion rate, the Hubble parameter, H = H(t), is.
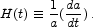 |
(8) |
The present value of the Hubble parameter, often referred to as the
Hubble "constant", is
H0  H(t0)
H(t0)
 100 h km
s-1Mpc-1 (throughout, unless explicitly stated
otherwise, the subscript "0" indicates the present time). The inverse of
the Hubble parameter provides an expansion timescale,
H0-1 = 9.78 h-1 Gyr. For
the HST Key Project
(Freedman et al. 2001)
value of H0 = 72 km s-1 Mpc-1
(h = 0.72), H0-1 = 13.6 Gyr.
100 h km
s-1Mpc-1 (throughout, unless explicitly stated
otherwise, the subscript "0" indicates the present time). The inverse of
the Hubble parameter provides an expansion timescale,
H0-1 = 9.78 h-1 Gyr. For
the HST Key Project
(Freedman et al. 2001)
value of H0 = 72 km s-1 Mpc-1
(h = 0.72), H0-1 = 13.6 Gyr.
The time-evolution of H describes the evolution of the universe. Employing the Robertson-Walker metric in the Einstein equations of General Relativity (relating matter/energy content to geometry) leads to the Friedmann equation
 |
(9) |
It is convenient to introduce a dimensionless density parameter,
 , defined by
, defined by
 |
(10) |
We may rearrange eq. 9 to highlight the relation between matter content and geometry
 |
(11) |
Although, in general, a, H, and
 are all
time-dependent, eq. 11 reveals that if ever
are all
time-dependent, eq. 11 reveals that if ever
 < 1, then it
will always be < 1 and in this case the universe is open
(
< 1, then it
will always be < 1 and in this case the universe is open
( < 0).
Similarly, if ever
< 0).
Similarly, if ever
 > 1, then it
will always be > 1 and in this case the universe is closed
(
> 1, then it
will always be > 1 and in this case the universe is closed
( > 0). For the special
case of
> 0). For the special
case of  = 1, where
the density is equal to the "critical density"
= 1, where
the density is equal to the "critical density"
 crit
crit
 3H2 / 8
3H2 / 8 G,
G,  is always
unity and the universe is flat (Euclidean 3-space sections;
is always
unity and the universe is flat (Euclidean 3-space sections;
 = 0).
= 0).
The Friedmann equation (eq. 9) relates the time-dependence of the scale factor to that of the density. The Einstein equations yield a second relation among these which may be thought of as the surrogate for energy conservation in an expanding universe.
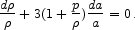 |
(12) |
For "matter" (non-relativistic matter; often called "dust"),
p <<
 , so that
, so that
 /
/
 0
= (a0 / a)3. In contrast,
for "radiation" (relativistic particles)
p =
0
= (a0 / a)3. In contrast,
for "radiation" (relativistic particles)
p =  /
3, so that
/
3, so that  /
/
 0 =
(a0 / a)4.
Another interesting case is that of the energy density and pressure
associated with the vacuum (the quantum mechanical vacuum is not
empty!). In this case p =
-
0 =
(a0 / a)4.
Another interesting case is that of the energy density and pressure
associated with the vacuum (the quantum mechanical vacuum is not
empty!). In this case p =
- , so that
, so that
 =
=
 0.
This provides a term in the Friedmann equation entirely equivalent
to Einstein's "cosmological constant"
0.
This provides a term in the Friedmann equation entirely equivalent
to Einstein's "cosmological constant"
 . More generally,
for p = w
. More generally,
for p = w
 ,
,
 /
/
 0 =
(a0 / a)3(1+w).
0 =
(a0 / a)3(1+w).
Allowing for these three contributions to the total energy density, eq. 9 may be rewritten in a convenient dimensionless form
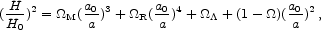 |
(13) |
where 

 M +
M +
 R +
R +

 .
.
Since our universe is expanding, for the early universe (t
<< t0)
a << a0, so that it is the "radiation"
term in eq. 13 which dominates; the early universe is
radiation-dominated (RD). In this case
a  t1/2 and
t1/2 and

 t-2, so that the age of the universe or,
equivalently, its expansion rate is fixed by the radiation
density. For thermal radiation, the energy density is only
a function of the temperature
(
t-2, so that the age of the universe or,
equivalently, its expansion rate is fixed by the radiation
density. For thermal radiation, the energy density is only
a function of the temperature
( R
R
 T4).
T4).
1.2.1. Counting Relativistic Degrees of Freedom
It is convenient to write the total (radiation) energy density in terms of that in the CMB photons
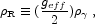 |
(14) |
where geff counts the "effective" relativistic degrees of freedom. Once geff is known or specified, the time - temperature relation is determined. If the temperature is measured in energy units (kT), then
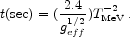 |
(15) |
If more relativistic particles are present, geff increases and the universe would expand faster so that, at fixed T, the universe would be younger. Since the synthesis of the elements in the expanding universe involves a competition between reaction rates and the universal expansion rate, geff will play a key role in determining the BBN-predicted primordial abundances.
Photons are vector bosons. Since they are massless, they have
only two degress of freedom: geff = 2. At temperature
T their number density is
n = 411(T / 2.726K)3
cm-3 = 1031.5 T3MeV
cm-3, while their contribution to the total radiation energy
density is
= 411(T / 2.726K)3
cm-3 = 1031.5 T3MeV
cm-3, while their contribution to the total radiation energy
density is

 =
0.261(T / 2.726K)4 eV cm-3. Taking
the ratio of the energy density to the number density leads to the
average energy per photon
<E
=
0.261(T / 2.726K)4 eV cm-3. Taking
the ratio of the energy density to the number density leads to the
average energy per photon
<E > =
> =

 /
n
/
n = 2.70 kT. All other relativistic
bosons may be simply related to photons by
= 2.70 kT. All other relativistic
bosons may be simply related to photons by
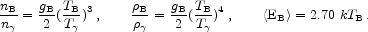 |
(16) |
The gB are the boson degrees of freedom (1 for a scalar,
2 for a vector, etc.). In general, some bosons may have decoupled
from the radiation background and, therefore, they will not
necessarily have the same temperature as do the photons
(TB  T
T ).
).
Accounting for the difference between the Fermi-Dirac and Bose-Einstein distributions, relativistic fermions may also be related to photons
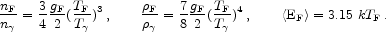 |
(17) |
gF counts the fermion degrees of freedom. For example, for electrons (spin up, spin down, electron, positron) gF = 4, while for neutrinos (lefthanded neutrino, righthanded antineutrino) gF = 2.
Accounting for all of the particles present at a given epoch in the early (RD) evolution of the universe,
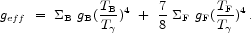 |
(18) |
For example, for the standard model particles at temperatures
T
 few MeV there are photons, electron-positron
pairs, and three "flavors" of lefthanded neutrinos (along with
their righthanded antiparticles). At this stage all these particles
are in equilibrium so that
T
few MeV there are photons, electron-positron
pairs, and three "flavors" of lefthanded neutrinos (along with
their righthanded antiparticles). At this stage all these particles
are in equilibrium so that
T = Te =
T
= Te =
T where
where


 e,
e,
 µ,
µ,

 . As a result
. As a result
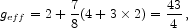 |
(19) |
leading to a time - temperature relation: t = 0.74 T-2Mev sec.
As the universe expands and cools below the electron rest mass energy,
the e± pairs annihilate, heating the CMB photons,
but not the
neutrinos which have already decoupled. The decoupled neutrinos
continue to cool by the expansion of the universe
(T
 a-1), as do the photons which now have a higher
temperature
T
a-1), as do the photons which now have a higher
temperature
T = (11/4)1/3
T
= (11/4)1/3
T (n
(n /
n
/
n = 11/3).
During these epochs
= 11/3).
During these epochs
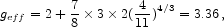 |
(20) |
leading to a modified time - temperature relation: t = 1.3 T-2Mev sec.
1.2.2. "Extra" Relativistic Energy
Suppose there is some new physics beyond the standard model of
particle physics which leads to "extra" relativistic energy so that
 R
R

 'R
'R

 R +
R +
 X;
hereafter, for convenience of notation, the subscript
R will be dropped. It is useful, and conventional, to account
for this extra energy in terms of the equivalent number of extra
neutrinos:
X;
hereafter, for convenience of notation, the subscript
R will be dropped. It is useful, and conventional, to account
for this extra energy in terms of the equivalent number of extra
neutrinos:  N
N

 X /
X /

 (Steigman, Schramm,
& Gunn 1977
(SSG); see also
Hoyle & Tayler 1964,
Peebles 1966,
Shvartsman 1969).
In the presence of this extra energy,
prior to e± annihilation
(Steigman, Schramm,
& Gunn 1977
(SSG); see also
Hoyle & Tayler 1964,
Peebles 1966,
Shvartsman 1969).
In the presence of this extra energy,
prior to e± annihilation
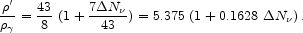 |
(21) |
In this case the early universe would expand faster than in the standard model. The pre-e± annihilation speedup in the expansion rate is
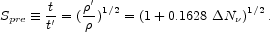 |
(22) |
After e± annihilation there are similar, but quantitatively different changes
 |
(23) |
Armed with an understanding of the evolution of the early universe and its particle content, we may now proceed to the main subject of these lectures, primordial nucleosynthesis.