2. PARTICLE AND RADIATION SPETRA IN STARBURST GALAXIES

Acceleration in SN shocks by the first-order Fermi process yields a power-law distribution with index q 2 (e.g., Protheroe & Clay 2004) in a very wide energy range, from a value close to the mean thermal energy of the gas particles (in non-relativistic shocks) to a very high value ( 10¹⁴ eV). The accelerated proton-to-electron (p/e) density ratio, N_p / N_e, in the source (either the SB or the full disk) region can be calculated assuming charge neutrality (Bell 1978, Schlickeiser 2002). This ratio reaches its maximum value, (m_p / m_e)^(q-1)/2 (for q > 1; m_e and m_p are the electron and proton masses), over most of the relevant range of particle energies, E > 1 GeV. (For the dependence of this ratio on particle energy, and more discussion on this and other relevant physical processes, see PRA and references therein.)

The electron density in the source region, N_e, is inferred from radio measurements (of the same region); by adopting the theoretically expected expression N_p / N_e = (m_p / m_e)^(q-1)/2, the proton density N_p can be deduced. The fit to the radio data provides both the normalization of the electron spectrum and the actual value of q, which is found to be somewhat larger than 2, even in the central SB region. In this procedure the electron population is composed of both primary and secondary electrons, with the latter self-consistently determined by accounting for the pion yield of energetic protons with protons in the gas. We note that the theoretically predicted value of the density ratio is valid in the source region, where energy equipartition is more likely to be attained since the relevant processes couple particles and fields more effectively than in the rest of galactic disk.

The particle spectral distributions evolve differently as they propagate out from their acceleration region. Typically, the electron spectrum is most directly deduced from measurements of synchrotron radio emission. The inferred spectrum can be related to the source spectrum through a solution of the kinetic equation describing the propagation modes and energy losses by electrons and protons as they move out from their acceleration region. A very useful detailed description of the time-dependent spectro-spatial distribtion of protons, diffusing out of a region with a discrete population of acceleration sites, was recently given by Torres et al. (2012). This study elucidates the explicit dependence of the distribution on distance from the acceleration site, energy loss time, and the diffusion coefficient. It also follows the temporal evolution of the distribution towards a steady state.

Since the estimated duration of a SB phase is ~ 10⁸ yr, a timescale which is much longer than any of the relevant energy loss or propagation timescales for electrons and protons, in (essentially) all treatments a steady state is assumed to be attained. Since the calculation of particle steady-state spectra requires inclusion of all the important energy loss mechanisms and modes of propagation, the treatment is necessarily numerical. We have employed the code of Arieli & Rephaeli (2007), which is based on a modified version of the GALPROP code (Moskalenko & Strong 1998, Moskalenko et al. 2003), to solve the kinetic equation for N_i(, R, z), where i = e, p, is the Lorentz factor, and R and z are the 2D spatial radius and the coordinate perpendicular to the galactic plane. The exact Fokker-Planck diffusion-convection equation (e.g., Lerche & Schlickeiser 1982) was solved in 3D with given source distribution and boundary conditions for electrons and protons. In addition to diffusion with an energy dependent coefficient, particles are assumed to be convected by a galactic wind with spatially varying velocity.

The dominant energy losses of high-energy electrons are synchrotron emission and Compton scattering by the FIR and optical radiation fields; these processes (obviously) depend on the mean strength of the magnetic field, B, and the energy density of the radiation fields, respectively. At energies below few hundred MeV, electrons lose energy mostly by Coulomb interactions with gas particles. At low energies proton losses are dominated by Coulomb interactions with gas particles. Protons with kinetic energy above the (range of) pion masses (~ 140 MeV) lose energy mainly through interactions with ambient protons, yielding neutral (⁰) and charged (^±) pions. Neutral pions decay into photons, while decays of ^± result in energetic e^± and neutrinos. The proton and (total) electron components are coupled through the production of secondary electrons in ^- decay (following their creation in pp interactions).

Measured synchrotron radio spectra provide the critically important information on the particle spectra and their overall normalization: Fitting the predicted radio emission to measurements fixes normalization of the steady state electron and - based on a theoretical prediction - proton energy distributions. From these measurements alone the electron density and mean magnetic field cannot be separately determined. To do so it is usually assumed that particle and magnetic field energy densities are equipartitioned. In our numerical treatment this approach necessitates an iterative procedure to solve for N_e, N_p, and the field strength at the center, B₀, given a measured value of the radio flux.

Particles diffuse and are convected out of their source region. Diffusion is likely to be random walk against magnetic field inhomogeneities, with an estimated value of ~ 3 × 10²⁸ cm²/s for the central diffusion coefficient. Convection is by galacitc wind with a typical velocity of ~ 500 km s^-1 (Strickland et al. 1997) in the source region. Based on Galactic cosmic-ray MHD wind models, we assume that the convection velocity increases linearly with distance from the disk plane (e.g. Zirakashvili et al. 1996).

The other quantities needed to calculate the steady state distributions of electrons and protons are the densities of neutral and ionized gas in the central SB region and throughout the disk, the central value of the (mean) magnetic field and its spatial profile across the disk, and energy densities of ambient radiation fields (including the CMB). As discussed in PRA, it is assumed that magnetic flux is conserved in the IS ionized gas, so that the mean strength of the field can be related to the local ionized gas density, n_e, using the scaling B n_e^2/3 (Rephaeli 1988). If instead energy equipartition is assumed, and the magnetic energy density is scaled to the thermal gas energy density, then the proportionality relation is B n_e^1/2. In our work we have taken the ionized gas density profile to be n_e exp(-z / z₀) / (1 + (R / R₀)²), typically with R₀ = 1.5 kpc, and z₀ = 0.5 kpc (as deduced for NGC 253 by Strickland et al. 2002).

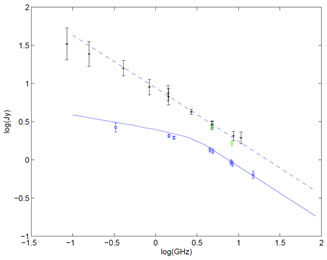Figure 1. Spectral fits to radio measurements of the SB and entire disk regions of NGC 253 (RAP). The solid line is a fit to the emission from the SB region; the dashed line is a fit to the emission from the entire disk. Data are from Klein et al. (1983, black dots), Carilli (1996, blue squares), and Heesen et al. (2008, green circles).

Given the measured radio fluxes from the central and full disk regions of the two nearby SBGs M 82 and NGC 253, shown in Figure 1 for the latter galaxy, and values of all the above quantities, the steady state particle spectra and their radiative yields were calculated using the modified GALPROP code. Here we present the results of this work; more details on the method and values of the input parameters can be found in PRA and RAP.

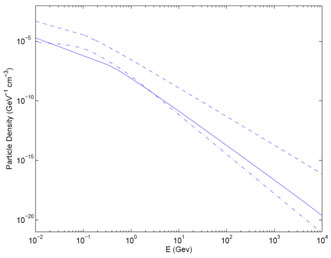Figure 2. Primary proton (dashed line), primary electron (solid line), and secondary electron (dashed-dotted line) spectral steady state density distributions in the central SB region NGC 253 (RAP).

The high-energy photon spectra of NGC 253 are shown in Figure 3 (from RAP). Emission levels depend mostly on values of the proton to electron ratio in the source region, on the magnetic field, gas density, and their spatial profiles. The basic normalization of the electron density is provided by the measured radio emission in the source region; the variation of the electron spectrum across the disk is largely determined by synchrotron losses. Uncertainty in the estimated level of emission is largely due to the steep dependence of the electron density on the field. As argued by RAP, the central value of the (mean) magnetic field is unlikely to be appreciably higher than the value deduced in N 253 (and also in M 82), B₀ ~ 200 µG. A lower field value would result in a reduced proton density and a lower rate of ⁰ decays. For a given radio flux the electron density would have to be correspondingly higher, resulting in higher bremsstrahlung and Compton yields, roughly compensating for the lower level of hadronic emission. Emission from ⁰ decay depends linearly on the ambient proton density in the central disk region.

Figure 3. High-energy emission from the disk of NGC 253. Radiative yields are from Compton scattering off the FIR radiation field (dotted line), electron bremsstrahlung off ambient protons (dashed line), 0 decay (dashed-dotted line), and their sum (solid line).

Detailed modeling of high-energy emission from M82 by PRA preceded its detection by VERITAS (Acciari et al. 2009) and Fermi (Abdo et al. 2010a). These observations are shown in Figure 4 (from Abdo et al. 2010a), together with theoretical predictions including that of PRA. The detected flux level agrees well with that predicted by PRA and by de Cea et al. 2009. Results from our similar treatment of the steady-state particle and radiation spectra of NGC 253 were presented in RAP. The estimated high energy fluxes for this galaxy (RPA, Paglione et al. 1996, Domingo-Santamaria & Torres 2005) were also in the range measured by Fermi (Abdo et al. 2010a), and - in the TeV region - by the HESS telescope (Acero et al. 2009), given the substantial observational and modeling uncertainties. These observational results and predicted spectra are shown in Figure 5 (adopted from Abdo et al. 2010a); our integrated spectrum and estimated 1 uncertainty region are marked by the dashed blue lines (inserted into the original figure). These two SBGs are the only extragalactic non-AGN sources that were detected at both GeV and TeV energies. The FIR luminosities of these galaxies are at or somewhat below the nominal level for SBGs; clearly, their apparent high brightness is due to their proximity.

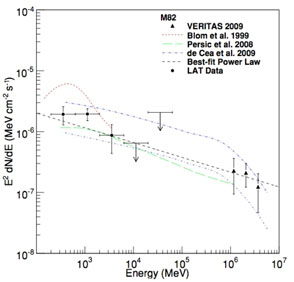Figure 4. High-energy emission from M82. VERITAS (Acciari et al. 2009) and Fermi measurements are shown together with predicted spectra. Figure is reproduced from Abdo et al. (2010a).

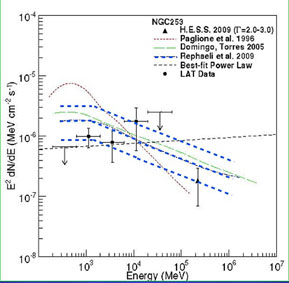Figure 5. High-energy emission from the disk of NGC 253. Fermi and H.E.S.S measurements are shown together with predicted spectra. The figure is reproduced from Abdo et al. (2010b); the added dashed blue lines show our predicted spectrum with the estimated range of 1 uncertainty.

A few other local and nearby galaxies were also detected at high energies: LMC (Abdo et al. 2010c), SMC (Abdo et al. 2010d), Andromeda (M 31; Abdo et al. 2010b), and the composite Sy2/SB galaxies NGC 1068 and NGC 4945 (Lenain et al. 2010). The emission in these galaxies is mostly hadronic -ray emission, except for NGC 1068 where emission from the active nucleus may be dominant. Among galaxies whose detected high energy emission is powered by stellar activity, Arp 220 has the highest SFR in the local universe, yet an attempt to detect it by MAGIC (Albert et al. 2007) was not successful due to its relatively large distance.