


In Sec. 10 we have seen that the WMAP 7-year CMB data together with the Hubble constant value testify about the existence of DM [49, 51]. In Sec. 11 we addressed the BAO data [53] with the same conclusion. In overall fits one combines these with supernova data (SN Ia) which offer a constraint nearly orthogonal to that of CMB in the ΩΛ - Ωm-plane.
The Union compilation of 307 selected SN Ia includes the recent large samples of SNe Ia from the Supernova Legacy Survey, the ESSENCE Survey, the older data sets, as well as the recently extended data set of distant supernovae observed with HST. M. Kowalski & al. [55] present the latest results from this compilation and discuss the cosmological constraints and its combination with CMB and BAO measurements. The CMB constraint is close to the line ΩΛ + Ωm = 1, whereas the supernova constraint is close to the line ΩΛ - 1.6 × Ωm = 0.2. The BAO data constrain Ωm, but hardly at all ΩΛ. This is shown in Fig. 17.
 |
Figure 17. 68.3 %, 95.4 % and 99.7% confidence level contours on ΩΛ and Ωm obtained from CMB, BAO and the Union SN set, as well as their combination (assuming w = -1). Note the straight line corresponding to a flat Universe with ΩΛ + Ωm = 1. From M. Kowalski & al. [55]. |
Defining the vacuum energy density parameter by Ωk = 1 - ΩΛ - Ωm, a flat Universe corresponds to Ωk = 0. For a non-flat Λ CDM Universe with a cosmological constant responsible for dark energy, a simultaneous fit to the data sets gives
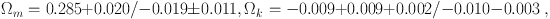 |
(14) |
where the first error is statistical and the second error systematic. Clearly one notes that the Universe is consistent with being flat. Subtracting Ωb = 0.045 from Ωm = 0.285 one obtains the density parameter for DM, Ωdm≈ 0.24. Assuming flatness, M. Kowalski & al. [55] find Ωm=0.274± 0.016± 0.013. This compares well with the combined 7-year WMAP data and the ACT data, Ωm = 0.276 ± 0.016 [51]. If one fits different models having more free parameters, one gets slightly different results, but all within these 1σ errors.