Annu. Rev. Astron. Astrophys. 2013. 51:63-104 Copyright © 2013 by Annual Reviews. All rights reserved

1. INTRODUCTION

Given the dominant role of radiation in astrophysics, its transport through a medium is one of the most fundamental processes to be considered. Analyzing the radiation an object sends provides us with information about not only its radiation source but also the medium in between and surrounding the object and the observer.

Dust grains play a special role in producing and processing radiation. They are efficient at absorbing and scattering UV through near-infrared (NIR) photons and then reradiating the absorbed energy in the infrared and submillimeter (submm) wavelength range. Cosmic dust can be found in many astrophysical objects such as the Solar System (Hoppe et al. 2010), comets and meteoroids (Küppers et al. 2005), substellar atmospheres (Harvey et al. 2012), young stellar objects (Keller et al. 2008), protostellar to protoplanetary disks (Watson et al. 2009), evolved stars (Groenewegen et al. 2011), reflection nebulae (Castellanos et al. 2011), supernova remnants (Rho et al. 2009), molecular clouds (Martel, Urban & Evans 2012), the interstellar medium (ISM) (Zhukovska, Gail & Trieloff 2008), galaxies (Dunne et al. 2011), AGN (Haas et al. 2000), and the high-redshift universe (Dwek, Galliano & Jones 2007). For an unbiased analysis of these objects intrinsic quantities, it should be taken into consideration that dust grains modify the radiation fields in these objects. Such an analysis requires performing radiative transfer (RT) calculations.

Aside from its importance as a tracer, the physical and chemical processes related to dust itself are of interest. They cover its formation; its cycle in galaxies; the variation in opacity with chemical composition; its growth and destruction processes in cloud cores and circumstellar disks, which allow it to act as a building block for planets; its interaction with magnetic fields; and its surface chemistry. For example, the dust RT is important for understanding chemistry in the ISM, as photodissociation rates are strongly dependent on the UV radiation field that includes a significant amount of photons scattered from dust grains. This review discusses the physical properties of dust only in the context of RT and the modeling of objects; for other aspects mentioned above, we refer the reader to any one of the many published works on dust (e.g. (Draine 2003a, Henning, Grün & Steinacker 2009, Henning 2010).

Many dusty objects have been observed at increasingly higher spatial resolution in the past 10 years. These observations cover UV/optical/NIR [e.g., the Hubble Space Telescope (HST), the Galaxy Evolution Explorer (GALEX), and ground-based telescopes] and infrared/submillimeter [e.g., the Spitzer Space Telescope (Spitzer), Akari, Herschel, Planck, the Wide-Field Infrared Survey Explorer (WISE), the Atacama Large Millimeter Array (ALMA), the James Clerk Maxwell Telescope (JCMT), the Atacama Pathfinder Experiment (APEX), and the Institut de Radio Astronomie Millimétrique (IRAM) 3-m Telescope] wavelengths. Space telescopes exploring atmospherically absorbed wavelength windows, high-resolution interferometric data, polarization data, and all-sky maps are just a few examples of the rich data set that awaits the RT modeler. Comparison of dust RT calculations with global and pixel-by-pixel resolved spectral energy distributions (SEDs) of dusty objects provides information on the properties of the illuminating sources (stars, accretion disks, integrated star formation rate, etc.), the distribution of the dust (disk structures, cloud geometries, underlying multiphase nature, etc.), and properties of the dust grains (size, shape, and composition). A feature commonly seen in high spatial resolution images at all wavelengths is the complex nature of the dust density distribution. Examples of global 3D geometries include complex arm structures in spiral galaxies (Patrikeev et al. 2006, Fritz et al. 2012)), large scale filaments in star-forming regions (André et al. 2010, Arzoumanian et al. 2011), and bow-shocked shells around evolved stars (Cox et al. 2012). A prominent example of locally complex 3D geometries is the known fractal nature of the ISM (Beech 1987, Falgarone, Phillips & Walker 1991). Images illustrating complex local and global 3D dust structures are shown in Figure 1. In addition, the illuminating sources of dust have been long known to have complex distributions from the combination of the anisotropic interstellar radiation field and local neighboring stars to the stellar distribution in galaxies. Both of these issues show that a complete 3D treatment of RT is inevitable and critical for progress in many fields.

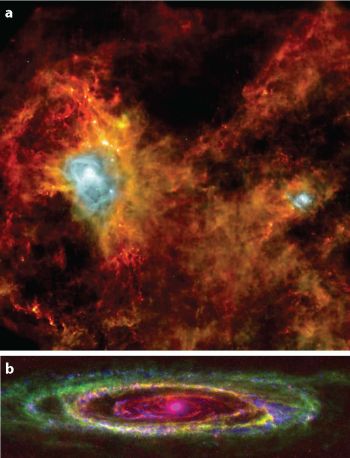Figure 1. The complex and filamentary structures of ISM dust are seen clearly in both Milky Way star-formation regions (a) and external galaxies (b). Panel a is a color image of the Aquila star-formation complex (André et al. 2010). This image was taken as part of the Gould Belt Survey Herschel Key Project; covers ~ 11 deg2; and was created from PACS 70-µm (blue), PACS 160-µm (green), and SPIRE 250-µm (red) observations. Panel b shows the complex structure of the ISM in M31 from Hi observations (green; see also Braun et al. 2009), embedded star formation using Spitzer 24-µm data (red; see also Gordon et al. 2006), and unobscured young stars from the GALEX far-UV images (blue; see also Thilker et al. 2005). Abbreviations: GALEX, the Galaxy Evolution Explorer; ISM, interstellar medium; PACS, Photodetector Array Camera & Spectrometer; SPIRE, Spectral and Photometric Imaging Receiver; Spitzer, the Spitzer Space Telescope.

Among the many computational problems in astrophysics, 3D line and dust RT has long been a major challenge and often approximated or neglected. Although, for example, 3D magnetohydrodynamics (MHD) codes have existed for many years, radiation transport is considered to be one of the four grand challenges in computational astrophysics. [See, for example, the Grand Challenge Problems in Computational Astrophysics conference series, 2005–2007, at the Institute for Pure and Applied Mathematics at the University of California, Los Angeles (https://www.ipam.ucla.edu/)]. Dust RT is different than line RT in that the dust opacities generally do not depend on the RT solution itself.

The reasons to neglect or approximate 3D dust RT are manifold. A good portion of the difficulties arises because the underlying physical processes combine, in the stationary case, to a nonlocal and nonlinear 6D problem. Because the radiation field needs to be determined in all directions, at any spatial location, and for each wavelength, the solution vector itself comprises three dimensions more than the variables in MHD problems. The RT problem is nonlocal in space (propagation of the photons within the entire domain), direction (scattering and absorption/re-emission), and wavelength (absorption/re-emission). This nonlocality makes it difficult to simplify the problem by neglecting processes or wavelengths. For example, absorption and scattering have roughly the same efficiency from UV to NIR, with strongly anisotropic scattering (Gordon 2004). In modeling far-infrared images, a consistent treatment of the dust emission requires the RT to be calculated where the dust absorption happens, at shorter wavelengths. Therefore, most of the current 3D dust applications are intrinsically multiwavelength in nature.

Other difficulties are related to the complexity of 3D structures. The underlying grids to resolve the sink and source contributions to the radiation fields are generally discretized and require substantial storage, and the RT calculation effort rises with the decreasing cell size. Moreover, when modeling the structure, the spatial distribution of the sources and sinks has to be parametrized. Modeling complex structures with a simple spatial distribution model can lead to misleading results. Witt & Gordon (1996) showed that RT through a 3D fractal dust distribution was significantly different than through similar, yet smooth, distributions. In addition to the longer runtimes expected for the 3D dust RT code, an exploration or optimization of the parameter space challenges the capabilities of current computers. Finally, more than for simple geometries, applying 3D dust RT is challenging given the loss of information due to projection effects.

As a result of nonlocal and nonlinear effects, the radiative transfer equation (RTE) is an integro-differential equation including a scattering integral; the thermal source term is nonlinearly coupled to a double-integral equation, making it difficult to apply common solvers. Moreover, the spatially varying extinction causes changes in the numerical nature of the RTE. Its character changes from parabolic for the diffusive transport to hyperbolic for freely streaming photons, to a combination of the two in the numerically difficult transition region. Solving such a high-dimensional nonlocal, nonlinear problem requires substantial computational resources (both computing power and memory), affecting the solution algorithms and potentially limiting the model complexity.

We review the significant progress made in this evolving and dynamic field to tackle this grand challenge problem. Within the past 10 years, the availability of high resolution images and increase in computer speed and storage have triggered an expansion of the dust RT community and the development of new codes capable of dealing with the complete 3D dust RT problem. Many of the techniques used to solve the 3D dust RT problem were developed originally for 1D or 2D geometries. The added computational complexity of solving the 3D problem has emphasized the need for highly efficient techniques, leading to refinements in and use of all possible 1D/2D methods in most 3D codes. Applications explicitly using 3D RT include models of young stellar objects (Wolf, Fischer & Pfau 1998), protostellar to protoplanetary disks (Indebetouw et al. 2006, Niccolini & Alcolea 2006), reflection nebulae (Witt & Gordon 1996), molecular clouds (Steinacker et al. 2005, Pelkonen, Juvela & Padoan 2009), spiral galaxies (Bianchi 2008, Schechtman-Rook, Bershady & Wood 2012), interacting and starburst galaxies (Chakrabarti et al. 2007, Hayward et al. 2011), and AGN (Schartmann et al. 2008, Stalevski et al. 2012). This expansion motivated the Cosmic Dust and Radiative Transfer workshop held in Heidelberg in 2008 (http://www.mpia.de/RT08/). During the workshop, we realized that a review of the various techniques and applications addressing 3D dust RT was needed to communicate common strategies between related fields. It would also be useful for coders and users of dust RT codes and people wishing to enter the field (including writers of line RT and MHD codes).

Although various solver techniques are used for RT problems, 3D dust RT is commonly solved using the Monte Carlo (MC) technique, with some applications using the ray-tracing (RayT) technique. Because modern MC solvers make use of some RayT methods, this review focuses on the spectrum of techniques based on these two approaches.

Other RT solution methods exist but have either not been used to solve the complete 3D dust RT problem, or have shown clear disadvantages. One potential solution method is discretizing the RTE, for example, with a finite-difference approach in spatial Cartesian coordinates and in direction space, to create a system of linear equations (Stenholm, Stoerzer & Wehrse 1991, Steinacker, Bacmann & Henning 2002). The corresponding matrix is extremely difficult to solve even with powerful matrix solvers (van der Vorst 1992). Two more methods are used for RT problems, but have yet to be applied to 3D dust RT. The discretization can be performed on unstructured grids (e.g., a Delaunay grid), and this can provide a very fast solver. Currently, such algorithms have been updated to handle freely streaming photon packages and changes in the optical depth, but treatment of scattering has yet to be explored. Finally, the moment method expands the intensity as a function of angle using spherical harmonics as basis functions. It has several numerical advantages both in terms of solution accuracy and storage requirements, but can exhibit nonphysical oscillations. A common variant of the moment method is the variable Eddington tensor method (used, e.g., for 2D in the code RADICAL; see description in Pascucci et al. 2004).

This review starts with the mathematical definition of the full 3D dust RT problem. Next, we present the discretization of the problem in spatial, direction, and wavelength dimensions. The RayT and MC methods of solving the 3D RT problem are described in detail. Challenges in comparing RT models with observations are discussed. A listing of existing 3D dust RT codes is given along with current benchmarking efforts. Finally, the review is concluded with a summary and discussion of the future of 3D RT.