5.3. Physical scales in the evolution of magnetic fields

When MHD is discussed in curved spce-times, the evolution of the geometry reflects in the time evolution of the physical scales of the problem.

5.3.1. Before matter-radiation equality

From Eq. (5.32), the comoving momentum corresponding to the magnetic diffusivity scale is given by the relation

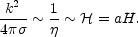(5.39)

In the case of a relativistic plasma at typical temperatures T >> 1 MeV, the flat-space conductivity (see Eq. (5.28)) is given by

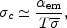(5.40)

where 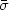~ _em² / T² is the cross section ⁽¹⁹⁾. Then, it can be shown [135] that

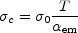(5.41)

where ₀ is a slowly increasing function of the temperature. In [135] it has been convincingly argued that ₀ ~ 0.06 at T ~ 100 MeV while it becomes 0.6 at T ~ 100 GeV (for a recent analysis of conductivity in high temperature QED see [136]). Recalling Eq. (5.28) the physical diffusivity scale = k / a() will be, in units of the Hubble parameter,

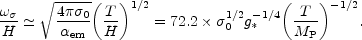(5.42)

Discounting for the effect due to the possible variations of the effective relativistic degrees of freedom, the (physical) magnetic diffusivity length scale is much smaller than the Hubble radius at the corresponding epoch. For instance, if T ~ 10 MeV, then L ~ ^-1 ~ 10^-10 H^-1. This means that there are ten decades length-scales where the magnetic field spectrum will not experience diffusion due to the finite value of the conductivity.

The estimate of the magnetic diffusivity scale will now be repeated in a different range of temperatures, namely for T < 0.2m_e when weak interactions have fallen out of thermal equilibrium. In this case the relevant degrees of freedom are the three neutrino species and the photon, i.e. g_* = 3.36. In this case the conductivity will be given by

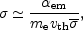(5.43)

which leads to

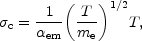(5.44)

if v_th ~ (T / m_e)^1/2 and 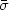~ _em² / T². Eq. (5.44) leads to the known T^3/2 dependence of the conductivity from the temperature, as expected from a non-relativistic plasma. The physical magnetic diffusivity momentum is, in this case

(5.45)

Eqs. (5.42) and (5.45) refer to two different ranges of temperatures. The magnetic diffusivity scale in units of the Hubble parameter obtained in Eq. (5.45) is smaller than the one given by in Eq. (5.42). Hence, by lowering the temperature across the weak equilibration temperature makes the conductivity length scale larger in units of the Hubble radius : inertial range ⁽²⁰⁾ of length scales (i.e. the region where conductivity effects are negligible) is larger above the weak equilibration temperature. Summarizing

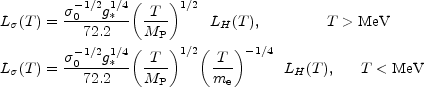(5.46)

where L_H(T) = H^-1(T).

In the evolution of the plasma it is sometimes relevant to estimate the thermal diffusivity scale setting a bound on the coherence of the velocity field and not of the magnetic field. There are however phenomena, like the propagation of MHD waves, where the excitations of a background magnetic field are coupled to the excitations of the velocity field. Generally speaking the typical correlation scale of the velocity field is always smaller, in various physical situations, than the typical correlation scale of the magnetic fields. The range of physical momenta affected by thermal diffusivity is bounded from above by the thermal diffusivity scale which can be obtained from the following relations

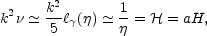(5.47)

were is the photon mean free path, i.e.

(5.48)

The mean free path measures the efficiency of a given process to transfer momentum. From Eqs. (5.29) and (5.47) the typical thermal diffusivity momentum is:

(5.49)

where _diff = k_diff / a is the physical momentum as opposed to the comoving momentum k_diff. The scale defined in Eq. (5.49) is sometimes called Silk scale. Notice that the mean free path given in Eq. (5.48) increases as a³(). Since the physical scale of an inhomogeneous region increases as a(), the photon mean free path can become larger than the physical size of an inhomogeneous region. This is the so-called free streaming regime.

If T < 0.2 m_e, the photon mean free path is given by Eq. (5.48) where 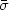_T = 1.7 × 10³ GeV^-2 _em m_e^-2 is the Thompson cross section and

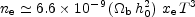(5.50)

is the electron number density depending both on the critical fractions of baryons, _b and on the ionization fraction x_e. The ionization fraction is the ratio between the number density of protons and the total number density of baryons. The value of x_e drops from 1 to 10^-5 in a short time around recombination. Eq. (5.50) is simply derived by noticing that, after electron positron annihilation, n_e 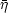n where 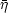= 2.74 × 10^-8 _b h₀² is the baryon-to-photon ratio, the free parameter of big-bang nucleosynthesis calculations [138, 139, 140, 141]. Thus

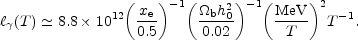(5.51)

For temperatures larger than the MeV the photon mean free path can be estimated by recalling that, above the MeV, the Thompson cross section is replaced by the Klein-Nishina cross section (i.e. 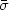~ T^-1 GeV^-1). Thus, from Eq. (5.48), the photon mean free path is

(5.52)

As before, it is useful to refer _diff to the Hubble rate and to compute the typical diffusion distances for T > 1 MeV

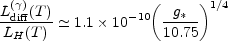(5.53)

and for T < 1 MeV

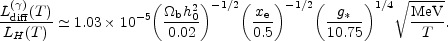(5.54)

At temperatures (MeV), corresponding roughly to e⁺ e^- annihilation, Eqs. (5.53) and (5.54) differ by a factor 10⁵. This drop of five orders of magnitude of the thermal diffusivity scale reflects the drop (by ten orders of magnitude) of the photon mean free path as it can be appreciated by comparing Eqs. (5.51) and (5.52) at T (MeV).

For temperatures smaller than the electroweak temperature (i.e. T ~ 100 GeV) down to the temperature of neutrino decoupling, neutrinos are the species with the largest mean free path and, therefore, the most efficient momentum transporters. Neglecting possible variations of the number of relativistic species the neutrino mean free path will be simply given by = G_F^-2 T^-2(n_lep + n_qua)^-1 where n_lep and n_qua are the leptons and quark number densities. Using this estimate the diffusion scale will be

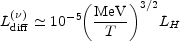(5.55)

which should be compared with Eq. (5.53). Clearly L_diff⁽⁾ > L_diff⁽⁾.

It seems then difficult to get observable effects from inhomogeneities induced by MHD excitations. In [137] the damping rates of Alfvén and magnetosonic waves have been computed and it has been shown that MHD modes suffer significant damping (see, however, [131, 132]). To avoid confusion, the results reported in Ref. [137] apply to the damping of MHD waves and not to the damping of magnetic fields themselves as also correctly pointed out in [142].

5.3.2. After matter-radiation equality

In standard big bang cosmology there are three rather close (but rather different) epochs,

• the matter-radiation equality taking place at T_eq 5.5 (₀ h₀²) eV, i.e. t_eq 4 × 10¹⁰ (₀ h₀²)^-2 sec;

• the decoupling of radiation from matter occurring at T_dec 0.26 eV i.e. t_dec 5.6 × 10¹² (₀ h₀²)^-1/2 sec

• the recombination occurring, for a typical value of the critical baryon fraction, at T_rec 0.3 eV, i.e. t_rec 4.3 × 10¹² (₀ h₀²)^-1/2 sec.

After plasma recombines, the baryons are no longer prevented from moving by viscosity and radiation pressure. Thus, the only term distinguishing the evolution of the baryons from the evolution of a cold dark matter fluid is the presence of the Lorentz force term in the Navier-Stokes equation. In this situation, it is interesting to investigate the interplay between the forced MHD regime and the generation of gravitational inhomogeneities. It was actually argued long ago by Wasserman [143] and recently revisited by Coles [144] that large-scale magnetic fields may have an important impact on structure formation. Assuming that, for illustrative purposes, the matter fluid consists only of baryons and cold dark matter, for t > t_rec the evolution of the system is described by the following set of non relativistic equations

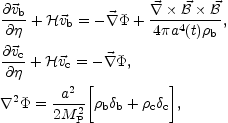(5.56) (5.57) (5.58)

where is the Newtonian potential. Eqs. (5.56) come from Eq. (5.31) for = 0 in the case of homogeneous pressure density. Eq. (5.57) can be obtained, in the same limit, from Eq. Eq. (5.31) by setting to zero the Lorentz force.

From Eq. (5.30), by linearizing the continuity equations for the two species, the relation between the divergence of the velocity field and the density contrast can be obtained

(5.59)

where _b = _b / _b and _c = _c / _c Thus, taking the divergence of Eqs. (5.56) and (5.57) and using, in the obtained equations, Eqs. (5.58) and (5.59) the following system can be obtained

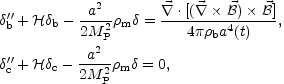(5.60) (5.61)

where = _{b b} + _{c c} and _m = _b + _c is the total matter density. Multiplying Eq. (5.60) by _b and Eq. (5.61) by _c, the sum of the two resulting equations can be written as

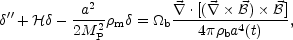(5.62)

where it has been used that the background geometry is spatially flat. Going to cosmic time, Eq. (5.62) reads

(5.63)

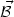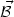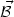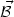The homogeneous term of Eq. (5.63) can be easily solved. The source term strongly depends upon the magnetic field configuration [88]. However, as far as time evolution is concerned, is constant in the inertial range of scales, i.e. L > L. Hence, = ₀ and (t) = ₀ / a²(t). Assuming a stochastic magnetic field the solution of equation (5.63) can be obtained integrating from t_rec and recalling that _b(t) ~ _b(t_rec) a^-3. The result is

(5.64)

where _B is the typical length scale of variation of the magnetic field. The total density contrast grows as t^2/3 with an initial condition set by the Lorentz force, i. e.

(5.65)

To seed galaxy directly the effective (t_rec) should be of the order off 10^-3 for a flat Universe, implying that

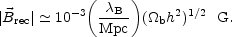(5.66)

Also the converse is true. Namely one can read Eq. (5.66) from left to right. In this case Eq. (5.66) will define the typical scale affected by the presence of the magnetic field. This scale is sometimes called magnetic Jeans scale [101]: it can be constructed from the Jeans length

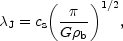(5.67)

where c_s is replaced by c_a, i.e. the Alfvén velocity.

¹⁹ The cross section is denoted with while the conductivity is . Back.

²⁰ In more general terms the inertial range is the interval of scales (either in momentum or in real space) where the dynamics is independent on the scales of dissipation so that, in this range, the difffusivities can be taken to zero. Back.