


The thickness of the gas layer in a disk galaxy can be used to measure the surface density of the disk. Assume the density distribution of the exponential, locally isothermal disk (that in eqn. (1) with n = 1). If the HI velocity dispersion < Vz2 > HI1/2 is independent of radius -as e.g. in the face-on spiral NGC 628, (Shostak & van der Kruit 1984) - and isotropic, and if the stars dominate the gravitational field, the HI layer has a full width at half maximum (to .5ex < /~ 3%) of
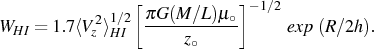 |
(15) |
So the HI layer increases exponentially in thickness with an e-folding 2h. This has first been derived and applied to HI observations of NGC 891 by van der Kruit (1981). One has to be careful to distinguish signatures for flaring from those of residual inclination away from exactly edge-on. Such studies can determine whether galaxies have in general maximum disks or not. The modelling, using photometry from van der Kruit & Searle (1981b), indicated that Vrot,disk of NGC 891 is ~ 140 km/s. The observed value is 225 ± 10 km/s, so the ratio in eqn.(1) is ~ 0.6, and NGC 891 is clearly sub-maximal.
For our Galaxy my preferred values are Vrot,disk ~ 155 ± 30 and Vrot,obs ~ 225 ± 10 km/s, so that the ratio is 0.69 ± 0.14 and the Milky Way also is sub-maximal. In other systems similar results were found; e.g. in NGC 4244 (Olling 1996b) deduced form the flaring a disk-alone rotation of 40 to 80% of that of observed rotation. Actually, the flaring of the HI layer in NGC 4244 was used by Olling (1996a) to infer that the dark matter is highly flattened (but see Olling & Merrifield (2000), who found for the Galaxy halo closer to spherical).
In a recent study by O'Brien et al. (2010) on the superthin edge-on galaxy UGC 7321, the rotation curve was decomposed using constraints from the thickness and flaring of the HI layer. This study was aimed at a determination of the shape of the dark matter halo, which was found to be close to spherical. The disk (I-band) M / L was found to be only about 0.2 and the galaxy is very far from maximum disk. This M / L is even somewhat lower than the range 0.5 to 2 indicated above. In fact, Banerjee et al. (2010) also concluded that the dark matter dominates the gravitational field everwhere in the disk of this galaxy.
Work on de compositions of rotation curves and analysis involving observations of the `baryonic' Tuly-Fisher relation has progressed as well. In particular, McGaugh (2005) has analysed a sample of galaxies with extended HI rotation curves and finds that high surface brightness galaxies are closer to maximum disk than low surface brightness ones. This may be the same effect as described above in terms of surface density. On the other hand, Weiner et al. (2001) find from detailed fluid dynamical gasflows in the barred galaxy NGC 4123 that this system must be close to maximum-disk. So, almost certainly some (massive) disks are maximal.
Finally I note the following clever argument of Courteau & Rix (1999) that makes use of the scatter in the Tully-Fisher relation. The amplitude of the rotation curve of the self-gravitating exponential disk is
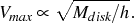 |
(16) |
For fixed disk-mass Mdisk we then get from differentiation
 |
(17) |
So at a given absolute magnitude (or mass) a lower scalelength disks should have a higher rotation velocity. If all galaxies were maximum disk this then should be visible in the scatter of the Tully-Fisher relation. This is not observed and the estimate is that on average Vdisk ~ 0.6 Vtotal and galaxies in general do not have maximal disks.
Recent reviews of disk masses in galaxies are by van der Kruit (2009) and McGaugh (2009) at the Kingston symposium. In summary: the overall state of affairs concerning the maximum disk hypothesis appears to be that in general galaxy disk are not maximal, except possibly the ones with the highest surface brightness and surface density.