


When we were describing the properties of the scalar field in
Section 5, we
emphasized its versatility. It can produce p =
+ for large
for large
 2 and
p = -
2 and
p = - for small
for small
 2.
2.
Belinskii and
Khalatnikov (1972),
who studied the singularity in a
space with a massless scalar field, obtained the stiff behavior p
= + (with a corresponding a(t)
(with a corresponding a(t)
 (t0 -
t)1/3
cosmological singularity; see
the original paper for details). The inflationary universe is based on the
p = -
(t0 -
t)1/3
cosmological singularity; see
the original paper for details). The inflationary universe is based on the
p = - or p
or p
 -
- situation created by the scalar
field. How can these
different approaches be reconciled? Or, more appropriately, what is the
rule for choosing one extreme case over the other?
situation created by the scalar
field. How can these
different approaches be reconciled? Or, more appropriately, what is the
rule for choosing one extreme case over the other?
The physical essence of the answer can be obtained by solving a simplified problem.
Assume a given constant rate of expansion H - this does not violate
any general principle, since one can imagine fields other than the scalar
field  that also contribute to the
energy density
that also contribute to the
energy density
 in the right-hand side
of the cosmological equation. These fields ensure that
in the right-hand side
of the cosmological equation. These fields ensure that
 = const and
H = const over the entire time under consideration. On the other
hand, we assume that these additional fields do not interact directly
with the scalar field
= const and
H = const over the entire time under consideration. On the other
hand, we assume that these additional fields do not interact directly
with the scalar field  . The
(inevitable) gravitational interaction
between the fields is transmitted via the expansion rate; this interaction
has already been included in the equation for
. The
(inevitable) gravitational interaction
between the fields is transmitted via the expansion rate; this interaction
has already been included in the equation for
 , which we rewrite here:
, which we rewrite here:
For constant H, this is a linear equation. The general solution
can easily
be obtained by a college freshman:
where
and the constants
We shall study the case in which the exponent is real (a complex
To do this, we assume that H is large: 9H2/4 >>
µ2. We shall discuss
two cases:
(1) H > 0: Expansion
In this case, the general solution is a sum of two decreasing solutions,
the first of which,
But the very fact that the solution is slowly decreasing obviously
means that
We shall now write down the formula for p /
Therefore,
As µ2 / H2 -> 0, p ->
-
It is instructive to see how the ratio p /
Since the mixed solutions are of two types, depending on whether
What is the salient characteristic of Fig. 6?
The general solutions
diverge from the upper dotted line and approach the lower dotted line.
One concludes that, in general, p
(2) H < 0: Contraction
It is now easy to study the case of contraction, i.e., H < 0, in
the same
way. We have two solutions in this case as well: a rapidly growing
solution and a slowly growing solution. The rapidly growing solution
dominates in the general case. We shall not carry out all of the trivial
calculations: Fig. 6 is valid with the time axis
reversed!
So we conclude that p
There is a moral to be learned from these simple calculations. The
result by and large conforms to Braun and Le Chatelier's principle, which
also holds true in human relations:
Every system resists outside forces.
The scalar field expands, and a negative pressure (or tension) builds
up. If the expansion were created by the motion of a piston in a cylinder
containing 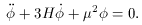
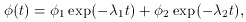

 1 and
1 and
 2 are determined by
the initial conditions.
2 are determined by
the initial conditions.
 would mean an oscillatory solution).
would mean an oscillatory solution).
 1
exp (-
1
exp (- 2t), is
slowly decreasing, and the second
of which,
2t), is
slowly decreasing, and the second
of which,  2 exp (-
2 exp (- 2t), is rapidly
decreasing. It is clear that in
general, when both
2t), is rapidly
decreasing. It is clear that in
general, when both  1
1
 0 and
0 and
 2
2
 0 and
0 and
 1 and
1 and
 2 are of the same
order, it is the slowly decreasing solution,
2 are of the same
order, it is the slowly decreasing solution,
 1 exp
(-
1 exp
(- 1t), that
survives
beyond the time span required to eliminate the rapidly decreasing
solution
1t), that
survives
beyond the time span required to eliminate the rapidly decreasing
solution  2 exp
(-
2 exp
(- 2t).
2t).
 2 is
small. So we obtain the qualitative result that the
solution which survives the expansion has a slow time dependence,
which means that
2 is
small. So we obtain the qualitative result that the
solution which survives the expansion has a slow time dependence,
which means that  2
is small and, consequently, that p
2
is small and, consequently, that p
 -
- .
.
 in the limiting case
H2 > µ2 for the "slow"
solution. Carrying out a series expansion, we obtain
in the limiting case
H2 > µ2 for the "slow"
solution. Carrying out a series expansion, we obtain
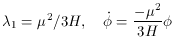
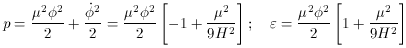
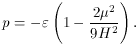
 .
.
 varies during the
expansion (see
Fig. 6, where the upper and lower horizontal
lines are the extreme
values p /
varies during the
expansion (see
Fig. 6, where the upper and lower horizontal
lines are the extreme
values p /  = +1 and
p /
= +1 and
p /  = -1; the upper
dotted line
describes p /
= -1; the upper
dotted line
describes p /  for
the pure rapidly decreasing
for
the pure rapidly decreasing
 2 exp
(-
2 exp
(- 2t)
solution (p /
2t)
solution (p /  = 1 -
2/9 µ2 / H2);
the lower dotted line is p /
= 1 -
2/9 µ2 / H2);
the lower dotted line is p /
 for the pure slowly
decreasing solution
for the pure slowly
decreasing solution
 1 exp
(-
1 exp
(- 1t),
which approaches - 1 at large H; and the mixed solutions
are shown by the dot-dash lines.
1t),
which approaches - 1 at large H; and the mixed solutions
are shown by the dot-dash lines.
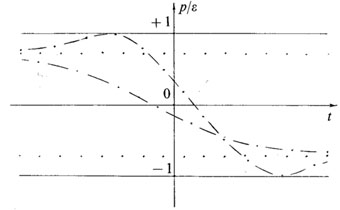
Figure 6.
 1 and
1 and
 2 have the same or
opposite sign, it is sufficient to calculate one
solution of each type; all others of a given type can be obtained by simple
translation along the t axis. The entire band between p /
2 have the same or
opposite sign, it is sufficient to calculate one
solution of each type; all others of a given type can be obtained by simple
translation along the t axis. The entire band between p /
 = -1 and
p /
= -1 and
p /  = 1 is
therefore filled, with two solutions going through every point.
= 1 is
therefore filled, with two solutions going through every point.
 -
- in the rapid expansion case.
in the rapid expansion case.
 +
+ for compression. That is
the end of the
story; the differences between the inflationary models and Belinskii and
Khalatnikov's work have been resolved.
for compression. That is
the end of the
story; the differences between the inflationary models and Belinskii and
Khalatnikov's work have been resolved.
 , the tension would
decelerate the piston. On the other
hand, if the field is being compressed, a positive pressure builds up,
producing a force opposing the motion of the piston. The above
remarks are very crude.
Belinskii, Grishchuk,
Khalatnikov, and the author (1985)
have analyzed the exact self-consistent equations of the
field and the universe in a detailed paper. This is not the right place to
give all of the results. The important result is that the general tendency
- the build-up of negative pressure due to expansion - is conformed.
, the tension would
decelerate the piston. On the other
hand, if the field is being compressed, a positive pressure builds up,
producing a force opposing the motion of the piston. The above
remarks are very crude.
Belinskii, Grishchuk,
Khalatnikov, and the author (1985)
have analyzed the exact self-consistent equations of the
field and the universe in a detailed paper. This is not the right place to
give all of the results. The important result is that the general tendency
- the build-up of negative pressure due to expansion - is conformed.