As is found from Fig. 8, the logarithmic distribution of the absolute pg magnitudes of galaxies of types E-So-Ir can be described by a straight line extending from the lower limit M = -10.6 to about M = -20; in the interval -20 to -22 the curve falls off rapidly. The magnitude distribution for the Sa-Sb-Sc group is represented by a bell-shaped curve, extending from about M = -15 to M = -22.
It is a very interesting fact that the luminosity function derived for the E-So-Ir group agrees with the curve suggested by Zwicky (1957, 1964) for the members of galaxy clusters. The dense clusters may possibly have populations that are comparable to the E-So-Ir population studied here; in any case, these clusters are practically free from Sa-Sb-Sc spirals. The agreement also extends to the inclination of the log. distribution line. A least-squares solution, based on the eight class frequencies that define the linear part of the distribution in Fig. 8, gives an inclination of 0.195 ± 0.007, a result that within the limits of the accidental errors agrees with Zwicky's coefficient of 0.2. In a recent investigation of the luminosity function of elliptical galaxies in the Virgo cluster, Abell and Eastmond (1968) have arrived at a curve of the same type and the same inclination (0.2). In earlier studies of some dense clusters (all members included) Abell (1962, 1964) has found inclination coefficients that are slightly larger.
It may be pointed out here that the shape and the inclination of the
linear distribution curve are not affected by the disturbance to be
expected from the accidental errors in the absolute magnitudes (or
absolute diameters). However, in accordance
with well-known relations of stellar statistics, the curve should be
corrected by a displacement towards fainter magnitudes by the amount
M =
0.195
2 /
2 log e, where
s is the mean error of M; if the error is 0.7 magn., as suggested
above, the displacement would be
M = +
0.11. On the other hand, it was found in the preceding section that
the absolute magnitudes of E-So galaxies, as derived by means of
eq. (1), would be
about 0:3 magn. too faint. Since, as far as can be ascertained from the
present material,
galaxies of these types make up 50-60% of all objects in the. E-So-Ir
group, the
average systematic correction to the absolute magnitudes would
approximately neutralize the disturbance caused by the accidental
magnitude errors. The distribution curve will be accepted without any
corrections.
With a good degree of approximation the distribution referring to the Sa-Sb-Sc spirals, in all 35, can be described by a normal error-curve with a mean absolute pg magnitude of -17.7 and a dispersion of 1.2 magn. The accidental magnitude errors will not affect the mean but will to some extent increase the dispersion. Since the results are based on comparatively small class frequencies, and since the right-hand half of the observed curve happens to agree well with the curve derived from galaxies of known redshifts, as will be shown below, no corrections have been applied. It is interesting to recollect that, except for a systematic displacement in magnitude, the distribution agrees rather nicely with the classical luminosity function derived by Hubble (1936) from a study of the brightest resolved stars in nearby galaxies; Hubble's material referred mainly to Sc and Sb spirals.
In order to make possible a more detailed study, the distributions referring to the more luminous magnitude classes have been reproduced in Fig. 10. The full curve (large open circles) and the broken curve (small open circles) correspond to the curves of Fig. 8. An absolute calibration has been introduced, giving the number of galaxies per magnitude class in 1 Mpc3. The calibration is based on the space density, 0.17 galaxies brighter than M = -15.0 per Mpc3, that is derived in sect. 14 - 15. It should be pointed out here that the distribution curves may be assumed to represent an average volume of space, since the satellite population investigated presumably approaches a random sample of all general field galaxies (cf. sect. 1).
 |
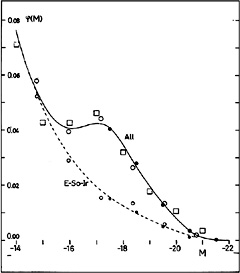Figure 10. The brighter end of the luminosity functions (corresponding to the right-hand half of Fig. 8), as calibrated for 1 Mpc3. The filled circles give the distributions derived for galaxies with known redshifts north of gal. lat +30°. The squares refer to the Local Group and the M81 group. |
In Fig. 10 two interesting comparisons are made with results available from other sources. The open squares represent the three nearby galaxy groups listed in Table 1, a material that is probably complete down to M = -13.5. The class frequencies are, except in the faintest class (five galaxies), overlapping means, 50% from the central class and 25% from each adjoining class. In spite of the small total number, only 18, there is a good agreement with the full curve. It should be remarked that the Milky way and M81 have been omitted, as being the central systems in the Local Group and the M81 group. The large filled circles represent all the galaxies in the redshift lists to be analyzed in sect. 15, whereas the small filled circles refer to the E-So-Ir galaxies alone. It is very satisfactory to find that the luminosity curves derived from the redshift material are in perfect agreement with the curves from the satellite groups, especially in view of the fact that in the brighter magnitude classes the former curves are based on a much larger number of objects.
To summarize the results obtained in this section, it can be stated that the total luminosity function (pg) referring to all galaxies in 1 Mpc3 (general field) is described by the expression:
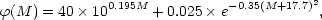
| (2) |
where the first part refers to types E-Sb-Ir, and the second part to
types Sa-Sb-Sc.
The formula represents the distribution from M = -10.6 to
M = -19 or -20; beyond
this limit the curve rapidly approaches zero. Whereas the total number
of galaxies is 0.17 per Mpc3 for magnitudes brighter than
-15.0, it increases to about 0.8 for M
-10.6. It should be
recollected that the luminosity function is based on a Hubble
parameter of 80 km/sec per Mpc.
In conclusion, some results will be given that refer to different classes of morphological type. In the interval M < -14.2 there are 34 E-So objects, 19 Ir I objects, and 8 Ir II objects, as is clear from Table 5. For the interval M < -15.0, where Sa-Sb-Sc spirals are more fully represented, the relative frequencies range from 34% (Sc) to 7% (Ir II). These figures naturally refer to physical members of the 160 satellite groups (optical companions eliminated). The mean absolute magnitude corresponding to a given volume of space cannot be determined for E-So-Ir galaxies; for Sa-Sb-Sc spirals the mean magnitude is -17.7, as stated above. It is however possible to compute, by means of the luminosity functions, the mean absolute magnitude that would correspond to a given class of apparent magnitude. The results are listed in the last column of Table 5. The mean magnitudes are to some extent based on a combination of the present data and the Holmberg (1964) catalogue, which is complete as regards galaxies of bright apparent magnitudes.
| Type | Number |  m |
| E-So | (34) 23 = 30% | -19.8 |
| Ir 1 | (19) 13 17 | -17.5 |
| Ir II | (8) 5 7 | (-19.0) |
| Sa-Sb | 9 12 | -20.0 |
| Sc | 26 34 | -19.2 |