2.4. Coupled perturbations

We will often be concerned with the evolution of perturbations in a universe that contains several distinct components (radiation, baryons, dark matter). It is easy to treat such a mixture if only gravity is important (i.e. for large wavelengths). Look at the perturbation equation in the form

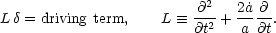(33)

The rhs represents the effects of gravity, and particles will respond to gravity whatever its source. The coupled equations for several species are thus given by summing the driving terms for all species.

Matter plus radiation     The only subtlety is that we must take into account the peculiarity that radiation and pressureless matter respond to gravity in different ways, as seen in the equations of fluid mechanics. The coupled equations for perturbation growth are thus

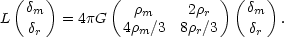(34)

Solutions to this will be simple if the matrix has time-independent eigenvectors. Only one of these is in fact time independent: (1, 4/3). This is the adiabatic mode in which _r = 4_m / 3 at all times. This corresponds to some initial disturbance in which matter particles and photons are compressed together. The entropy per baryon is unchanged, (T³) / (T³) = _m, hence the name `adiabatic'. In this case, the perturbation amplitude for both species obeys L = 4 G(_m + 8_r / 3). We also expect the baryons and photons to obey this adiabatic relation very closely even on small scales: the tight coupling approximation says that Thomson scattering is very effective at suppressing motion of the photon and baryon fluids relative to each other.

Isocurvature modes     The other perturbation mode is harder to see until we realize that, whatever initial conditions we choose for _r and _m, any subsequent changes to matter and radiation on large scales must be adiabatic (only gravity is acting). Suppose that the radiation field is initially chosen to be uniform; we then have

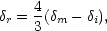(35)

where _i is some initial value of _m. The equation for _m becomes

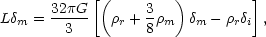(36)

which is as before if _i = 0. The other solution is therefore a particular integral with _i. For = 1, the answer can be expressed most neatly in terms of y _m / _r (Peebles 1987):

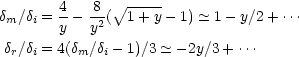(37)

At late times, _m 0, while _r -4_i / 3. This mode is called the isocurvature mode, since it corresponds to a total density perturbation / 0 as t_i 0. Subsequent evolution attempts to preserve constant density by making the matter perturbations decrease while the amplitude of _r increases. An alternative name for this mode is an entropy perturbation. This reflects the fact that one only perturbs the initial ratio of photon and matter number densities. The late-time evolution is then easily understood: causality requires that, on large scales, the initial entropy perturbation is not altered. Hence, as the universe becomes strongly matter dominated, the entropy perturbation becomes carried entirely by the photons. This leads to an increased amplitude of microwave-background anisotropies in isocurvature models (Efstathiou & Bond 1986), which is one reason why such models are not popular. Of course, a small admixture of isocurvature perturbations is always going to be hard to rule out (e.g. Bucher, Moodley & Turok 2002), so neglect of this mode is primarily justified by the fact that the simplest model for the generation of cosmological perturbations (single-field inflation) produces pure adiabatic modes. Models with multiple fields, such as the decaying curvaton of Lyth & Wands (2002) tend to generate order-unity isocurvature contributions, which are impossible to reconcile with CMB data (e.g. Gordon & Lewis 2002).

Baryons and dark matter     This case is simpler, because both components have the same equation of state:

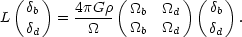(38)

Both eigenvectors are time independent: (1, 1) and (_d, - _b). The time dependence of these modes is easy to see for an = 1 matter-dominated universe: if we try tⁿ, then we obtain respectively n = 2/3 or -1 and n = 0 or -1/3 for the two modes. Hence, if we set up a perturbation with _b = 0, this mixture of the eigenstates will quickly evolve to be dominated by the fastest-growing mode with _b = _d: the baryonic matter falls into the dark potential wells. This is one process that allows universes containing dark matter to produce smaller anisotropies in the microwave background: radiation drag allows the dark matter to undergo growth between matter-radiation equality and recombination, while the baryons are held back.

This is the solution on large scales, where pressure effects are negligible. On small scales, the effect of pressure will prevent the baryons from continuing to follow the dark matter. We can analyse this by writing down the coupled equation for the baryons, but now adding in the pressure term (sticking to the matter-dominated era, to keep things simple):

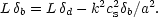(39)

In the limit that dark matter dominates the gravity, the first term on the rhs can be taken as an imposed driving term, of order _d / t². In the absence of pressure, we saw that _b and _d grow together, in which case the second term on the rhs is smaller than the first if kc_S t / a << 1. Conversely, for large wavenumbers (kc_S t/a >> 1), baryon pressure causes the growth rates in the baryons and dark matter to differ; the main behaviour of the baryons will then be slowly declining sound waves, and we can write the WKB solution.

(40)

where is conformal time. An alternative way to see that the baryons are damped is to write the coupled equations as

(41)

where k / k_J. In the special case _b 0 and = constant, a solution is clearly

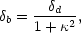(42)

and this is found to be the asymptotic solution in more general cases (Nusser 2000).

This oscillatory behaviour holds so long as pressure forces continue to be important. However, the sound speed drops by a large factor at recombination, and we would then expect the oscillatory mode to match on to a mixture of the pressure-free growing and decaying modes. This behaviour can be illustrated in a simple model where the sound speed is constant until recombination at conformal time _r and then instantly drops to zero. The behaviour of the density field before and after t_r may be written as

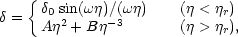(43)

where kc_S. Matching and its time derivative on either side of the transition allows the decaying component to be eliminated, giving the following relation between the growing-mode amplitude after the transition to the amplitude of the initial oscillation:

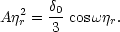(44)

The amplitude of the growing mode after recombination depends on the phase of the oscillation at the time of recombination. The output is maximised when the input density perturbation is zero and the wave consists of a pure velocity perturbation; this effect is known as velocity overshoot. The post-recombination transfer function will thus display oscillatory features, peaking for wavenumbers that had particularly small amplitudes prior to recombination. Such effects can be seen at work in determining the relative positions of small-scale features in the power spectra of matter fluctuations and microwave-background fluctuations.