4.3. Thermal Relics

As we have mentioned, particles typically do not stay in equilibrium forever; eventually the density becomes so low that interactions become infrequent, and the particles freeze out. Since essentially all of the particles in our current universe fall into this category, it is important to study the relic abundance of decoupled species. (Of course it is also possible to obtain a significant relic abundance for particles which were never in thermal equilibrium; examples might include baryons produced by GUT baryogenesis, or axions produced by vacuum misalignment.) In this section we will typically neglect factors of order unity.

We have seen that relativistic, or hot, particles have a number density that is proportional to T³ in equilibrium. Thus, a species X that freezes out while still relativistic will have a number density at freeze-out T_f given by

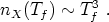(112)

Since this is comparable to the number density of photons at that time, and after freeze-out both photons and our species X just have their number densities dilute by a factor a(t)^-3 as the universe expands, it is simple to see that the abundance of X particles today should be comparable to the abundance of CMB photons,

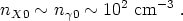(113)

We express this number as 10² rather than 411 since the roughness of our estimate does not warrant such misleading precision. The leading correction to this value is typically due to the production of additional photons subsequent to the decoupling of X; in the Standard Model, the number density of photons increases by a factor of approximately 100 between the electroweak phase transition and today, and a species which decouples during this period will be diluted by a factor of between 1 and 100 depending on precisely when it freezes out. So, for example, neutrinos which are light (m < MeV) have a number density today of n = 115 cm^-3 per species, and a corresponding contribution to the density parameter (if they are nevertheless heavy enough to be nonrelativistic today) of

(114)

(In this final expression we have secretly taken account of the missing numerical factors, so this is a reliable answer.) Thus, a neutrino with m ~ 10^-2 eV would contribute ~ 2 × 10^-4. This is large enough to be interesting without being large enough to make neutrinos be the dark matter. That's good news, since the large velocities of neutrinos make them free-stream out of overdense regions, diminishing primordial perturbations and leaving us with a universe which has much less structure on small scales than we actually observe.

Now consider instead a species X which is nonrelativistic or cold at the time of decoupling. It is much harder to accurately calculate the relic abundance of a cold relic than a hot one, simply because the equilibrium abundance of a nonrelativistic species is changing rapidly with respect to the background plasma, and we have to be quite precise following the freeze-out process to obtain a reliable answer. The accurate calculation typically involves numerical integration of the Boltzmann equation for a network of interacting particle species; here, we cut to the chase and simply provide a reasonable approximate expression. If ₀ is the annihilation cross-section of the species X at a temperature T = m_X, the final number density in terms of the photon density works out to be

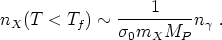(115)

Since the particles are nonrelativistic when they decouple, they will certainly be nonrelativistic today, and their energy density is

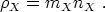(116)

We can plug in numbers for the Hubble parameter and photon density to obtain the density parameter,

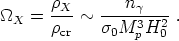(117)

Numerically, when = c = 1 we have 1 GeV ~ 2 × 10^-14 cm, so the photon density today is n ~ 100 cm^-3 ~ 10^-39 GeV^-3. The Hubble constant is H₀ ~ 10^-42 GeV, and the Planck mass is M_p ~ 10¹⁸ GeV, so we obtain

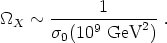(118)

It is interesting to note that this final expression is independent of the mass m_X of our relic, and only depends on the annihilation cross-section; that's because more massive particles will have a lower relic abundance. Of course, this depends on how we choose to characterize our theory; we may use variables in which ₀ is a function of m_X, in which case it is reasonable to say that the density parameter does depend on the mass.

The designation cold may ring a bell with many of you, for you will have heard it used in a cosmological context applied to cold dark matter (CDM). Let us see briefly why this is. One candidate for CDM is a Weakly Interacting Massive Particle (WIMP). The annihilation cross-section of these particles, since they are weakly interacting, should be ₀ ~ _W² G_F, where _W is the weak coupling constant and G_F is the the Fermi constant. Using G_F ~ (300 GeV)^-2 and _W ~ 10^-2, we get

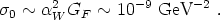(119)

Thus, the density parameter in such particles would be

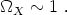(120)

In other words, a stable particle with a weak interaction cross section naturally produces a relic density of order the critical density today, and so provides a perfect candidate for cold dark matter. A paradigmatic example is provided by the lightest supersymmetric partner (LSP), if it is stable and supersymmetry is broken at the weak scale. Such a possibility is of great interest to both particle physicists and cosmologists, since it may be possible to produce and detect such particles in colliders and to directly detect a WIMP background in cryogenic detectors in underground laboratories; this will be a major experimental effort over the next few years [13].