2. EARLY ACCRETION DISK MODELS

Even if one assumes that angular momentum plays an important role in the dynamics of the central accretion flow, the flow geometry is still very uncertain. The simplest assumption that one can make is that the radial dynamics of the flow is dominated by gravitation and rotation, with radiation pressure, gas pressure, and magnetic forces being negligible by comparison. The plasma in the disk then orbits the black hole on nearly circular test particle ("Keplerian") orbits, and the flow takes the form of a geometrically thin disk. The vertical extent of this disk is determined by balancing the small (by assumption!) internal pressure of the disk with the small vertical component of gravity from the central black hole. At large radii, self-gravity can also become important. The thin disk assumption necessarily breaks down at high accretion luminosities approaching the Eddington limit, where radiation pressure becomes important. In addition it may not be a good assumption at very low accretion rates. We will discuss these complications further in Section 5 below.

The fundamental uncertainty that plagues all theoretical models of accretion disks is the mechanism of outward angular momentum transfer, which is responsible for the slow inward spiral of material that converts gravitational potential energy into radiation and/or an outflow. There are two particularly promising mechanisms for angular momentum transfer in disks, both of which involve magnetic fields. The first is a magnetohydrodynamic (MHD) outflow, where magnetic fields supply torques to the disk and carry away angular momentum in the outflow (Blandford & Payne 1982). The second is internal MHD turbulence, as seen in simulations of the nonlinear development of the Balbus-Hawley instability (Balbus & Hawley 1991; Stone et al. 1996; Balbus & Hawley 1998). Very few models of the radiation produced in the accretion flow are based on these ideas, however, and generally angular momentum transport is treated through a parameterized viscous stress. The most popular prescription is the -viscosity of Shakura & Sunyaev (1973), in which the radial/azimuthal component of the viscous stress is taken to be a parameter times the total (gas plus radiation) pressure. We will have very little to say here about the detailed mechanisms whereby gravitational energy is converted to radiative and kinetic power in AGNs. Nevertheless, until this is understood, models and interpretation of observations will be severely handicapped.

The basic theoretical models of stationary, geometrically thin disks around black holes were developed by Shakura & Sunyaev (1973) and Novikov & Thorne (1973) (see also Page & Thorne 1974; Eardley & Lightman 1975; Riffert & Herold 1995; and Abramowicz, Lanza, & Percival 1997 for important corrections and refinements to these fundamental papers). From the point of view of predicting the observed spectrum, such models are the most well constrained of all the possible accretion flows considered to date. They make several key assumptions about the flow. In particular, the disk is stationary and axisymmetric and extends down to the innermost stable circular orbit, where zero stress is assumed to be exerted on the disk. Inside this point material falls quickly into the hole and emits very little radiation. Angular momentum transport within the disk occurs by local "viscous" stresses that convert gravitational energy entirely into heat. Because the disk is thin, this heat is assumed to flow vertically out of the disk, thereby being emitted at the same radius as it was generated. Under these assumptions, the radiative flux emerging from each face of the disk at radius r is given by

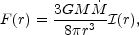(1)

where M is the mass of the black hole, is the mass accretion rate, and (r) is a correction factor that depends on the mass and angular momentum of the hole and approaches unity at large radii. The local disk flux therefore depends only on the properties of the black hole and the accretion rate. At large radii, F(r) r^-3, which implies that the effective temperature of the disk surface varies as T_eff r^-3/4.

The geometry of the thin disk allows one to calculate the overall emergent spectrum from this total flux by dividing the disk into concentric annuli, calculating the spectrum emitted by each annulus and then summing them all together. The very simplest assumption is that each annulus radiates like a blackbody. The r^-3/4 effective temperature distribution at large radii then gives a long wavelength SED of F ^1/3. As we discuss in Section 3.1 below, this is much bluer than is typically observed in the optical/UV spectra of AGNs.

Blackbody emission is unlikely to be a good approximation to the spectrum at each radius, even if the disk were optically thick, which it may not be! Careful modeling of the vertical structure and radiative transfer through each annulus is necessary to calculate the overall emitted spectrum. In contrast to the radial structure, the vertical structure of the disk is highly dependent on the assumed mechanism of angular momentum transfer, including its vertical dependence. Vertical energy transport can proceed by bulk motions of plasma and magnetic fields as well as by radiation through a supposedly stationary medium. Magnetic and turbulent stresses may well contribute to the vertical pressure support. The disk can be effectively optically thin in the vertical direction, particularly in the innermost regions if the viscosity is high. Even in models in which the disk is optically thick and vertical energy transport occurs through radiation, stellar atmosphere modeling is necessary. In addition to atomic opacity sources, including lines broadened by turbulence, electron scattering (both Thomson and Compton) is crucial in determining both the radiative transfer and the vertical disk structure. High temperatures and low densities mean that many atomic level populations are strongly coupled to the radiation field, and non-LTE effects are important. Illumination of the disk photosphere by external sources of X-ray radiation with significant power is also important, particularly in Seyfert galaxies. Relativistic effects must be included. Gravitational light bending will change the flux distribution through absorption and reprocessing (as will flaring or warping of the outer disk). Corrections must be made for the relativistic "transfer function" (Cunningham 1975; Speith, Riffert, & Ruder 1995; Agol 1997); i.e., the spectrum observed at infinity is altered by Doppler shifts, aberration, gravitational redshifts, and light bending. Radiation from disks around rapidly rotating Kerr holes can also be focused back onto the disk, modifying the emerging spectrum (Cunningham 1976). Given the enormous complexities and uncertainties, it is essential that models pay close attention to, and be guided by, observations.

A crude, first-order modification to the blackbody assumption is to take into account the fact that Thomson scattering opacity may dominate absorption opacity in the innermost regions of the accretion flow. The spectrum at each annulus may then be crudely modeled as a modified blackbody. This flattens the SED and produces more high-energy photons (Shakura & Sunyaev 1973). This is an important effect in accretion disks around stellar mass black holes, but whether or not it occurs in the disks of AGNs depends on the detailed vertical structure of the disk (see Section 4).

Shields (1978) proposed that the optical continuum in AGNs could be due to thermal emission from an accretion disk. He fitted the SED of 3C 273 by a nonthermal power law that extended from the infrared to the X-rays with an additional thermal component in the optical/UV. Thus, the BBB was for the first time associated with thermal accretion disk radiation. The nonthermal continuum prescription continued to be used in later models (Malkan 1983; Sun & Malkan 1989; Laor & Netzer 1989; Laor 1990) to account for the flat optical continua observed in the spectra of AGNs (see Section 3.1).

Multiwavelength observations of other AGNs were first compared to accretion disk models by Malkan (1983). In his comparisons, there were three components: a nonthermal infrared-to-X-ray power-law spectrum, a thermal accretion disk spectrum, and a recombination spectrum. The accretion disk models in this early work were just face-on disks in which the emergent flux was described by blackbodies. The models did account for relativistic effects in the disk structure as well as the effects of the relativistic transfer function. The results of this work showed that accretion was at super-Eddington rates in bright QSOs, where the assumptions of these accretion disk models break down. This work also discussed many observational tests for accretion disks. Sun & Malkan (1989) extended this work to include disk inclination angle effects and fitted many more sources. However, the fits relied crucially on the assumption of an underlying infrared power law, and the emergent flux was still approximated by blackbodies.

The earliest attempt at calculating the detailed overall spectrum of optically thick, geometrically thin accretion disks using stellar atmosphere models appropriate for AGNs was by Kolykhalov & Sunyaev (1984). They used the -viscosity prescription to calculate the vertical structure of each annulus. They then took existing stellar atmosphere models from the libraries existing at that time (e.g., Kurucz 1979) with appropriate surface gravities and effective temperatures and then summed them together to generate overall spectra. The most interesting result of their calculations was the existence of very large absorption edges at the hydrogen Lyman limit - edges that existed even when the smearing effects of the relativistic transfer function were included. As we discuss in Section 3.2 below, such edges are not observed, and they noted this discrepancy at the time. This problem motivated much of the later theoretical and observational work on the Lyman edge region of the spectra of AGNs. But as we discuss in Section 4, these early calculations suffered from severe limitations.

Because electron-scattering opacity is important in the disk atmosphere at the expected temperatures and densities, the radiation emerging from the disk can be substantially polarized. Most of the early work assumed that the polarization would be similar to that emerging from an optically thick, pure electron-scattering atmosphere. In this case the polarization is perpendicular to the disk axis and has a strong aspect angle dependence, varying from 0% for a face-on disk (because of symmetry) to 11.7% for an edge-on disk (Chandrasekhar 1960; Rees 1975; Lightman & Shapiro 1975). Pioneering work in the 1980s showed that optical polarization in AGNs is generally much lower ( 1%) than predicted by these simple models. Often no polarization is detected, and the polarizations are parallel to the nuclear axes, when the latter can be inferred from a radio jet position angle (Stockman, Angel, & Miley 1979; Antonucci 1988).