


6.2. The Higgs Field
It was at this dramatic moment that the idea of the scalar field was born anew, albeit in a different role.
If a vector particle has a mass m, this means that the energy density of the vector field A contains a term m2A2. The field equation for a free field describing massive vector particles is
Compare (6.2.1) with the corresponding equation for the scalar field
(Section 5) and check the role of the
m2A term. The energy density of the
vector field now contains a term
m2A2/2 in addition to the
m2
The new idea now consists of considering an initially massless A
interacting with the charged scalar field
When we say that
The energy density of the scalar field contains the term
The interaction with A means that each space or time derivative is
transformed into a combination involving A:
The sum of the squares of the derivatives yields the characteristic
term (7)
This is, in effect, a mass term for the A field, if the average
<
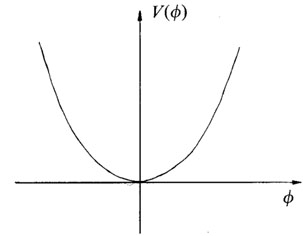
Figure 1.
How can this be arranged? The idea proposed by Higgs was to
introduce the potential
in the initial equation for the energy density of the scalar field in place
of the simple
(see Figs. 1 and 2); in
the simple case Fig. 2, the lowest state is,
obviously, symmetric:
Figure 2.
In both cases (+, -), the equation for the vector field (return to the
term e2
The equation for the vector potential has all of the required experimentally
verified properties: short range of action and large mass of the
particles associated with A (the W and Z fields
being examples of A).
On the other hand, when it comes to the higher-order corrections and
renormalization, it turns out quite magically that all of our difficulties
are over! When we had "manually" inserted the mass of A as a
constant, this led to nonrenormalizability, i.e., an unacceptable theory.
The same mass does not spoil the theory as long as it is due to
interaction with the scalar field
Put in a nutshell, the story is really funny: first the scalar field was
invented; it was then pushed out by the vector fields; but it finally turns
out that the massive vector fields themselves need scalar Higgs fields in
order to survive.
7 To be exact, this term is needed in the
Lagrangian, not in the energy density. The
difference is important for real calculations; however, in that case,
one must read other
papers not this one. As far as one's understanding of the general idea
goes, this is splitting hairs. Back.

 2/2 term
that appears in the energy density of the scalar field. The quantum
theory of an equation like this with an m2A
term in the right-hand side
leads nowhere, in contrast to the successful QED (electrodynamics with
massless photons) case.
2/2 term
that appears in the energy density of the scalar field. The quantum
theory of an equation like this with an m2A
term in the right-hand side
leads nowhere, in contrast to the successful QED (electrodynamics with
massless photons) case.
 .
.
 has a "charge",
we don't mean electric charge,
but the charge that describes the interaction with the vector field
A (for
example, that associated with the W± or
Z0 vector fields, or any other
vector field other than the electromagnetic field).
has a "charge",
we don't mean electric charge,
but the charge that describes the interaction with the vector field
A (for
example, that associated with the W± or
Z0 vector fields, or any other
vector field other than the electromagnetic field).
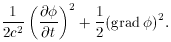

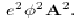
 2> is not zero.
2> is not zero.
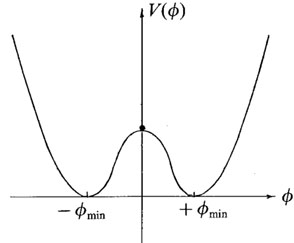
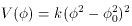
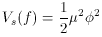
 = 0. But the
first, more complicated case is chosen precisely in order to have the minima
Vmin(
= 0. But the
first, more complicated case is chosen precisely in order to have the minima
Vmin( m)
= 0 occur at
m)
= 0 occur at
 m =
±
m =
±  0. The more
complicated case is still symmetric
V(
0. The more
complicated case is still symmetric
V( ) =
V(-
) =
V(- ), but the center of
symmetry at
), but the center of
symmetry at  = 0 is now a maximum. At
least at low temperatures, the system must be in one of the two states
= 0 is now a maximum. At
least at low temperatures, the system must be in one of the two states
 =
+
=
+ 0 or
0 or
 =
-
=
- 0 (see
Fig. 1 above).
0 (see
Fig. 1 above).
 2A2) is in
effect identical to the equation for a massive vector field:
2A2) is in
effect identical to the equation for a massive vector field:
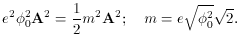
 ; the extra virtual processes with
; the extra virtual processes with
 cancel out the virtual processes with A (with
<
cancel out the virtual processes with A (with
< 2>
2>
 0); the theory is
renormalizable and acceptable to highbrow theoreticians. Putting m in
directly is called the "hard method" of introducing the mass; while the
e
sqrt[2<
0); the theory is
renormalizable and acceptable to highbrow theoreticians. Putting m in
directly is called the "hard method" of introducing the mass; while the
e
sqrt[2< 20>]
method is called the "soft method". The soft method is
logically impeccable, but it necessarily involves introducing a new field
- a scalar field with the peculiar energy term
V(
20>]
method is called the "soft method". The soft method is
logically impeccable, but it necessarily involves introducing a new field
- a scalar field with the peculiar energy term
V( ) - in
Fig. 1, the so-called Higgs scalar field.
) - in
Fig. 1, the so-called Higgs scalar field.