Copyright © 1992 by Annual Reviews. All rights reserved
| Annu. Rev. Astron. Astrophys. 1992. 30:
311-358 Copyright © 1992 by Annual Reviews. All rights reserved |
 (i), relative
to the direct ray in the absence of the lens, is given by
(Refsdal 1964b,
Cooke &
Kantowski 1975,
Kayser & Refsdal
1983,
Borgeest 1983,
Schneider 1985)
(i), relative
to the direct ray in the absence of the lens, is given by
(Refsdal 1964b,
Cooke &
Kantowski 1975,
Kayser & Refsdal
1983,
Borgeest 1983,
Schneider 1985)
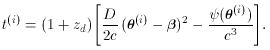
The quantity t (i) itself cannot be measured, but the
relative time delay between two images, t (ij) =
t (i) - t (j), can be. Since D
(cf Equation 4) is inversely proportional to H0, a
measurement of t (ij) provides vital information for
cosmography (cf Section 4.1).
The term proportional to ( (i) -
(i) -  )2 in Equation
11 is due to the geometrical excess path length and the
term proportional to
)2 in Equation
11 is due to the geometrical excess path length and the
term proportional to  is the
gravitational time delay,
known as the ``Shapiro effect'' in the solar system
(Shapiro 1964).
is the
gravitational time delay,
known as the ``Shapiro effect'' in the solar system
(Shapiro 1964).
 ) is taken to be
the fundamental quantity and the lens
Equation 2 is obtained by invoking Fermat's principle, which
states that images are formed at stationary points of t
(Weyl 1922,
Schneider 1985,
Blandford &
Narayan 1986).
Equation 11 can be generalized to multiple lenses
(Blandford &
Narayan 1986,
Kovner 1987b),
as well as to nonstationary gravitational fields
(Kovner 1990,
Nityananda &
Samuel 1992).
) is taken to be
the fundamental quantity and the lens
Equation 2 is obtained by invoking Fermat's principle, which
states that images are formed at stationary points of t
(Weyl 1922,
Schneider 1985,
Blandford &
Narayan 1986).
Equation 11 can be generalized to multiple lenses
(Blandford &
Narayan 1986,
Kovner 1987b),
as well as to nonstationary gravitational fields
(Kovner 1990,
Nityananda &
Samuel 1992).