m and 
are densities expressed in units of the critical
density
which is defined as 
crit = 3H02 /
8
G, where
G is Newton's constant. Thus,
m = m / crit where
m is
the mass density of the universe in grams per cubic centimeter.
The dimensionless cosmological constant =
/ crit = / 3H02 where
is the cosmological constant
introduced in 1917
[A. Einstein, Sitzungsber. Preuss.
Akad. Wiss. Phys. Math. Kl., 142 (1917), English translation in
Principle of Relativity, H.A. Lorentz et al.
(Dover, New York, 1952) p. 175].
S.D.M. White, J.F. Navarro, A.E. Evrard, C.S. Frenk, Nature 366, 429 (1993).
C.H. Lineweaver and D. Barbosa, Astrophys. J. 496, 624 (1998).
S. Perlmutter et al., ibid., in press, (available at http://xxx.lanl.gov/abs/astro-ph/9812133).
A. G. Riess et al., Astron. J. 116, 1009 (1998). t0flat = 15.2 ± 1.7 results from including a flat prior in the analysis.
Galaxy cluster mass-to-light ratio limits are from R. Carlberg et al., [Astrophys. J. 478, 462 (1997)] and R. Carlberg et al., [paper presented at the 33rd Rencontres de Moriond, Fundmental Parameters in Cosmology, Les Arcs, France, 17 to 24 January, 1998 (available at http://xxx.lanl.gov/abs/astro-ph/9804312)]. The error bars in Table 1 are conservative in the sense that they include their "worst case", 73% errors.
N.A. Bahcall and X. Fan,
Astrophys. J. 504, 1 (1998);
N.A. Bahcall, X. Fan, R. Cen,
ibid. 485, L53 (1997).
Table 1 lists their reported 95% errors,
which I use as 1
error bars in
this analysis.
E.J. Guerra, R.A. Daly, L. Wan,
in preparation, (available at
http://xxx.lanl.gov/abs/astro-ph/9807249).
I have doubled the 1 errors
quoted in the abstract.
C.H. Lineweaver, Astrophys. J. 505, L69 (1998).
M. White, ibid. 506, 495 (1998), A.M. Webster et al. ibid. 509, 65 (1998), E. Gawiser and J. Silk, Science 280, 1405 (1998).
M. Tegmark,
Astrophys. J. 514, L69 (1999).
Gravitational and reionization effects were included in this
analysis weakening the CMB constraints on closed models (upper right of
the m -
plane).
G. Efstathiou, S.L. Bridle, A.N. Lasenby, M.P. Hobson, R.S. Ellis, Mon. Not. R. Astron. Soc. 303, 47 (1999).
J.E. Felten and R. Isaacman, Rev. Mod. Phys. 58, 689 (1986). S.M. Carroll, W.H. Press, E.L. Turner, Annu. Rev. Astron. Astrophy. 30, 499 (1992) Eq. 17. There may be some ambiguity about what the "age of the universe" really means. I have used the Friedman equation derived from general relativity to extract the age of the universe. This age is the time that has elapsed since the early moments of the universe when the classical equations of general relativity became valid. In eternal inflation models [see, for example, A. Linde, Particle Physics and Inflationary Cosmology (Harwood Academic, Chur, Switzerland) p. 292ff (1990)] or other multiple universe scenarios ("multiverses"), the age of the universe is more complicated. If we live in a multiverse, then the age computed here refers only to the age of our bubble-like part of it.
A.E. Evrard, Mon. Not. R. Astron. Soc. 292, 289 (1997).
S. Burles and D. Tytler, Astrophys. J. 499, 699 (1998).
B.F. Madore et al. (available at
http://xxx.lanl.gov/abs/astro-ph/9812157) report
h = 0.72
(± 0.05)r[± 0.12]s from an analysis
which combines recent H0 measurements. The errors are
random and systematic, respectively.
F. Hoyle, G. Burbidge and J.V. Narlikar
[Mon. Not. R. Astron. Soc., 286, 173, 1997]
reviewed the observational literature and concluded
h = 0.58+0.10-0.05.
Both h = 0.65 ± 0.10 and h = 0.70 ± 0.10
are often cited as standard estimates
[see for example (12, 18)].
I adopt h = 0.68 ± 0.10 to represent the world's efforts
to measure the Hubble constant,
but I also explore the h dependence of the age results in Table 2.
In the likelihood,
Hubble,
I distinguish the observationally determined estimate
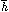from the free parameter h.
Y. Yoshii, T. Tsujimoto, K. Kawara, Astrophys. J. 507, L113 (1998).
M. Fukugita, C.J. Hogan, P.J.E. Peebles, ibid. 503, 518 (1998).
Baryonic fraction of the mass of clusters of galaxies.
Evrard (14) reports
m /
b = (12.5
± 0.7) h4/3 (independent of
)
which becomes m /
b= (12.5 ± 3.8)
h4/3 when the suggested 30% systematic error
is added in quadrature. Burles & Tytler
(15) report
b
h2 = 0.019
± 0.001 but Fukugita et al. (18) favor a lower
but larger range which
includes b
h2 = 0.020. I adopt b h2 = 0.015 ± 0.005.
When this is combined with the Evrard (14) result, one
gets the constraint:
m
h2/3 = 0.19 ± 0.12.
These five i have no simple analytic form.
These combinations prefer the upper left region of
the (m, ) plane. I
(9) have analyzed this complementarity
and obtained
=
0.62 ± 0.16 and m = 0.24 ± 0.10.
The addition of the cluster abundance evolution measurements and
improvements in the SNe measurements tightens these limits to the values
given.
(h,
m,
) was
derived as in (9).
For a discussion of taking the maximum of the likelihood, rather than
doing an integral
to marginalize over the nuisance variable, see
(3, p. 626) and
(11, Section 2.4) and references therein.
The lower right of Fig. 3A shows that low h values are preferred
(which correspond to older ages because t0
1 /
h).
This preference contrasts with the iso-t0 lines
(Fig. 3A)
which have the younger ages in the lower right.
Therefore, the three-dimensional CMB preferences will yield tighter
limits on t0
than is apparent from the figure.
In the absence of any direct constraint on h, the data are more
consistent with large values of h.
For example, if no Hubble(h) is used in Eq. 1, the
combined data yield a lower limit h > 0.67. The corresponding age
limit is t0 < 14.2 Ga .
No upper limit could be determined since the
best fit for this data is h = 1.00, at the edge of the range
explored.
This preference for higher h values (lower ages) helps explain the
h dependence of the results in
Table 2.
Results for 0.60 
h
0.80 are reported in
Table 2.
When a small uncertainty in h was assumed
(effectively conditioning on an h value), it
dominated the h information from the other constraints.
For example, although the central h values are the same, the
results from
using h = 0.64 ± 0.02 and h = 0.64 ± 0.10
are quite different: 14.6 and 13.5 Ga, respectively
(Fig. 2).
If t0 1 /
h were adhered to, there would be no difference.
P. Stetson, D.A. VandenBerg, M. Bolte, Publ. Astron. Soc. Pac. 108, 560 (1996).
T.D. Oswalt, J.A. Smith, M.A. Wood, P. Hintzen, Nature 382, 692 (1996); J.D. Oswalt, personal communication. Together, these references provided the age that is referred to as ``Oswalt98'' in Fig. 2.
S.P. Driver, et al., Astrophys. J. 496, L93 (1998). But there is no consensus on this issue. See F.R. Marleau and L. Simard, ibid. 507, 585 (1998).
E.E. Falco, C.S. Kochanek, J.A. Munoz, ibid. 494, 47 (1998).
Y.N. Cheng and L.M. Krauss, in preparation (available at http://xxx.lanl.gov/abs/astro-ph/9810393).
A.R. Cooray, in preparation, (available at http://xxx.lanl.gov/abs/astro-ph/9811448).
R. Quast and P. Helbig, in preparation, (available at http://xxx.lanl.gov/abs/astro-ph/9904174).
I. Zehavi, paper presented at the MPA/ESO Cosmology Conference, Evolution of Large-Scale Structure: From Recombination to Garching, 2 to 7 August 1998 (available at http://xxx.lanl.gov/abs/astro-ph/9810246).
A. Dekel, D. Burstein, S.D.M. White, in Critical Dialogues in Cosmology N. Turok, Ed. (World Scientific, River Edge, NJ 1997), pp. 175-191.
P.M. Garnavich et al., Astrophys. J. 509, 74 (1998).
S. Perlmutter, M.S. Turner, M. White, available at http://xxx.lanl.gov/abs/astro-ph/9901052.
I use SNe constraints large enough to encompass the constraints from the
two SNe groups.
Perlmutter etal. (4) report 0.8*m - 0.6* = -0.2
± 0.1. Evaluated at = 0
this yields
m=0 = -0.25 ± 0.13.
Assuming spatial flatness, they report
mflat =
0.28+0.09-0.08
(+0.05-0.04) statistical
(systematic) errors respectively.
Adding the statistical and systematic errors in quadrature yields
mflat
= 0.28+0.10-0.09.
The Riess etal. (5) constraints are from their figures
6 and 7 ("MLCS method" and "snapshot method")
using either the solid or dotted contours whichever is larger
(corresponding to the analysis
with and without SN1997ck, respectively). This yields
m=0 = -0.35 ± 0.18 and
mflat =
0.24+0.17-0.10.
I use the weighted average of these Perlmutter etal.
(4) and Riess
etal. (5) constraints
to obtain the SNe constraints listed above. The crucial upper error
bars on m are large
enough to include the constraints from either reference.
One would like to be less Galactocentric, but measuring the ages of even nearby extragalactic objects is difficult. See K.A. Olsen etal. [Mon. Not. R. Astron. Soc., 300, 665 (1998)] who find that the ages of old globular clusters in the Large Magellanic Cloud are the same age as the oldest Galactic globular clusters.
D.B. Guenther and P. Demarque, Astrophys. J. 484, 937 (1997).
B. Chaboyer, E.M. Green, J. Liebert, available at http://xxx.lanl.gov/abs/astro-ph/9812097.
S.K. Leggett, M.T. Ruiz, P. Bergeron, Astrophys. J. 497, 294 (1998).
G. Carraro, A. Vallenari, L. Girardi, A. Richichi, Astron. Astrophys. 343, 825, (1999). (available at http://xxx.lanl.gov/abs/astro-ph/9812278).
41. R.L. Phelps, Astrophys. J. 483, 826 (1997).
42. B. Chaboyer, P. Demarque, P.J. Kernan, L.M. Krauss, ibid. 494, 96 (1998).
R.G. Gratton et al., ibid. 491, 749 (1997).
I.N. Reid, Astron. J. 114, 161 (1997).
M. Salaris and A. Weiss, Astron. Astrophys. 327, 107 (1997).
F. Grundahl, D.A. VandenBerg and M.T. Andersen, Astrophys. J. 500, L179 (1998).
J.J. Cowan et al., ibid. 480, 246 (1997).
R. Jimenez, available at http://xxx.lanl.gov/abs/astro-ph/9810311.
F. Pont, M. Mayor, C. Turon, D.A. VandenBerg, Astron. Astrophys. 329, 87 (1998).
L.M. Krauss and G.D. Starkman, in preparation, (available at http://xxx.lanl.gov/abs/astro-ph/9902189).
I thank R. De Propris for helpful discussions on the Galactic age estimates of Table 2. I acknowledge a Vice-Chancellor's Fellowship at the University of New South Wales.