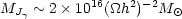8.5. Pregalactic radiation sources and explosive theories of galaxy formation

One of the most important problems in astronomy concerns the nature of missing mass. Whilst the dark matter could be in the form of weakly interacting particles, Occam's Razor invites us to associate its origin with early star formation and galactic evolution. A crucial question concerns the masses of the first generation of stars to form from primordial gas soon after recombination. Whilst there are some theoretical arguments which indicate a lower mass limit of ~ 10M, theory is not yet refined enough to be convincing (see Silk, 1983b; Kashlinsky and Rees, 1983). There are, however, some observational arguments (Terlevich, 1982) which indicate that the mass spectrum of stars is dependent on metallicity in the sense that for lower metal abundance the spectrum is weighted towards high mass stars.

However, we cannot at present rule out the possibility that most of the dark matter is in low-mass stars. Limits on the mass-to-light ratios in the extended halos of spiral galaxies indicate that low-mass stars ( 0.1 M) of spectral type M8 or later would be required (Hegyi and Gerber, 1977). Stars less massive than ~ 0.08 M would never have undergone hydrogen burning and would be extremely difficult to detect. One novel test has been proposed by Gott (1981). Low-mass stars in galaxy halos could act as gravitational lenses leading to variability in the intensities of quasar images on time-scales of ~ several years but so far the effect has not been observed.

Another possibility is that very massive objects (VMO's) form in the mass range 10² - 10⁵ M (Carr, Arnett and Bond, 1982; Arnett et al., 1983). Arnett, Bond and Carr suggest that the oxygen cores of a VMO would collapse to a black hole if the precursor hydrogen star has a mass in excess of M_crit ~ 200 - 500 M. The large uncertainty in M_crit arises because it is difficult to calculate how much mass is lost in the hydrogen and helium burning phases. VMO's offer a way of producing black hole remnants without producing too many metals, though if most of the mass is to end up in black hole remnants, in order to explain the high mass-to-light ratios of clusters of galaxies, one may require several generations of VMO'S.

Pregalactic stars of mass < M_crit may help to solve several out-standing problems. Pregalactic enrichment may explain the lack of low metallicity stars in the galaxy (Truran and Cameron, 1971) and various abundance anomalies such as the high ratios of O/Fe and N/Fe observed in metal-poor stars (Sneden, Lambert and Whitaker, 1979; Edmunds and Pagel, 1978). There are tentative indications of spectral distortions in the microwave background (Woody and Richards, 1979). Rowan-Robinson, Negroponte and Silk (1979) suggest that this effect may be explained if a substantial fraction of the background radiation ( 25%) was generated by pregalactic stars and the radiation was thermalized by grains produced by the stars.

It may even be possible to produce the entire microwave background if pregalactic stars form at a redshift of ~ 10³ (Rees, 1978) in which case one could contemplate a cold big-bang model (s = photon 1) or a tepid model (1 << s << 10⁸). A VMO may return 20-50% of its mass as helium before the oxygen core phase. If most of the stars have masses > M_crit one may be able to explain the observed helium abundance of 25% in a cold big bang model without overproducing heavy elements (Bond and Carr, 1983); moreover, the deuterium abundance could also be explained (Audouze and Silk, 1983).

Ostriker and Cowie (1981) and Ikeuchi (1981) suggest that explosions of supermassive stars could provide an amplification mechanism for generating galaxies and groups of galaxies (see also Doroshkevich, Zel'dovich and Novikov, 1967). In Ostriker and Cowie's model, the explosion of a preexisting "seed" (a supermassive star or a cluster of supermassive stars) with an energy release of ~ 10^-5 mc² leads to a blast wave which sweeps up a shell of surrounding gas. If the explosion occurs at z 10, the shell can radiatively cool and fragment, leading to new objects ~ 10³ times more massive than the original seeds. The new objects may themselves explode, leading to the collapse of still more massive shells, etc. Thus, if we start with small seeds it may be possible to generate all structure up to the characteristic mass-scale that can cool within a Hubble time. Whilst it may be possible to cool on mass-scales corresponding to galaxies or groups of galaxies (cf. Eqs. 8.4) it is difficult to see how a cluster of galaxies such as Coma (~ 10¹⁵ M) could radiate its binding energy and, therefore, why rich clusters should be bound at all (Hogan, 1983). Ostriker and Cowie argue that explosions which occur between 150 z 10 (when Compton drag is ineffective and Compton scattering of electrons by the background radiation provides the dominant cooling mechanism) are likely to lead to the formation of supermassive stars. The explosive model for galaxy formation raises the interesting possibility that perhaps we can account for the origin of galaxies and large-scale structure using astrophysical processes at relatively late epochs without the need to invoke a primordial spectrum of density fluctuations. This idea has been explored further by Hogan (1983) and by Hogan and Kaiser (1983). In Hogan's model, pregalactic radiation sources are assumed to form at redshifts ~ 10² - 10³. The inhomogeneous luminosity of the sources produces gradients in the radiation pressure which in turn generate density fluctuations in the gas. Hogan makes the interesting point that the radiation Jeans mass

represents the largest scale on which radiation pressure could be important in generating density fluctuations, an idea previously exploited by McClelland and Silk (1977). This is interestingly, close to the masses of the largest non-linear structures observed today. Detailed computations of the density fluctuation spectra and the microwave background anisotropies expected on this model are presented by Hogan and Kaiser (1983).