Observations of Galaxy Structure and Dynamics

4.2.4. Central Velocity Dispersions.
III. Correlation With Line Strength

The parameter correlations discussed so far suggest that the properties of elliptical galaxies are largely controlled by one parameter, the total luminosity L (e.g., Faber 1973b). Core parameters correlate closely with L; these include central velocity dispersion, core radius, central surface brightness and metallicity ⁽⁶⁾ Also correlating with L, although with larger scatter, are global parameters such as the effective radius and surface brightness (section 3.3.3) and a measure (V/ sigma )^* of the dynamical importance of rotation versus random motions (section 4.2.6). However, a closer examination of the log sigma - M_B and Mg₂ - M_B correlations suggests that a second parameter is at work. This result (Terlevich et al. 1981) is summarized in Figure 34.

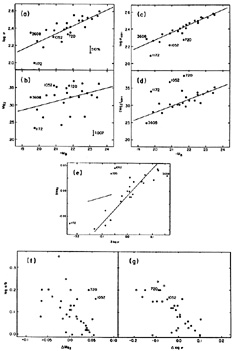Figure 34. Parameter plots from Terlevich et al. (1981) illustrating the probable two-parameter nature of elliptical galaxies. Panels (a) - (d) show correlations with absolute magnitude M_B of the logarithm of the velocity dispersion, log sigma , and the metallicity index Mg₂. The measured parameters are shown in (a) and (b). The scatter is large compared with the estimated error bars, and is therefore real. Panel (e) shows that the deviations delta log sigma and delta Mg₂ from the least-squares lines shown in (a), (b) correlate with each other. Terlevich and collaborators argue that the correlation does not result from subtle measuring errors. In particular, errors in M_B are likely to be too small to have an effect, and would produce the wrong slope. The arrow in (e) shows the result of a 1 mag error in M_B. The equation of the correlation is delta log sigma = 1.852( delta Mg₂) + 0.015 (straight line). The fact that the deviations correlate suggests that a single second parameter is responsible. The effect of this parameter can be removed by using the delta log sigma - delta Mg₂ relation to reduce (say) the observed log sigma - M_B correlation (a) to the mean Mg₂ - M_B relation. The result is panel (c), which shows log sigma _corr = log sigma - 1.852( delta Mg₂) - 0.015 versus M_B. The Mg₂ - M_B correlation is similarly reduced to the mean log sigma - M_B relation in panel (d). In both cases the scatter is greatly reduced and consistent with measuring errors. The straight lines in (c), (d) are new least-squares fits (equations 24, 25), with the four labeled galaxies omitted.

The correlation of Mg₂ and log implies that the galaxies occupy a canted surface in log - Mg₂ - M_B space. A combined least-squares fit yields

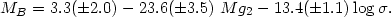(23)

The individual relations corrected for the second parameter (Fig. 34c,d) are:

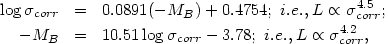(24)

using M_B and log sigma _corr as independent variables, respectively;

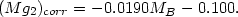(25)

Figure 34 suggests that the second parameter may be related to the intrinsic axial ratio. On average, apparently rounder galaxies have higher sigma and stronger metal lines than flatter galaxies (cf. van den Bergh's 1979 result that rounder galaxies are redder). Note that projection effects should fill in the region below the correlations in Figure 34 (f) and (g) with intrinsically flattened galaxies that are seen nearly face-on.

The above results have been criticized by Tonry and Davis (1981b), who do not confirm the correlations with axial ratio. They suggest that log sigma and Mg₂ do not separately correlate with M_B. Instead, they believe that the delta log sigma - delta Mg₂ relation is driven by a correlation between log sigma and Mg₂. Such a correlation does exist. When plotted using parameters from Terlevich et al. (1981) the scatter is even consistent with the measuring errors, a result which supports Tonry and Davis' suggestion. In fact, the scatter is produced in part by the dispersion in M_B (the "second parameter" in this plot). Thus it may be that the true second parameter in ellipticals is related to Mg₂ or log sigma as well as being related to flattening. However, flattening does seem to be involved in the second parameter (Tonry and Davis apparently did not find any correlations with flattening because their measuring errors were too large, Davies 1982). The above discussion is consistent with Terlevich et al. (1981), who state their conclusions cautiously.

The correlations of Figure 34 contain information about the true shapes of ellipticals, although they are difficult to interpret because intrinsic correlations with flattening as well as the viewing geometry may be involved. The parameter relations also have implications for theories of galaxy formation, being generally easier to reconcile with dissipational-collapse theories than with dissipationless formation. Our understanding of these implications is very rudimentary. Besides enlarging the galaxy sample, we need to explore correlations with parameters which measure more global dynamical quantities.

Panels (f) and (g) suggest that the second parameter may be related to intrinsic axial ratio. They show correlations between the logarithm of the observed axial ratio a/b and deviations Delta Mg₂ and Delta log sigma from the corrected relations (c), (d). Only galaxies brighter than M_B appeq - 20 are illustrated; fainter galaxies tend to be more heterogeneous in their stellar populations (Faber 1977) and show weaker correlations with log(a/b).

⁶ The metallicity is measured using an index Mg₂ of the strength of Mg I "b" plus MgH at ~ 5178Å, see Faber, Burstein and Dressler (1977). The calibration of Mg₂ (in mag) is [Fe/H] = 3.9 Mg₂ - 0.9, where [Fe/H] is the logarithmic metal, abundance relative to the sun (Burstein 1979a; Terlevich et al. 1981). Further discussion of Mg₂ is found in references given in Terlevich et al. (1981). The Mg₂ - M_B correlation is illustrated in Fig. 1 of Faber (1977). Back.