5.7.4. Accretion-driven star formation

What is the duration of these cooling flows in clusters? Both optical and X-ray clusters have been observed at moderately high redshifts, and the gas distributions in clusters are relaxed and smooth; both suggest that the intracluster medium has been present in clusters for a significant fraction of the Hubble time. As described above, the cooling times at the centers of these flows are less than the Hubble time, which suggests that the flows have persisted for a significant fraction of the cluster lifetime. Thus the total mass of gas that has cooled and been accreted by the central galaxy in the cluster should be

(5.111)

This assumes that the accreted mass is not ejected from the cluster center.

Although this is a small fraction of the total gas mass in a cluster ( 10¹⁴ M), it is comparable to the mass in luminous material in a very large (cD) galaxy. It is important to understand where this gas goes after it cools. Many possibilities can be ruled out. First, the gas could remain gaseous but cooler. However, the observations of the X-ray and optical line emission give upper limits on the total amount of ionized gas well below the total accreted mass. Observations of the 21 cm hydrogen line from central galaxies in clusters with cooling flows (Section 3.7) give upper limits on the total amount of neutral atomic hydrogen, typically M_HI 10⁹ M (Burns et al., 1981a; Valentijn and Giovanelli, 1982). Because of the instrumental sensitivity, current observations do not rule out the possibility that the accreted gas could be molecular hydrogen, although it seems likely that the large amounts of molecular gas required would lead to an efficient conversion into stars. As mentioned above, many of the accreting galaxies are radio sources (Burns et al., 1981b), and this may indicate that some of the accreted gas flows into the galactic nucleus and is used to power the central engine of the radio source. However, these sources require only 10^-2 M / yr of gas to provide their observed radio power (Burns et al., 1981b), so it is likely that the fraction of the gas accreted by the central engine is small. Moreover, there could not be as much as 10¹² M in the nucleus of these galaxies, given their observed stellar velocity dispersions (Sarazin and O'Connell, 1983).

Generally, conversion of gas into stars is quite efficient within galaxies, and this conversion could provide both a stable reservoir for the accreted gas and a partial explanation for the existence of central dominant galaxies. Burns et al. (1981a) argued that star formation cannot be occurring at a high rate in these galaxies, because they would then contain more neutral hydrogen than is observed (see above). However, Fabian et al. (1982b) and Sarazin and O'Connell (1983) showed that the cooling times for neutral hydrogen were short enough that all the accreted gas could be cooling through this temperature range and forming stars. Within the disk of our own galaxy, stars are formed with a very wide range of masses extending up to 100 M (Salpeter, 1955). Stars more massive than 10 M produce Type II supernovae when they die, and the rate of these supernovae would probably heat the accreted gas sufficiently to prevent the formation of cooling flows (Wirth et al., 1983). Moreover, if the spectrum of stellar masses formed from the cooling gas (the 'initial mass function') were similar to that in our own galaxy, the central galaxies in the accretion flows would be considerably bluer and brighter than they are observed to be (Fabian et al., 1982b, 1984a; Sarazin and O'Connell, 1983). Burns et al. (1981a) gave similar arguments and concluded that star formation cannot be the ultimate reservoir for the cooling gas.

However, there is really no reason why the initial mass function for star formation in these cooling flows should be the same as that in the disk of our galaxy. If the forming stars had low masses 1 M, these stars would not be very different from the stars found in typical elliptical galaxies (Cowie and Binney, 1977). Since star formation is very poorly understood and there is no successful quantitative theory for this process, one cannot calculate the initial mass function directly. However, Fabian et al. (1982b) and Sarazin and O'Connell (1983) have given a simple plausibility argument as to why the initial mass function for star formation in cooling flows might be limited to low mass stars; a similar argument for elliptical galaxies in general was given by Jura (1977). It is assumed that stars form eventually from the thermally unstable clouds of gas that are seen as optical filaments. Star formation is assumed to start when these clouds become gravitationally unstable and can no longer support themselves against their own gravity and the pressure of the surrounding medium. Clouds become gravitationally unstable when their mass exceeds the Jeans' mass, which for a spherical, static, nonmagnetic isothermal cloud of temperature T immersed in a low density medium of pressure P is given by (Spitzer, 1978)

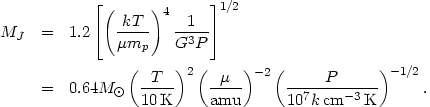
(5.112)

Once a cloud starts to collapse, the pressure within the cloud will increase and the Jeans' mass may be reduced; this can cause the cloud to fragment and result in lower mass stars being formed. It is difficult to produce stars more massive than M_J, however, because before a suitably massive cloud could be assembled, it would become unstable and collapse. Thus it is possible that the Jeans' mass may provide an upper limit on the mass of the largest stars that form. In the disk of our galaxy, the interstellar medium typically has a pressure of P 2 × 10³k cm^-3 K, and equation (5.112) gives M_J 50 M. In the cooling flows in clusters, the pressures derived from models for the X-ray emission or determined directly from the optical line emitting filaments are 10^3-4 times larger (P 10^6-7 k cm^-3 K), and thus the Jeans' mass is M_J 1M. Thus it is possible that only low mass stars are formed from the cooling gas in clusters. (A similar argument was given earlier for low mass star formation in normal elliptical galaxies by Jura (1977).) In Fabian et al. (1982) and Sarazin and O'Connell (1983), this conclusion is shown to be unaffected by the temperature dependence in equation (5.112).

Fabian et al. (1982b) also point out that star formation in cooling flows may be different than in the disk of our galaxy because the star forming regions in these flows are unlikely to contain dust grains. In star forming regions in our galaxy, most of the refractory heavy elements are in the form of solid dust grains, and these grains absorb starlight, emit infrared radiation, and act as a heat source for the gas. Dust grains are destroyed in high temperature gas. Since the gas in cooling flows is initially very hot, any grains would have evaporated (Cowie and Binney, 1977; Fabian et al., 1982b), and it is very difficult for grains to form in low density gas, even if it is cool. Thus it is unlikely that grains will be present in the cooling flows, even in the coolest, star forming clouds. The lack of grains probably lowers the gas temperature, which also tends to reduce M_J. Further, any attempt to estimate the star forming rate in these galaxies from the infrared emission of dust (Wirth et al., 1983) is likely to greatly underestimate the real rate.

Sarazin and O'Connell (1983) have calculated the expected colors and optical spectra of central galaxies assuming that their stellar populations are a mixture of a normal giant elliptical population with a continuously forming population due to accretion-driven star formation. A variety of values for the upper mass limit and the shape of the initial mass function were used. They found that the accreting galaxies should have spectra and colors measurably different from those of nonaccreting giant ellipticals (color differences of typically (U - V) -0.3 mag). They also found that with accretion rates of typically 100 M / yr, the entire stellar population of the central galaxies in many clusters could be due to accretion-driven star formation.

Valentijn (1983) has attempted to measure the colors of the stellar populations in 7 cD galaxies and their spatial variations by surface photometry in two colors (B and V). He finds very large color gradients within these galaxies of typically (B - V) 0.4 mag, with the galaxy centers being extremely red. Valentijn argues that these gradients are the result of accretion-driven star formation, and that as the pressure increases inwards in the cooling flow, the Jeans' mass is lowered (equation 5.112) and the stellar population becomes redder. One problem is that the innermost regions of these galaxies are so red that the required stellar population would have a very small mass-to-light ratio and could not provide enough light (for the observed accretion rates) to account for the observed galaxy luminosity. The color gradients observed by Valentijn are very large (larger than Sarazin and O'Connell predicted), and it is very important that they be confirmed by further observations. Valentijn's photometry appears to disagree, in some cases, with that of other observers (Hoessel, 1980; Malumuth and Kirshner, 1985).

Color gradients might also result from dust extinction, abundance gradients in an old stellar population, or mergers of galaxies having differing colors. A more direct way to detect a stellar population due to accretion is to observe absorption features due to that population in the spectrum of the cD galaxy. The galaxy NGC1275 in the Perseus cluster (Figure 20; Section 4.5.2) is particularly interesting in this regard. Its stellar surface brightness distribution is similar to that of a typical giant elliptical galaxy (Oemler, 1976). However, the stellar population is very blue, and has an A-star absorption spectrum (Kent and Sargent, 1979), whereas typical giant elliptical galaxies are dominated by K stars. Sarazin and O'Connell (1983) show that the colors and spectrum of this galaxy can be understood if the luminous portion of the galaxy is entirely due to accretion-driven star formation at a rate of 300 / yr as given by the X-ray observations, and the upper mass cutoff of the stars formed is 2.8 M. (By contrast, Wirth et al. (1983) present a model for NGC1275 in which the initial mass function for star formation is similar to that in our galaxy, and very massive O stars are formed. However, in this model the star formation rate is more than an order of magnitude less than the observed rate of accretion onto NGC1275.)

One concern with NGC1275 is the presence of the foreground spiral galaxy. Hu et al. (1983) have suggested that this galaxy is colliding with the cooling flow and that this collision powers the optical line emission. It is also possible that this collision might affect the rate and initial mass function of star formation in the galaxy. It is thus very important to observe the spectra of other accreting central dominant galaxies, and see if a younger stellar population can be detected in them. Recently, O'Connell et al. (1987) obtained spectra for the inner regions of a number of accreting galaxies. The cD in A1795, which has a very large accretion rate 400 M / yr (Table 4), has an F-star stellar spectrum, consistent with the entire galaxy being the result of accretion-driven star formation with an upper mass cutoff of about 1.5 M.

Central dominant cluster galaxies appear, in many cases, to have a very large number of globular star clusters (spherical clusters of 10^5-6 stars) associated with them (Harris et al., 1983b). Fabian et al. (1984b) have suggested that these globular clusters might be produced by accretion-driven star formation. They note that the mass of a globular cluster is similar to the Jeans' mass of gas in the cooling flows at a temperature of 10⁴ K, the temperature at which thermally unstable clouds are repressurized. However, Fall and Rees (1985) showed that the cooling time was much shorter than the free-fall time for Jeans' unstable clumps at this temperature, preventing the gravitational instability of the gas. The cooling time depends on the abundance of heavy elements, which may have been considerably lower when galaxies first formed (Section 5.10). Thus Fall and Rees argue that globular clusters formed out of cooling flows during the formation of galaxies.