C. Inflation

1. The scenario

The deep issue inflation addresses is the origin of the large-scale homogeneity of the observable universe. In a relativistic model with positive pressure we can see distant galaxies that have not been in causal contact with each other since the singular start of expansion (Sec. II.C, Eq. [26]); they are said to be outside each other's particle horizon. Why do apparently causally unconnected parts of space look so similar? ²⁶ Sato (1981a, 1981b), Kazanas (1980), and Guth (1981) make the key point: if the early universe were dominated by the energy density of a relatively flat real scalar field (inflaton) potential V() that acts like , the particle horizon could spread beyond the universe we can see. This would allow for the possibility that microphysics during inflation could smooth inhomogeneities sufficiently to provide an explanation of the observed large-scale homogeneity. (We are unaware of a definitive demonstration of this idea, however.)

In the inflation scenario the field rolls down its potential until eventually V() steepens enough to terminate inflation. Energy in the scalar field is supposed to decay to matter and radiation, heralding the usual Big Bang expansion of the universe. With the modifications of Guth's (1981) scenario by Linde (1982) and Albrecht and Steinhardt (1982), the community quickly accepted this promising and elegant way to understand the origin of our homogeneous expanding universe. ²⁷

In Guth's (1981) picture the inflaton kinetic energy density is subdominant during inflation, 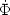² << V(), so from Eqs. (30) the pressure p is very close to the negative of the mass density , and the expansion of the universe approximates the de Sitter solution, a exp(Ht) (Eq. [27]).

For our comments on the spectrum of mass density fluctuations produced by inflation and the properties of solutions of the dark energy models in Sec. III.E we will find it useful to have another scalar field model. Lucchin and Matarrese (1985a, 1985b) consider the potential

(38)

where q and A are parameters. ²⁸ They show that the scale factor and the homogeneous part of the scalar field evolve in time as

(39)

where N = 2q( A)^1/2 / [G(6 - q)]^1/2. If q < 2 this model inflates. Halliwell (1987) and Ratra and Peebles (1988) show that the solution (39) of the homogeneous equation of motion has the attractor property ²⁹ mentioned in connection with Eq. (31). This exponential potential is of historical interest: it provided the first clear illustration of an attractor solution. We return to this point in Sec. III.E.

A signal achievement of inflation is that it offers a theory for the origin of the departures from homogeneity. Inflation tremendously stretches length scales, so cosmologically significant lengths now correspond to extremely short lengths during inflation. On these tiny length scales quantum mechanics governs: the wavelenghts of zero-point field fluctuations generated during inflation are stretched by the inflationary expansion, ³⁰ and these fluctuations are converted to classical density fluctuations in the late time universe. ³¹

The power spectrum of the fluctuations depends on the model for inflation. If the expansion rate during inflation is close to exponential (Eq. [27]), the zero-point fluctuations are frozen into primeval mass density fluctuations with power spectrum

(40)

Here (k, t) is the Fourier transform at wavenumber k of the mass density contrast (, t) = (, t) / <(t)> - 1, where is the mass density and <> the mean value. After inflation, but at very large redshifts, the spectrum in this model is P(k) k on all interesting length scales. This means the curvature fluctuations produced by the mass fluctuations diverge only as log k. The form P(k) k thus need not be cut off anywhere near observationally interesting lengths, and in this sense it is scale-invariant. ³² The transfer function T(k) accounts for the effects of radiation pressure and the dynamics of nonrelativistic matter on the evolution of (k, t), computed in linear perturbation theory, at redshifts z 10⁴. The constant A is determined by details of the chosen inflation model we need not get into.

The exponential potential model in Eq. (38) produces the power spectrum ³³

(41)

When n 1(q 0) the power spectrum is said to be tilted. This offers a parameter n to be adjusted to fit the observations of large-scale structure, though as we will discuss the simple scale-invariant case n = 1 is close to the best fit to the observations.

The mass fluctuations in these inflation models are said to be adiabatic, because they are what you get by adiabatically compressing or decompressing parts of an exactly homogeneous universe. This means the initial conditions for the mass distribution are described by one function of position, (, t). This function is a realization of a spatially stationary random Gaussian process, because it is frozen out of almost free quantum field fluctuations. Thus the single function of position is statistically prescribed by its power spectrum, as in Eqs. (40) and (41). More complicated models for inflation produce density fluctuations that are not Gaussian, or do not have simple power law spectra, or have parts that break adiabaticity, as gravitational waves (Rubakov, Sazhin, and Veryaskin, 1982) or magnetic fields (Turner and Widrow, 1988; Ratra, 1992b) or new hypothetical fields. All these extra features may be invoked to fit the observations, if needed. It may be significant that none seem to be needed to fit the main cosmological structure constraints we have now.

2. Inflation in a low density universe

We do need an adjustment from the simplest case -- an Einstein-de Sitter cosmology -- to account for the measurements of the mean mass density. In the two models that lead to Eqs. (40) and (41) the enormous expansion factor during inflation suppresses the curvature of space sections, making _K0 negligibly small. If = 0, this fits the Einstein-de Sitter model (Eq. [35]), which in the absence of data clearly is the elegant choice. But the high mass density in this model was already seriously challenged by the data available in 1983, on the low streaming flow of the nearby galaxies toward the nearest known large mass concentration, in the Virgo cluster of galaxies, and the small relative velocities of galaxies outside the rich clusters of galaxies. ³⁴ A striking and long familiar example of the latter is that the galaxies immediately outside the Local Group of galaxies, at distances of a few megaparsecs, are moving away from us in a good approximation to Hubble's homogeneous flow, despite the very clumpy distribution of galaxies on this scale. ³⁵ The options (within general relativity) are that the mass density is low, so its clumpy distribution has little gravitational effect, or the mass density is high and the mass is more smoothly distributed than the galaxies. We comment on the first option here, and the second in connection with the cold dark matter model for structure formation in Sec. III.D.

Under the first option we have two choices: introduce a cosmological constant, or space curvature, or maybe even both. In the conventional inflation picture space curvature is unacceptable, but there is another line of thought that leads to a universe with open space sections. Gott's (1982) scenario commences with a large energy density in an inflaton at the top of its potential. This behaves as Einstein's cosmological constant and produces a near de Sitter universe expanding as a exp(H t), with sufficient inflation to allow for a microphysical explanation of the large-scale homogeneity of the observed universe. As the inflaton gradually rolls down the potential it reaches a point where there is a small bump in the potential. The inflaton tunnels through this bump by nucleating a bubble. Symmetry forces the interior of the bubble to have open spatial sections (Coleman and De Luccia, 1980), and the continuing presence of a non-zero V() inside the bubble acts like , resulting in an open inflating universe. The potential is supposed to steepen, bringing the second limited epoch of inflation to an end before space curvature has been completely redshifted away. The region inside the open bubble at the end of inflation is a radiation-dominated Friedmann-Lemaître open model, with 0 < _K0 < 1 (Eq. [16]). This can fit the dynamical evidence for low _M0 with = 0. ³⁶

The decision on which scenario, spatially-flat or open, is elegant, if either, depends ultimately on which Nature has chosen, if either. ³⁷ But it is natural to make judgments in advance of the evidence. Since the early 1980s there have been occasional explorations of the open case, but the community generally has favored the flat case, _K0 = 0, without or, more recently, with a cosmological constant, and indeed the evidence now is that space sections are close to flat. The earlier preference for the Einstein-de Sitter case with _K0 = 0 and ₀ = 0 led to considerable interest in the picture of biased galaxy formation in the cold dark matter model, as we now describe.

²⁶ Early discussions of this question are reviewed by Rindler (1956); more recent examples are Misner (1969), Dicke and Peebles (1979), and Zee (1980). Back.

²⁷ Aspects of the present state of the subject are reviewed by Guth (1997), Brandenberger (2001), and Lazarides (2002). Back.

²⁸ Similar exponential potentials appear in some higher-dimensional Kaluza-Klein models. For an early discussion see Shafi and Wetterich (1985). Back.

²⁹ Ratra (1989, 1992a) shows that spatial inhomogeneities do not destroy this property, that is, for q < 2 the spatially inhomogeneous scalar field perturbation has no growing mode. Back.

³⁰ The strong curvature of spacetime during inflation makes the vacuum state quite different from that of Minkowski spacetime (Ratra 1985). This is somewhat analogous to how the Casimir metal plates modify the usual Minkowski spacetime vacuum state. Back.

³¹ For the development of these ideas see Hawking (1982), Starobinsky (1982), Guth and Pi (1982), Bardeen, Steinhardt, and Turner (1983), and Fischler, Ratra, and Susskind (1985). Back.

³² The virtues of a spectrum that is scale-invariant in this sense were noted before inflation, by Harrison (1970), Peebles and Yu (1970), and Zel'dovich (1972). Back.

³³ This is discussed by Abbott and Wise (1984), Lucchin and Matarrese (1985a, 1985b), and Ratra (1989, 1992a). Back.

³⁴ This is discussed in Davis and Peebles (1983a, 1983b) and Peebles (1986). Relative velocities of galaxies in rich clusters are large, but the masses in clusters are known to add up to a modest mean mass density. Thus most of the Einstein-de Sitter mass would have to be outside the dense parts of the clusters, where the relative velocities are small. Back.

³⁵ The situation a half century ago is illustrated by the compilation of galaxy redshifts in Humason, Mayall, and Sandage (1956). In this sample of 806 galaxies, 14 have negative redshifts (after correction for the rotation of the Milky Way galaxy and for the motion of the Milky Way toward the other large galaxy in the Local Group, the Andromeda Nebula), indicating motion toward us. Nine are members of the Local Group, at distances 1 Mpc. Four are in the direction of the Virgo cluster, at redshift ~ 1200 km s^-1 and distance ~ 20 Mpc. Subsequent measurements indicate two of these four really have negative redshifts, and plausibly are members of the Virgo cluster on the tail of the distribution of peculiar velocities of the cluster members. (Astronomers use the term peculiar velocity to denote the deviation from the uniform Hubble expansion velocity.) The last of the 14, NGC 3077, is in the M 81 group of galaxies at 3 Mpc distance. It is now known to have a small positive redshift. Back.

³⁶ Gott's scenario is resurrected by Ratra and Peebles (1994, 1995). See Bucher and Turok (1995), Yamamoto, Sasaki, and Tanaka (1995), and Gott (1997), for further discussions of this model. In this case spatial curvature provides a second cosmologically-relevant length scale (in addition to that set by the Hubble radius H^-1), so there is no natural preference for a power law power spectrum (Ratra, 1994; Ratra and Peebles, 1995). Back.

³⁷ At present, high energy physics considerations do not provide a compelling specific inflation model, but there are strong indications that inflation happens in a broad range of models, so it might not be unreasonable to think that future advances in high energy physics could give us a compelling and observationally successful model of inflation, that will determine whether it is flat or open. Back.