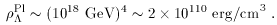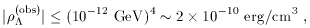1.3 Vacuum energy
The cosmological constant
 is a dimensionful
parameter with units of (length)-2. From the point
of view of classical general relativity, there is no
preferred choice for what the length scale defined by
is a dimensionful
parameter with units of (length)-2. From the point
of view of classical general relativity, there is no
preferred choice for what the length scale defined by
 might be. Particle physics,
however, brings
a different perspective to the question. The cosmological
constant turns out to be a measure of the energy density
of the vacuum - the state of lowest energy - and although
we cannot calculate the vacuum energy with any
confidence, this identification allows us to consider the
scales of various contributions to the cosmological
constant [14,
15].
might be. Particle physics,
however, brings
a different perspective to the question. The cosmological
constant turns out to be a measure of the energy density
of the vacuum - the state of lowest energy - and although
we cannot calculate the vacuum energy with any
confidence, this identification allows us to consider the
scales of various contributions to the cosmological
constant [14,
15].
Consider a single scalar field
 , with potential
energy V(
, with potential
energy V( ). The action can
be written
). The action can
be written
(where g is the determinant of the metric tensor
gµ
In this theory, the configuration with the lowest energy
density (if it exists) will be one in which there is no
contribution from kinetic or gradient energy, implying
ðµ
with
The effect of an
energy-momentum tensor of the form (12) is
equivalent to that of a cosmological constant, as can be
seen by moving the
This equivalence is the origin of the identification of
the cosmological constant with the energy of the vacuum.
In what follows, I will use the terms ``vacuum energy" and
``cosmological constant" essentially interchangeably.
It is not necessary to introduce scalar fields
to obtain a nonzero vacuum energy. The action for general
relativity in the presence of a ``bare'' cosmological
constant
where R is the Ricci scalar. Extremizing this action
(augmented by suitable matter terms)
leads to the equations (7). Thus, the cosmological
constant can be thought of as simply a constant term in
the Lagrange density of the theory. Indeed, (15)
is the most general covariant action we can construct out of
the metric and its first and second derivatives, and is
therefore a natural starting point for a theory of gravity.
Classically, then, the effective cosmological constant is
the sum of a bare term
A precisely analogous situation holds in field theory.
A (free) quantum field can be thought of as a collection of an
infinite number of harmonic oscillators in momentum space.
Formally, the zero-point energy of such an infinite collection
will be infinite. (See
[10,
3] for further
details.) If, however, we discard the very high-momentum
modes on the grounds that we trust our theory only up to
a certain ultraviolet momentum cutoff kmax, we
find that the resulting energy density is of the form
This answer could have been guessed by dimensional analysis;
the numerical constants which have been neglected will depend
on the precise theory under consideration.
Again, in the absence of gravity this energy has no effect,
and is traditionally discarded (by a process known as
``normal-ordering''). However, gravity does exist, and
the actual value of the vacuum energy has important
consequences. (And the vacuum fluctuations themselves are
very real, as evidenced by the Casimir effect
[16].)
The net cosmological constant, from this point of view, is the
sum of a number of apparently disparate contributions,
including potential energies from scalar fields and zero-point
fluctuations of each field theory degree of freedom, as well
as a bare cosmological constant
Similar contributions can arise even without invoking
``fundamental" scalar fields. In the strong interactions,
chiral symmetry is believed to be broken by a nonzero
expectation value of the quark bilinear
These contributions are joined by those from any number
of unknown phase transitions in the early universe,
such as a possible contribution from grand unification
of order MGUT ~ 1016 GeV.
In the case of vacuum fluctuations, we should choose our
cutoff at the energy past which we no longer trust our
field theory. If we are confident that we can use ordinary
quantum field theory all the way up to the Planck scale
MPl = (8
Field theory may fail earlier, although quantum gravity
is the only reason we have to believe it will fail at
any specific scale.
As we will discuss later, cosmological observations imply
much smaller than any of the individual effects listed
above. The ratio of (19) to (20)
is the origin of the famous discrepancy of 120 orders
of magnitude between the theoretical and observational
values of the cosmological constant.
There is no obstacle to imagining that all of
the large and apparently unrelated contributions listed add
together, with different signs, to produce a net
cosmological constant consistent with the limit (20),
other than the fact that it seems ridiculous. We know
of no special symmetry which could enforce a vanishing
vacuum energy while remaining consistent with the
known laws of physics; this conundrum is the ``cosmological
constant problem''.
In section 4
we will discuss a number of issues related to this
puzzle, which at this point remains one of the most
significant unsolved problems in fundamental physics.
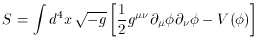
 ), and the
corresponding energy-momentum tensor is
), and the
corresponding energy-momentum tensor is
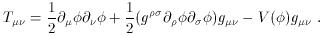
 = 0, for which
Tµ
= 0, for which
Tµ =
- V(
=
- V( 0)
gµ
0)
gµ ,
where
,
where  0
is the value of
0
is the value of  which minimizes
V(
which minimizes
V( ). There
is no reason in principle why
V(
). There
is no reason in principle why
V( 0) should vanish.
The vacuum energy-momentum tensor can thus be written
0) should vanish.
The vacuum energy-momentum tensor can thus be written
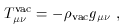
 vac
in this example given by
V(
vac
in this example given by
V( 0).
(This form for the vacuum energy-momentum tensor can also be
argued for on the more general grounds that it is the only
Lorentz-invariant form for
Tvacµ
0).
(This form for the vacuum energy-momentum tensor can also be
argued for on the more general grounds that it is the only
Lorentz-invariant form for
Tvacµ .)
The vacuum can therefore be thought of as a perfect fluid
as in (4), with
.)
The vacuum can therefore be thought of as a perfect fluid
as in (4), with

 gµ
gµ term in
(7) to the right-hand side and setting
term in
(7) to the right-hand side and setting
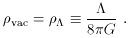
 0 is
0 is
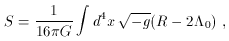
 0 and the
potential energy
V(
0 and the
potential energy
V( ), where the latter may
change with time as the
universe passes through different phases. Quantum
mechanics adds another contribution, from the
zero-point energies associated with vacuum fluctuations.
Consider a simple harmonic oscillator,
i.e. a particle moving in a one-dimensional potential
of the form V(x) = 1/2
), where the latter may
change with time as the
universe passes through different phases. Quantum
mechanics adds another contribution, from the
zero-point energies associated with vacuum fluctuations.
Consider a simple harmonic oscillator,
i.e. a particle moving in a one-dimensional potential
of the form V(x) = 1/2
 2 x2.
Classically, the ``vacuum'' for this system is the state in
which the particle is motionless and at the minimum of the
potential (x = 0), for which the energy in this case vanishes.
Quantum-mechanically, however, the uncertainty principle
forbids us from isolating the particle both in position and
momentum, and we find that the lowest
energy state has an energy E0 = 1/2
2 x2.
Classically, the ``vacuum'' for this system is the state in
which the particle is motionless and at the minimum of the
potential (x = 0), for which the energy in this case vanishes.
Quantum-mechanically, however, the uncertainty principle
forbids us from isolating the particle both in position and
momentum, and we find that the lowest
energy state has an energy E0 = 1/2

 (where I have temporarily re-introduced explicit factors
of
(where I have temporarily re-introduced explicit factors
of  for clarity).
Of course, in the absence of gravity either system actually
has a vacuum energy which is completely arbitrary; we could
add any constant to the potential (including, for example,
-1/2
for clarity).
Of course, in the absence of gravity either system actually
has a vacuum energy which is completely arbitrary; we could
add any constant to the potential (including, for example,
-1/2 
 ) without changing the theory.
It is important, however, that the zero-point energy
depends on the system, in this case on the frequency
) without changing the theory.
It is important, however, that the zero-point energy
depends on the system, in this case on the frequency
 .
.
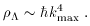
 0. Unlike the last
of these, in the first two cases we can at least make
educated guesses at the magnitudes. In the Weinberg-Salam
electroweak model, the phases of broken and unbroken
symmetry are distinguished by a potential energy
difference of approximately MEW ~ 200 GeV
(where 1 GeV = 1.6 x 10-33 erg); the
universe is in the broken-symmetry phase during our
current low-temperature epoch, and is believed to have
been in the symmetric phase at sufficiently high temperatures
in the early universe. The effective cosmological constant
is therefore different in the two epochs; absent some
form of prearrangement, we would naturally expect a
contribution to the vacuum energy today of order
0. Unlike the last
of these, in the first two cases we can at least make
educated guesses at the magnitudes. In the Weinberg-Salam
electroweak model, the phases of broken and unbroken
symmetry are distinguished by a potential energy
difference of approximately MEW ~ 200 GeV
(where 1 GeV = 1.6 x 10-33 erg); the
universe is in the broken-symmetry phase during our
current low-temperature epoch, and is believed to have
been in the symmetric phase at sufficiently high temperatures
in the early universe. The effective cosmological constant
is therefore different in the two epochs; absent some
form of prearrangement, we would naturally expect a
contribution to the vacuum energy today of order
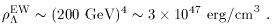
 q (which
is itself a scalar, although constructed from fermions).
In this case the energy difference between the symmetric
and broken phases is of order the QCD scale MQCD
~ 0.3 GeV, and we would expect a corresponding
contribution to the vacuum energy of order
q (which
is itself a scalar, although constructed from fermions).
In this case the energy difference between the symmetric
and broken phases is of order the QCD scale MQCD
~ 0.3 GeV, and we would expect a corresponding
contribution to the vacuum energy of order

 G)-1/2 ~
1018 GeV, we expect a contribution of order
G)-1/2 ~
1018 GeV, we expect a contribution of order