


2.4. The Value of H0 and the Age of the Universe
The immense effort that has been put towards the goal of determining H0, with an accuracy of a few %, has recently come to fruition with different methods providing consistent (within < 10%) values. In Table 1, I present a list of some of the most recent determinations of H0. Note that there are 3 different measurements based on SNIa, giving different values of H0. Although many of the SNIa they use are common, the difference is most probably attributable to the different local calibrations that they employ. Thus, the differences in their derived H0's should reflect the systematic uncertainty introduced by the different local calibrations and it is indeed comparable to the systematic uncertainty that the individual studies have estimated.
| Method | N | H0 | <z> | reference |
| Cepheid | 23 | 75±10 | 0.006 | Freedman et al 2001 |
| 2-ary methods | 77 | 72±8.0 |  0.1 0.1 |
Freedman et al 2001 |
| IR SBF | 16 | 76±6.2 | 0.020 | Jensen et al. 2000 |
| SN Ia | 36 | 73±7.3 |  0.1 0.1 |
Gibson & Brook 2001 |
| SN Ia | 35 | 59±6 |  0.1 0.1 |
Parodi et al 2000 |
| SN Ia | 46 | 64±7 |  0.1 0.1 |
Jua et al 1999 |
| CO-line T-F | 36 | 60±10 | < 0.11 | Tutui et al 2001 |
| S-Z | 7 | 66±15 | < 0.1 | Mason et al 2001 |
| Grav. Lens | 5 | 68±13 | Koopmans & Fassnacht 2000 | |
The method in the last row of Table 1 is based on
gravitational lensing
(cf. [20]).
The basic principle behind this method is that there is a
difference in the light travel time along two distinct rays from a source,
which has been gravitationally lensed by some intervening mass. The
relative time delay
( t), between
two images of the source, can be
measured if the source is variable. Then it can be shown that the Hubble
constant is just:
t), between
two images of the source, can be
measured if the source is variable. Then it can be shown that the Hubble
constant is just:

| (58) |
where 
 is the image separation
and
is the image separation
and
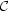 is a constant that
depends on
the lens model. Although, this method has well understood physical
principles, still the details of the lensing model provide quite large
uncertainties in the derived H0.
is a constant that
depends on
the lens model. Although, this method has well understood physical
principles, still the details of the lensing model provide quite large
uncertainties in the derived H0.
A crude n-weighted average of the different H0-determinations in Table 1 gives:

|
where the uncertainty reflects that of the weighted mean (the individual
uncertainties have not been taken into account). However, there seems to
be some clustering around two preferred values (H0
 60 and
60 and
 72 km
s-1 Mpc-1) and thus the above averaging provides
biased results. More
appropriate is to quote the median value and the 95% confidence limits:
72 km
s-1 Mpc-1) and thus the above averaging provides
biased results. More
appropriate is to quote the median value and the 95% confidence limits:

| (59) |
The anisotropic errors reflect the non-Gaussian nature of the distribution of the derived H0-values. Note that the largest part of the individual uncertainties, presented in Table 1, of all methods except the last two, are systematic because they rely on local calibrators (like the distance to the LMC), which then implies that a systematic offset of the local zero-point will "perpetuate" to the secondary indicators although internally they may be self-consistent. A further source of systematic errors is the peculiar velocity model, used to correct the derived distances, which can easily introduce ~ 7% shifts in the derived H0 values [146], [190].
With the value (59), we obtain a Hubble time, tH, equal to:

| (60) |
the uncertainties reflecting the 95% confidence interval.
It is trivial to state that the present age of the Universe t0, should always be larger than the age of any extragalactic object. A well known problem that has troubled cosmologists, is the fact that the predicted age of the Universe, in the classical Einstein de-Sitter Cosmological Model is smaller than the measured age of the oldest globular clusters of the Galaxy. This can be clearly seen from (60) and

| (61) |
and although the latest estimates of the globular cluster ages have been drastically decreased to [84]:

|
One should then add the age of the formation of the globular clusters
and assuming a redshift of formation z
 5 then this age is ~
0.6 -0.8 Gyr's which brings the lower 95% limit of tgc
to ~ 11.6 Gyr's (see however
[30]
for possible formation at z
5 then this age is ~
0.6 -0.8 Gyr's which brings the lower 95% limit of tgc
to ~ 11.6 Gyr's (see however
[30]
for possible formation at z
 10). It is
evident that there is
a discrepancy between t0 and
tgc. This discrepancy could however be
bridged if one is willing to push in the right direction the 95% limit of
both tH and tgc.
10). It is
evident that there is
a discrepancy between t0 and
tgc. This discrepancy could however be
bridged if one is willing to push in the right direction the 95% limit of
both tH and tgc.
However, other lines of research point towards the age-problem. For
example, if at some large redshift we observe galaxies with old stellar
populations, for which we know the necessary time for evolution to
their locally "present"state, then we can deduce again the age
of the Universe. In an EdS we have R
 t2/3 and thus we have:
t2/3 and thus we have:

| (62) |
Galaxies have been found at z
 3 with spectra that
correspond to a
stellar component as old as ~ 1.5 Gyr's, in their local rest-frame. From
(62) we then have that t0
3 with spectra that
correspond to a
stellar component as old as ~ 1.5 Gyr's, in their local rest-frame. From
(62) we then have that t0
 12 Gyr's, in
disagreement with the EdS age (61).
12 Gyr's, in
disagreement with the EdS age (61).
This controversy could be solved in a number of ways, some of which are:
 < 1)
cosmological model,
< 1)
cosmological model,

 > 0.
> 0.
The first possibility is in contradiction with many observational data and
most importantly with the recent CMB experiments (BOOMERANG, MAXIMA and
DASI), which show that
 = 1 (see
[44],
[43],
[90],
[164]
[130]).
= 1 (see
[44],
[43],
[90],
[164]
[130]).
The second possibility [173] can solve the age-problem by assuming that we live in a local underdense region that extends to quite a large distance, which would then imply that the measured local Hubble constant is an overestimate of the global one by a factor:

|
where the bias factor b, is the ratio of the fluctuations in
galaxies and
mass. To reduce the Hubble constant to a comfortable value to solve the
age problem, say from 72 to 50 km sec-1 Mpc-1, one
then needs
 N / N
N / N
 -0.9 / b. Values
of b are highly uncertain and model dependent,
but most recent studies point to b ~ 1 (cf.
[88])
which would then mean
that we need to live in a local very underdense region, something
that is not supported by the linearity of the Hubble relation out
to z
-0.9 / b. Values
of b are highly uncertain and model dependent,
but most recent studies point to b ~ 1 (cf.
[88])
which would then mean
that we need to live in a local very underdense region, something
that is not supported by the linearity of the Hubble relation out
to z  0.03 (cf. [64])
or out to z
0.03 (cf. [64])
or out to z
 0.1 (cf.
[171]).
This is not to
say that we are not possibly located in an underdense region,
but rather that this cannot be the sole cause of the age-problem
[193].
0.1 (cf.
[171]).
This is not to
say that we are not possibly located in an underdense region,
but rather that this cannot be the sole cause of the age-problem
[193].
Thus we are left with the last possibility of a Universe dominated by
vacuum energy (a Universe with

 > 0 - see
section 1.3). If we live in
the accelerated phase (see Fig.1)
we will measure:
> 0 - see
section 1.3). If we live in
the accelerated phase (see Fig.1)
we will measure:

|
ie.,
a larger Hubble constant, and thus smaller Hubble time as we progress in
time, resolving the age-problem. In fact we have strong indications (see
next section), from the SNIa results, which trace the Hubble relation
at very large distances (see section 3.2),
and from the combined
analysis of CMB anisotropy and galaxy clustering measurements
in the 2dF galaxy redshift survey
[54],
for a flat Universe with


 0.7. Then from (23) we obtain the age of the Universe in such a model:
0.7. Then from (23) we obtain the age of the Universe in such a model:

|
Indeed the resolution of the age-problem gives further support to the

 > 0 paradigm.
> 0 paradigm.