


In this section we provide background information intended to help the reader follow and interpret the developments in the field of galaxy alignments. This includes a brief and qualitative overview of key concepts in galaxy formation and evolution processes, an introduction to weak gravitational lensing and associated measurements, as well as a summary of the theory of tidally induced galaxy alignments.
2.1. A primer on galaxy formation and evolution
We give a brief introduction to the current picture of galaxy formation and evolution, with a focus on processes that are thought to be relevant for a galaxy's morphology and alignment with surrounding structures. Detailed accounts of this topic can be found in Benson (2010) as well as Longair (2008) and Mo et al., (2010). Technical introductions to the theory of structure formation are for instance given in Peacock (1999) and Dodelson (2003).
Structure formation takes place on the background of an expanding, homogeneous and isotropic spacetime described by a Friedman-Lemaître-Robertson-Walker (FLRW) metric. The mean total matter density at a given time is determined by the curvature of spacetime as well as the abundances of radiation (matter with relativistic velocities and thus exerting radiation pressure), non-relativistic matter (including ordinary matter of which stars, planets, etc. are composed, and dark matter which makes up a large fraction of cosmological structures and dominates their dynamics), and dark energy. The latter is usually assumed to permeate space smoothly and therefore only affects structure formation via its impact on the background density and the expansion rate. Radiation can easily escape gravitational wells (free-streaming) and thereby suppresses the build-up of structures.
About four to five times more abundant than ordinary matter, it is dark matter that governs the formation of structures via gravitational interaction. The absence of elastic collisions between dark matter particles implies a pressureless and non-viscous fluid. The subdominant ordinary matter, comprising mostly neutrons, protons, and electrons (summarised henceforth under the common but somewhat inaccurate term baryons), closely follows the dark matter after decoupling at recombination on scales above the Jeans length where gravity dominates over pressure in the baryon fluid. The distribution of initially tiny fluctuations around the mean matter density is thought to be provided by the process of inflation in the early Universe, which enlarges quantum fluctuations to macroscopic scales. Inflationary models also predict that these density fluctuations are well described by a Gaussian random field. The fluctuation strength is scale-invariant with a power-law exponent close to one, meaning that there are identical amounts of fluctuation in the density field in each logarithmic wavelength interval.
Once pressureless matter dominates the expansion history, density fluctuations within the horizon (i.e. in regions in causal contact, which can physically interact) grow via gravitational interaction, initially by processes that are well understood in linear perturbation theory. Eventually, over-densities begin to evolve non-linearly and collapse into a halo, an approximately stable state in which the random motions of the constituent particles or objects balance gravity. Structures continue to grow in a bottom-up scenario, i.e. small haloes form first and then coalesce into ever larger haloes. The abundance of dark matter haloes for a given mass can be estimated analytically (Press & Schechter 1974, Bond et al., 1991), while it was found empirically from simulations (Navarro et al., 1997, Gao et al., 2008) that they have a near-universal radial density distribution. Interpreted as quasi-stable bodies with only little exchange of matter with their surrounding, haloes can be assigned an angular momentum vector and a shape, often approximated by an ellipsoid which is determined by the eigenvalues and eigenvectors of the inertia tensor (see Figure 1). These quantities, which are believed to be central to alignment processes, depend (sometimes strongly) on practical implementation choices such as the definition of a halo and the weights assigned to particles in the computation of the inertia tensor (Bett et al., 2007).
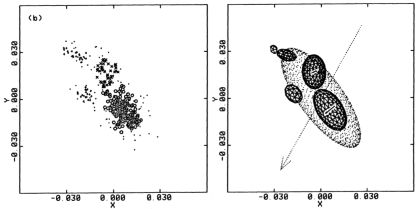 |
Figure 1. Left: Sample of simulation particles subsumed into a common halo in an N-body simulation. The halo was identified by a variant of the friends-of-friends algorithm, identifying arbitrarily shaped regions with a density above a certain threshold. Increasing this threshold, the halo is decomposed into a number of sub-haloes indicated by the different symbols. Right: Representation of the halo and its substructure by ellipsoids which are determined by the eigenvalues and directions of the inertia tensor. The directions of the angular momenta of the larger sub-haloes as well as of the parent halo are given by the arrows. Halo shapes and spins are key ingredients for the study of halo and galaxy alignments. © AAS. Reproduced with permission from Barnes & Efstathiou (1987). |
N-body simulations illustrate in a striking manner how the initially Gaussian density fluctuations evolve under gravity into the cosmic web, a network of overdense filaments which intersect at massive haloes. The filaments are in turn embedded into medium-density walls or sheets which surround large, nearly empty regions of space called voids. The general direction of gravitational acceleration will cause matter to flow away from the centres of voids onto sheets, in the plane of sheets towards filaments, and along filaments into the massive haloes at the nodes (possibly having collapsed into smaller haloes well before). Figure 2 displays part of the cosmic web obtained in a simulation that contained collision-less dark matter particles as well as gas and star particles (Codis et al., 2014).
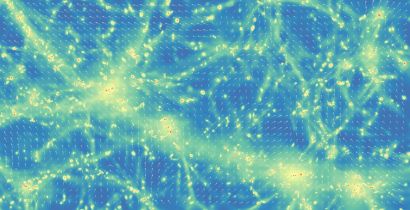 |
Figure 2. Gas density tracing the cosmic web in a subvolume (12.5 Mpc / h comoving horizontally, stacked over 25 Mpc / h comoving along the line of sight) of the HORIZON-AGN simulation. The arrows indicate the direction of the smallest eigenvector of the gravitational tidal tensor (given by the Hessian of the gravitational potential at that point), which is expected to align with filamentary structures on average (see e.g. the top left corner). See Section 5 for more details about the classification of filaments. Reproduced with permission from Codis et al., (2014). |
Baryons follow the dark matter and thus accumulate in the centres of haloes to eventually form galaxies, via processes that are still poorly understood. When baryons fall into haloes, pressure becomes important in the form of accretion shocks, which convert the ordered infall motion into the random velocities of a virialised gas. Further collapse only takes place when the gas can cool, i.e. lose kinetic energy via radiation. If the cooling is efficient, as is expected for low-mass haloes up to Milky Way size (Rees & Ostriker 1977), the baryonic gas can essentially free-fall into the centre of the halo. Otherwise, the hot gas fills the halo from where it gradually descends towards the centre, with the more dense central regions cooling faster.
In any case, the gas reaching the central part of the halo will build up angular momentum, for instance by free-falling with a certain impact parameter from a preferred direction given by the adjacent filaments, or by being subjected to the tidal gravitational field of surrounding structures which exerts a torque on in-falling material. This leads to the generation of a rotationally-supported disc of gas and, eventually, of stars. Note that the vast majority of alignment studies have been conducted in optical passbands in which the brightness distribution of galaxies is determined by the light of the stars. In these scenarios, the orientation of the angular momentum vector and hence the disc is expected to be linked to the configuration of the surrounding large-scale structure (see e.g. Prieto et al., 2014), which could act as a seed for galaxy alignments. However, since only a fraction of the gas in a galaxy is converted into stars, there must exist feedback processes that limit (or even reverse) the gravitational collapse of gas, which in turn will also impact on the angular momentum amplitude and orientation of the stellar distribution.
The complex history of mergers of a dark matter halo with other haloes has a strong impact on the evolution and appearance of the galaxy that it may host. Accreted smaller haloes can survive for a long time orbiting inside the large halo. The satellite galaxies inside these subhaloes may be tidally stripped, may have their orientation tidally locked with respect to the centre of the host halo, and could eventually be tidally disrupted (see e.g. Pereira et al., 2008). A major merger, i.e. the coalescence of two haloes or galaxies with comparable mass, may disrupt the progenitors completely, erasing any memory of alignments generated during galaxy formation, and lead to the formation of a dynamically hot, spheroidal system, such as elliptical galaxies or the central bulges of disc galaxies 1.
New alignments may be formed in major mergers by the re-arrangement of stellar orbits, and thus the light distribution. Elliptical galaxies seem to have similar shapes and orientations as their underlying dark matter haloes, which in turn are well-described by triaxial ellipsoids (though reality can be more complex, with different effective ellipticity or orientation as a function of radius; see, e.g., Schneider et al., 2012, and substantial misalignment between galaxy and halo; see Section 4.1). The characteristics of these ellipsoids are determined in a complex way by the surrounding matter distribution and details of the merger history, such as the provenance of progenitor haloes. These, and possibly other effects, lead to alignments of halo shapes out to separations of tens of megaparsecs (e.g. Hopkins et al., 2005), which are stronger the more massive the haloes.
The more secular processes of galaxy evolution, such as star formation and subsequent chemical enrichment of the interstellar medium (McKee & Ostriker, 2007), or the balance of continued accretion of intergalactic gas versus feedback processes by supernovae (Efstathiou 2000) and active galactic nuclei (Silk & Rees 1998), could also play some role in the evolution of galaxy alignments, for instance via the re-distribution of angular momentum and the modification of the galaxy's spectral energy distribution, which implies that we may study the alignments of different components of a galaxy at different times for a given passband.
All in all, the processes of galaxy formation and evolution are intimately linked at various stages to the creation or destruction of galaxy alignments. We can therefore expect alignment signals to depend on the galaxy's large-scale environment, its morphological type (late for disc galaxies, early for elliptical galaxies), its colour (blue for star-forming disc galaxies, red for ellipticals dominated by old stellar populations), its mass or luminosity, redshift or age, and more. Both galaxy evolution and alignments depend on highly non-linear physics acting over a wide range of spatial and mass scales, and involve dark and baryonic matter, which makes it a challenge to model them accurately.
2.2. A primer on weak gravitational lensing
Correlations induced by the distortions of the images of distant galaxies due to gravitational lensing are a sensitive probe of the large-scale matter distribution as well as the geometry of spacetime. Intrinsic galaxy alignments partly mimic this correlation signal and can thus bias cosmological constraints inferred from galaxy shape correlations if those correlations are presumed to be entirely due to lensing. To facilitate the insight into this link, we sketch the basic methodology and formalism of weak gravitational lensing in this section. Note that galaxy alignment observations share many challenges with weak lensing, in particular the measurement of the ellipticities and orientations of faint galaxy images. A standard technical introduction to weak lensing is given in Bartelmann & Schneider (2001); see also Schneider (2006). Bartelmann (2010) provided an overview on the whole theme of gravitational lensing, while more specialised reviews are presented in Munshi et al., (2008) and Hoekstra & Jain (2008) on cosmological applications of weak lensing, in Massey et al., (2010) on the study of dark matter particularly via weak lensing, and in Kilbinger (2014) on recent progress in weak lensing by the large-scale structure.
The gravitational deflection of light is accurately described in the framework of general relativity and served as the first successful observational test of the validity of Einstein's theory (Dyson et al., 1920). According to Fermat's principle, light follows paths, called geodesics, for which the light travel time is stationary, i.e. the derivative of the light travel time with respect to position is zero. In spatially flat FLRW cosmologies without structures, this results in a straight path for the light ray, while in a spacetime curved by a large mass such as a galaxy cluster, light will generally travel along curved geodesics. For background light sources close to this massive object (the lens), several stationary points in light travel time may exist, corresponding to multiple images of the same object according to Fermats principle. The differential deflection of light from extended sources distorts the images into arcs tangentially around the centre of the lens, and can also magnify them. These sometimes spectacular effects visible on individual objects define the regime of strong gravitational lensing.
At larger distances from the lens no multiple images occur, and distortions and magnification only cause small modifications to the original light profile of the source, usually also a galaxy (see Figure 3). Yet, by averaging in an annulus around the lens over the shapes of source galaxies, one may still be able to recover the net effect induced by gravitational lensing. If statistical tools need to be employed to detect a signal, one refers to weak gravitational lensing effects. The changes to an image are captured to first order in the Jacobian matrix A of the mapping between the source and the image,
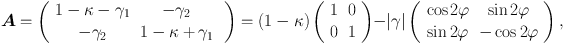 |
(1) |
where κ is the convergence and γ = γ1 + i γ2 the gravitational shear. The second equality provides an illustrative understanding for this mapping. The convergence κ yields isotropic focusing, whereas γ quantifies distortions of the image (and anisotropic focusing). Sources with circular isophotes are mapped into elliptical images, where a combination of κ and |γ| determines the length of the major and minor axes, while the polar angle of γ, denoted by ϕ, describes the orientation of the ellipse. The factor of 2 in the phase takes into account that the shear is a polar (i.e. spin-2) quantity which maps onto itself after a rotation by 180 degrees. The magnification of the image is given by µ = 1 / |detJ|, and both the flux and the size are modified by factors of µ since lensing does not change the surface brightness. To date, magnification effects have not been used as extensively as gravitational shear in studies of galaxies or cosmology because one generally expects lower signal-to-noise than for equivalent shear statistics (for applications see e.g. Scranton et al., 2005 on magnification bias, Ford et al., 2014 on flux magnification, and Huff & Graves 2014 on size magnification). They come in principle with their own intrinsic correlations of galaxy observables, which we will not discuss further here.
Galaxies as light sources are intrinsically non-circular in general, and the deviation from a circular image can to first order be described by an intrinsic ellipticity єs. This ellipticity is intrinsic in the sense that it is a property of the galaxy itself rather than induced by gravitational deflection as the light travels to the observer, after leaving the galaxy. The observed ellipticity under the gravitational lens mapping is then given by Seitz & Schneider (1997)
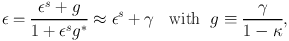 |
(2) |
where g is called the reduced shear. Both ellipticities and shear are understood as complex numbers in this equation (with the complex conjugate denoted by a star), encoding the shape in the absolute value and the orientation with respect to some reference axis in the phase, e.g. є = |є| e 2i ϕ . The simple summation of shear and ellipticity in the second equality of Equation (2) only holds in the limit of very weak lensing effects 2, i.e. |γ|, κ ≪ 1. It is important to note that the term ellipticity is not uniquely defined in general and, even if galaxy images were simple solid ellipses with semi-minor to semi-major axis ratio b / a, could correspond to several quantities which are functions of b / a. The formalism presented in this section applies to the definition |є| = (a − b) / (a + b).
Under the assumption of randomly oriented galaxies, ⟨єs ⟩ = 0 (angular brackets denote ensemble averages), so that in the weak limit of Equation (2) the observed ellipticity is an unbiased estimator of gravitational shear, ⟨є ⟩ = γ. Averaged over large areas of sky, the shear is expected to vanish as well due to the isotropy of the Universe. Hence, to lowest order, one generally considers two-point statistics to detect weak gravitational lensing effects, i.e. correlations between pairs of galaxy shapes or alignments of galaxy shapes with reference positions. Typically, gravitational shear modifies the ellipticity of a galaxy only at the percent level, so that large samples of source galaxies are required to obtain sufficient signal-to-noise. Correlations of gravitational shears measured over large patches of sky yield a signal referred to as cosmic shear which measures the net lensing effect by the intervening large-scale structure.
Specifically, weak lensing shear provides a measurement of the projected tidal gravitational field through its distorting effect on a galaxy shape and combines geometrical information (due to the mapping of spatial derivatives to angular derivatives) with information about structure growth and gravity (relating tidal shear to the density field). In the most basic form, the average weak lensing shear is given by a line-of-sight integration,
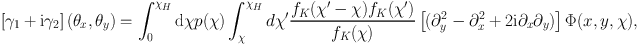 |
(3) |
collecting second derivatives of the gravitational potential Φ at the spatial position (x, y, χ) which are, in the small angle limit, related to the angular position through θx = x / χ and θy = y / χ. Here, χ is the comoving distance, χH ∼ c / H0 is the comoving horizon distance (H0 is the Hubble constant and c is the speed of light), and fK(χ) is the comoving angular diameter distance, given by
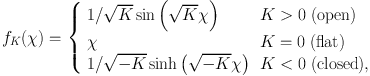 |
(4) |
where 1 / √|K| is interpreted as the curvature radius of the spatial part of spacetime. The line-of-sight average is taken over the probability distribution of comoving distances for the source galaxies, p(χ).
Analytically, the power spectrum, i.e. the Fourier transform of the correlation function, is the most convenient two-point statistic to work with. The angular power spectrum, Cγγ(ℓ), of weak gravitational shear is derived from the line of sight expression in Equation (3) by Limber-projection (Kaiser 1992),
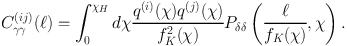 |
(5) |
With increasing χ, ever smaller wavenumbers k = ℓ /
fK(χ) contribute to the fluctuation on the
multipole ℓ, from which one can obtain the angular scale through
π / ℓ. The shear correlations are generated by the
continuous deflection of light by the matter distribution between the
source galaxies and Earth, hence the line-of-sight integration over the
power spectrum Pδ δ of the matter density
contrast δ = ρ /
 − 1, where
− 1, where
 denotes the mean matter density. The projected and three-dimensional
power spectra are formally defined as
denotes the mean matter density. The projected and three-dimensional
power spectra are formally defined as
 |
(6) |
where the tilde denotes Fourier transforms, and δD(n) is the n-dimensional Dirac delta distribution. Angular frequencies and wavenumber in bold denote vectors in two and three dimensions, respectively. The integral in Equation (5) is weighted by the lensing efficiency
 |
(7) |
which is proportional to the ratio of the distance between source and lens over the distance between source and observer, weighted by the line-of-sight distribution of source galaxies, p(i)(χ). Different galaxy samples can be (cross-)correlated, and these are indexed by the superscripts in parentheses. Note that a = 1 / (1 + z) in the equation above refers to the cosmic scale factor, z denotes redshift, and Ωm is the matter density parameter. In practice, lensing is most efficient when the lens is approximately midway between us and the source.
While the shear power spectrum can be obtained from a catalogue of shear estimates directly, most analyses to date are based on its Fourier transforms, the shear correlation functions
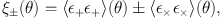 |
(8) |
as they are insensitive to the generally very complex angular selection function of weak-lensing quality photometric survey data. Since the shear is a complex quantity, one can obtain three real-valued correlation functions of which only the two given above contain cosmological information (the third vanishes if parity is conserved). The correlation functions are given in terms of the tangential ellipticity component є+ = − Re (є e2 i ϕ) and the cross component є× = − Im (є e2 i ϕ), where the polar angle ϕ is measured against the line connecting the pair of galaxies 3. The averages in Equation (8) are calculated by summing the corresponding products of ellipticity components over all galaxy pairs in a given angular separation bin centred on θ.
Other statistical measures with desirable properties can be derived from the shear correlation functions, for instance the aperture mass dispersion (Schneider et al., 1998), which to a good approximation separates the field of gravitational shears into a curl-free and a divergence-free part, called E- and B-modes respectively, in analogy to decompositions of polarisation. Gravitational lensing effects only generate a negligible level of B-modes through higher-order effects, so estimates of B-mode shear correlations can therefore be employed as a test for systematic effects in the shape measurement process. Moreover, the source galaxies are often split into redshift slices, which improves cosmological constraints (Hu 1999), particularly on those parameters that encapsulate evolutionary effects (e.g. the dark energy equation of state parameters). To perform this tomography, a large number of redshifts for faint galaxies are required, which is too costly to obtain via spectroscopy. Instead, multi-band photometry, usually in the optical and supplemented by near-ultraviolet and near-infrared passbands if available, is used to obtain very low-resolution information on the spectral energy distribution of a galaxy. The precision of these photometric redshifts is limited to a scatter typically of order 0.05(1 + z). Catastrophic failures can occur e.g. due to the confusion of spectral features like the Balmer and Lyman breaks, leading to potentially large systematic offsets in redshift and hence to groups of outliers in the line-of-sight distribution of source galaxies that enters Equation (7). The estimation of photometric redshifts and the characterisation of their quality via calibration samples or clustering measurements is an active field of research (e.g. Hildebrandt et al., 2010).
Cosmic shear was first detected at the turn of the millennium (Bacon et al., 2000, Kaiser et al., 2000, Van Waerbeke et al., 2000, Wittman et al., 2000) and is developing into an increasingly mature cosmological probe (e.g. Schrabback et al., 2010, Heymans et al., 2013, Simpson et al., 2013, Kitching et al., 2014). The large scatter of intrinsic galaxy ellipticities limits the signal-to-noise of these measurements, introducing a shot noise-like term in the statistical errors, so that the efforts to measure cosmic shear are driven towards faint galaxy samples in deep surveys with high number densities. This in turn renders the estimation of gravitational shear from noisy, small and pixelated galaxy images a challenge, which has spawned large community effort to develop more powerful algorithms (Heymans et al., 2006b, Massey et al., 2007, Bridle et al., 2010, Kitching et al., 2012, Mandelbaum et al., 2014). In addition to shear estimation biases and accurate photometric redshift determination, a further key issue for the forthcoming generation of cosmic shear measurement campaigns are intrinsic galaxy alignments 4 which can mimic the correlations expected from cosmic shear. Using Equation (2) in its weak limit, a generic correlator of two galaxy ellipticities, as is for instance found in Equation (8), reads
 |
(9) |
In the following we will adopt a common shorthand notation for the resulting terms: GG for the shear correlation, which is the desired quantity for cosmological analysis, II for correlations between the intrinsic ellipticities of two galaxies, and GI for correlations between the gravitational shear acting on one galaxy and the intrinsic shape of another galaxy. Note that one of the GI terms in Equation (9) is expected to vanish because the shear acting on a galaxy in the foreground cannot be affected by a galaxy behind the source galaxy, unless their positions along the line of sight are confused because of errors in the redshift measurement. If galaxy shapes are intrinsically randomly oriented, only GG is non-zero. However, since galaxies are known to align with other galaxies (generating II) and with the large-scale structure that in turn contributes to the gravitational deflection of light from background galaxies (generating GI), cosmic shear measurements may be severely biased if these alignment effects are not accurately modelled or removed from the signal. An illustration of the generation of II and GI correlations is provided in Figure 3. Their two-point correlations for the tomographic case can be expressed analogously to Equation (5) as (e.g. Joachimi & Bridle 2010)
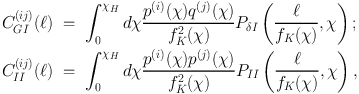 |
(10) |
where p(i)(χ) is the distribution of galaxies in the ith tomographic bin and the resulting lensing efficiency function is q(i)(χ); see Equation (7). The power spectra, Pδ I and PII, quantify the correlation between the matter distribution and the intrinsic shear, γI, and among the intrinsic shears of different galaxies, respectively. The intrinsic shear can be understood as the correlated part of the intrinsic ellipticity of a galaxy, which is not an observable quantity per se. Yet, when considering ensembles of galaxy shapes, it is conceptually useful to split the intrinsic ellipticity into γI, which determines the alignments, and a purely random part, which only leads to a noise contribution in correlations.
The gravitational lensing effect is correlated across tomographic bins because two bins share the common light path through the large-scale structure in front of the less distant bin. In contrast, intrinsic alignments are local processes so they are only correlated within the same tomographic bin, unless the galaxy distributions of different bins overlap due to scatter in the photometric redshifts. The challenge is to obtain a good model for the underlying power spectra, Pδ I and PII. This has prompted an interest in galaxy alignments from the cosmology community in recent years. The new large and high-quality datasets for cosmic shear surveys and the advancements in data analysis techniques, especially the accurate measurement of galaxy shapes in the presence of noise, complex models of the telescopes point-spread function (PSF), and image artefacts, have also greatly improved the power and fidelity of galaxy alignment observations (see Kirk et al., 2015).
Finally, we briefly mention the rich field of weak galaxy lensing (often referred to as galaxy-galaxy lensing), probing the (dark) matter environment around individual galaxies (first detected by Brainerd et al., 1996). Since the lensing signal from a single galaxy is too weak to detect, lens galaxies selected with certain properties (e.g. colour, luminosity, redshift) are stacked. Alternatively, one can carry out this process with individual galaxy clusters or ensembles of galaxy clusters and groups. Stacking is performed statistically by measuring correlation functions of the form ξg+(θ) = ⟨δg є+ ⟩(θ), where δg = Ng / ⟨N ⟩g − 1, denotes the number density contrast of lens galaxies, with Ng the galaxy number count. The tangential ellipticity is measured with respect to the line connecting the background galaxy to the lens. For large separations θ this correlation probes the large-scale matter distribution and how it is traced by the lens galaxies, while on megaparsec scales and below it measures the average tangential matter profile of the lenses. If the same type of statistic is applied to a source galaxy sample that resides at the same redshifts as the lens galaxies, one does not expect any gravitational lensing effects but instead obtains a measure of the alignment of galaxy shapes towards the positions of physically close neighbours. Measurements of this kind will be further discussed in Section 6.
We briefly review the basic concepts of how tidal gravitational fields are thought to generate alignments between the shapes of galaxies or larger bound structures such as galaxy clusters, and the large-scale matter distribution or the shapes of other galaxies. We will distinguish between the tidal processes thought to apply to the two major types of galaxies: angular momentum generation for disc galaxies, and the coherent modification of stellar orbits for elliptical galaxies. For the plethora of observational signatures that will be discussed in this work, most – possibly all – analytic models are based on the assumption of tidally generated alignments. This theory has been quite successful at predicting the general form of correlations between tidal fields and observables, but is bound to fail at making quantitative statements about the amplitude of signals as these depend strongly on highly non-linear and stochastic processes (such as the later stages of gravitational collapse that lead to galaxy formation). For this reason we shall for simplicity limit ourselves to establish proportionalities in most equations. Note that other alignment theories will be discussed in Section 6.
Tidal interaction of galaxies with the surrounding gravitational field can in many cases be understood as a perturbative process, in particular at early stages of galaxy formation. Commonly, the interaction is described by Lagrangian perturbation theory, where the basic quantities are trajectories of dark matter constituent particles. These trajectories are determined by the strength and direction of gravitational fields, and the perturbation series is constructed by considering higher-order derivatives of the gravitational potential that lead successively to more detailed curved trajectories.
At lowest order, however, the Zel'dovich approximation (Zel'dovich, 1970) tells us that all dark matter particles follow straight lines parallel to the gravitational field at their initial positions, or
 |
(11) |
in Cartesian coordinates with α = 1,2,3, where x is the particle's comoving coordinate and ζ its coordinate in the Lagrangian frame. The linear growth factor of structure is given by D(a) and Ψ denotes a displacement potential, which is proportional to the gravitational potential Φ.
The dynamics of objects such as protogalaxies are obtained by integrating over the Lagrangian trajectories of all particles that make up the object. The notion of an actual object allows the definition of a centre of gravity ζ, relative to which the motion of a test particle can be expanded in a Taylor-series,
 |
(12) |
such that one obtains the peculiar motion ∝∂α Ψ(ζ) of the object as a whole and the differential motion of the particles relative to the centre of gravity ∝ ∂α ∂β Ψ(ζ).
Gradients of the gravitational force across the object can lead to a change in the protogalaxy's shape (tidal stretching) as well as generate angular momentum (tidal torquing). The strength of both effects depends on the orientation of the protohalo's inertia tensor, Iαβ, relative to the quadrupole of the gravitational potential, given by the tidal shear tensor Tαβ ∝ ∂α∂β S{Φ}, where S{} is a smoothing operator that removes structures below the scale of the galaxy and keeps only the large-scale contributions (however, the theory does not specify the smoothing scale). For a more rigorous argument on the foundation of tidal alignment theory see the review by Schäfer (2009).
The protogalaxy is expected to contract fastest in the direction of the strongest positive curvature of the gravitational potential, so that an initially spherical mass distribution will evolve into an ellipsoid whose principal axes are collinear with those of the tidal shear tensor. Using a similar argument, but applying it directly in projection onto the sky, Catelan et al., (2001) proposed the following model for the intrinsic shear, γI,
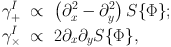 |
(13) |
where x and y are Cartesian coordinates in the plane of the sky, and the shear tangential and cross components are measured with respect to the x-axis. The constant of proportionality absorbs the response of the shape of the visible galaxy to the tidal field, as well as any stochastic misalignments once an ensemble of galaxies is considered. Hirata & Seljak (2004) adopted this model, related the gravitational potential to the matter density contrast δ, and derived a cross-power spectrum between matter density contrast and the intrinsic shear,
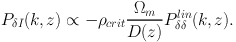 |
(14) |
They assumed that the tidal field at the time of galaxy formation determines the alignment, so that the correlation with the matter field is frozen in since then, whence the growth factor D(z) is divided out 5. The constant ρcrit corresponds to the total matter density today in a spatially flat universe. This result can be used to predict a GI signal via Equation (10), and Hirata & Seljak (2004) also obtained a similar expression for the power spectrum of pairs of intrinsic shears that leads to an II signal,
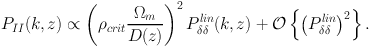 |
(15) |
The same authors also related Equation (14) to a correlation function between matter and tangential intrinsic shear, projected along the line of sight,
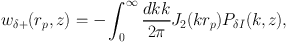 |
(16) |
where J2 is a cylindrical Bessel function of the first kind. Using galaxies as tracers for the distribution of mass, this correlation function is measured in bins of transverse separation rp and line-of-sight separation between pairs of galaxies and then summed over the line of sight to boost signal-to-noise and wash out effects of redshift space distortions. This statistic is more readily determined than the power spectrum from data with complex spatial geometry and selection functions, and is therefore the most widely used observable of large-scale galaxy alignment measurements; see Section 6 and Kirk et al., (2015).
A large body of work has considered alignment of orientation angles rather than full ellipticities. Assuming that the galaxy ellipticity components and the galaxy distribution follow a multivariate Gaussian, Blazek et al., (2011) derived the relation between wδ + and the average of cos(2 θ) over all galaxy pairs where θ is the angle between the major axis of one galaxy and the line connecting the pair (see e.g. Li et al., 2013). It reads
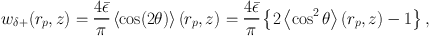 |
(17) |
where  is
the mean absolute value of the ellipticity of
the sample. We added the second equality, which is a useful expression
in a slightly different context, using trigonometric relations. The
average of cos2 θ over all pairs is a popular statistic in
galaxy cluster alignment studies, where in this context θ
corresponds to the angle between the major axis of the satellite
distribution and the line to the other cluster in the pair (e.g.
Smargon et al.,
2012).
All these predictions originate from the ansatz in Equation (13), which
leads to a linear scaling of the intrinsic shear with matter density
contrast and is thus termed the linear alignment model. It is
widely considered appropriate for elliptical galaxies, as well as the
distribution of galaxies within clusters, as these are believed to trace
the shape of the underlying dark matter haloes.
is
the mean absolute value of the ellipticity of
the sample. We added the second equality, which is a useful expression
in a slightly different context, using trigonometric relations. The
average of cos2 θ over all pairs is a popular statistic in
galaxy cluster alignment studies, where in this context θ
corresponds to the angle between the major axis of the satellite
distribution and the line to the other cluster in the pair (e.g.
Smargon et al.,
2012).
All these predictions originate from the ansatz in Equation (13), which
leads to a linear scaling of the intrinsic shear with matter density
contrast and is thus termed the linear alignment model. It is
widely considered appropriate for elliptical galaxies, as well as the
distribution of galaxies within clusters, as these are believed to trace
the shape of the underlying dark matter haloes.
For disc galaxies it seems physically reasonable to consider a model that relates galaxy angular momentum to the tidal field. Using Equation (11), White (1984) showed that the angular momentum of a proto-galaxy is given by the expression
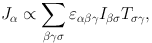 |
(18) |
where єαβγ is the Levi-Civita symbol. If the inertia and tidal tensors are perfectly aligned, i.e. if they are diagonal in the same coordinate system, no angular momentum is generated. However, since Iβσ is determined solely by the proto-galaxy's shape, whereas Tσγ is dominated by large-scale distribution of matter (especially when the potential has been smoothed), this is generally not expected. Simulations indicate that significant correlation between the inertia and shear tensors is present (Porciani et al., 2002b), which will suppress the magnitude of the resulting angular momenta and their correlations. To account for this, Lee & Pen (2000) proposed the following effective one-parameter model for the Gaussian angular momentum distribution p(Jα|Tασ) for a given tidal shear, by making an ansatz for the covariance matrix ⟨Jα Jα′ ⟩ between the angular momentum components,
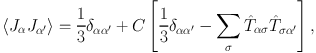 |
(19) |
where
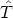 ασ
is the normalised, trace-less tidal
shear tensor and δαα′ is
the Kronecker-δ. It can be derived from the gravitational
quadrupole by subtraction of the trace,
ασ
is the normalised, trace-less tidal
shear tensor and δαα′ is
the Kronecker-δ. It can be derived from the gravitational
quadrupole by subtraction of the trace,
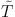 αα′ =
Tαα′ −
δαα′
Tr(T) / 3 and subsequent normalisation,
αα′ =
Tαα′ −
δαα′
Tr(T) / 3 and subsequent normalisation,
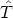 ασ
=
ασ
= 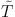 ασ /
(
ασ /
(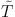 αρ
αρ
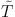 ρσ)1/2,
where the Einstein summation
convention over repeated indices is implied. For C = 1,
Equation (19) reproduces the result for angular momentum
correlations obtained when assuming completely uncorrelated inertia and
shear tensors
(Lee & Pen 2001),
which yields the tightest coupling of the angular momentum to the tidal
field. A value C < 1 is expected due to non-linear evolution
because of the partial alignment of the inertia and shear tensors, while
in the limit C = 0 the angular momentum directions are
randomised. Assuming a Gaussian distribution of the components of the
angular momentum vector,
Lee & Pen (2001)
then deduced the correlation of the directions of angular momenta (as
opposed to the correlation of the full angular momentum components in
the foregoing equation),
ρσ)1/2,
where the Einstein summation
convention over repeated indices is implied. For C = 1,
Equation (19) reproduces the result for angular momentum
correlations obtained when assuming completely uncorrelated inertia and
shear tensors
(Lee & Pen 2001),
which yields the tightest coupling of the angular momentum to the tidal
field. A value C < 1 is expected due to non-linear evolution
because of the partial alignment of the inertia and shear tensors, while
in the limit C = 0 the angular momentum directions are
randomised. Assuming a Gaussian distribution of the components of the
angular momentum vector,
Lee & Pen (2001)
then deduced the correlation of the directions of angular momenta (as
opposed to the correlation of the full angular momentum components in
the foregoing equation),
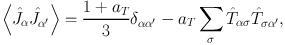 |
(20) |
where Ĵ is the normalised spin vector. This equation looks deceptively similar to Equation (19), but note that aT is now a parameter that runs between 0 (random angular momenta) and 3/5 (maximum alignments).
Assuming that the ellipticity of a galaxy is given by the projection of a circular disc which is orthogonal to the angular momentum direction of the galaxy, the intrinsic shear can be computed as (Catelan et al., 2001, Crittenden et al., 2001)
 |
(21) |
where the coordinate system is defined as above: the z-direction is parallel to the line-of-sight and the indices run over the x- and y-directions. Crittenden et al., (2001) proceeded to show that
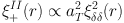 |
(22) |
holds for a correlation function of intrinsic shears, measured as a function of three-dimensional pair separation r. Here, ξδδ is the correlation function of the matter density contrast (the Fourier transform of Pδδ). This proves that the parameter aT only modifies the amplitude of correlations, and is thus degenerate with the impact of finite disc thickness and a stochastic misalignment between the spins of the stellar and dark matter components of a galaxy. This quadratic alignment model predicts correlations that scale with the square of the matter correlation function or power spectrum to lowest order, which suppresses the alignment signals. Besides, in linear theory there are no correlations between matter and quadratically aligned intrinsic shear if both fields are Gaussian (Hirata & Seljak 2004). Note however that non-linear evolution may introduce a linear scaling (Hui & Zhang, 2002), so that disc galaxy alignments could in principle feature signals of the form predicted by the linear alignment model. Equation (20) has also been used to derive distributions of alignment angles between angular momentum directions and the principal axes of the tidal tensor; e.g. in the case of void surfaces one obtains for the angle θ between the angular momentum direction and the surface normal (Lee & Erdogdu 2007; in the corrected form by Slosar & White 2009)
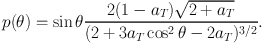 |
(23) |
1 For an alternative formation hypothesis of spheroids involving cold gas streams, which is also closely linked to alignments with the surrounding dark matter and gas distribution, see e.g. Dekel et al., (2009). Back.
2 There is a subtlety involved in this approximation: for an individual galaxy, as Equation (2) has been written, the expansion produces another term that is first order in the shear and proportional to g* (єs)2. However, since the relation is only considered in practice when averaging over large numbers of galaxies, this term (as well as all higher-order terms) becomes negligible if the intrinsic galaxy shapes are uncorrelated, or only weakly correlated, with the shear acting on them. Back.
3 The minus sign in these definitions ensures that the tangential alignment of shear around an object yields a positive signal. As a caveat, measurements of galaxy alignments tend to omit the minus sign in related statistics because in this situation the generally expected radial alignment is desired to yield a positive signal. Back.
4 An aside on nomenclature: galaxy alignments often receive the attribute intrinsic, especially if the physical alignments inherent to the galaxy population need to be distinguished from the apparent alignments on galaxy images induced by gravitational lensing (occasionally denoted as extrinsic; see Catelan et al., 2001). The term is also applied in a slightly different context to distinguish between the physical three-dimensional shape of a galaxy and its projected shape we observe on the sky (see Sandage et al., 1970 for the earliest occurence that we could trace). Back.
5 As we will discuss later, this model describes the observed galaxy alignments of bright early-type galaxies rather well, including its redshift evolution (see also Kirk et al., 2015). This is somewhat puzzling because these galaxies are thought to have been created only recently (typically at redshifts below two) by major mergers, disruptive events that should erase all memory of alignment processes during galaxy formation. Hence, the assumptions underlying Equation (14) may not be fully valid, but it could be that any modifications would primarily affect the amplitude of the predicted correlations, which is unconstrained by the model anyway. Back.