


The general implications of pseudobulges for galaxy formation are the main subject of this Winter School. Here, I focus on two additional astrophysical implications that came – at least to me – as a surprise. First is the challenge that classical-bulge-less galaxies (even ones that contain pseudobulges) present for our picture of galaxy formation by hierarchical clustering and merging. Second is the lack of any tight correlation between the masses of supermassive black holes and the properties of pseudobulges.
Look at any movie of a numerical simulation of hierarchical clustering in action. Your overwhelmingly strong impression will be that the lives of dark matter halos are violent. They continually collide with and accrete smaller halos, which – by and large – approach from random directions. And virtually no halo grows large 3 without undergoing at least a few major mergers between progenitors of comparable mass.
Given this merger violence, how can there be so many bulgeless galaxies? The puzzle has two parts. How does hierarchical clustering prevent the formation of classical bulges that are the scrambled-up remnants of the progenitor stars that predate the merger? And how does the merger assembly of galaxy halos prevent the destruction of large but very flat disks, at least some of which are made in part of old stars. Bulgeless disks are not rare.
Figure 48 shows the purest examples of this problem, the iconic late-type galaxies whose edge-on orientations make it clear that they have no classical bulges and no signs of pseudobulges. Such galaxies are common (Karachentsev et al. 1993; Kautsch et al. 2006). UGC 7321 is studied by Matthews et al. (1999a); Matthews (2000); Banerjee et al. (2010). IC 5249 is studied by van der Kruit et al. (2001). Matthews et al. (1999b), Kautsch (2009) and van der Kruit & Freeman (2011) review superthin galaxies.
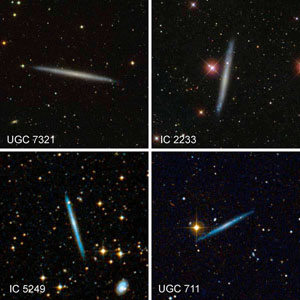 |
Figure 48. Edge-on, completely bulgeless, pure-disk galaxies. All images are from http://www.wikisky.org; the top galaxies are from the SDSS and the bottom ones are from DSS. The DSS images have a bluer color balance than the SDSS. |
It is a challenge to explain these galaxies. It helps that they are not large: Hyperleda lists rotation velocities of Vcirc = 95, 79, 97 and 92 km s-1 for UGC 7321, IC 2233, IC 5249 and UGC 711, respectively. This is smaller than Vcirc = 135 ± 10 km s-1 in M 33 (Corbelli & Salucci 2000; Corbelli 2003). Explaining bulgeless disks is least difficult for dwarf galaxies. They suffer fewer mergers and tend to accrete gas in cold streams or as gas-rich dwarfs (Maller et al. 2006; Dekel & Birnboim 2006; Koda et al. 2009; Brooks et al. 2009; Hopkins et al. 2009d, 2010). Energy feedback from supernovae counteracts gravity most effectively in dwarf galaxies (Dekel & Silk 1986; Robertson et al. 2004; D'Onghia et al. 2006; Dutton 2009; Governato et al. 2010). Attempts to explain pure disks have come closest to success in explaining dwarf galaxies (Robertson et al. 2004; Governato et al. 2010).
Kormendy et al. (2010) conclude that the highest-mass, pure-disk galaxies are the ones that most constrain our formation picture. They inventory all giant galaxies (Vcirc ≥ 150 km s-1) at distances D ≤ 8 Mpc within which we can resolve small enough radii to find or exclude even the smallest bulges. Table 3 documents the B / T and PB / T luminosity ratios for these galaxies. Giant, bulgeless galaxies are not rare. Figure 49 shows the most extreme galaxies in which B / T = 0 rigorously. Kormendy et al. (2010) emphasize that "we do not have the freedom to postulate classical bulges which have arbitrary properties (such as low surface brightnesses) that make them easy to hide. Classical bulges and ellipticals satisfy well-defined fundamental plane correlations (Fig. 68). Objects that satisfy these correlations cannot be hidden in the above galaxies. So B / T = 0 in 4/19 of the giant galaxies in our sample." Seven more galaxies contain pseudobulges; since we believe that these are grown secularly out of disks and not made via mergers, these are pure-disk galaxies from the hierarchical clustering point of view. Four more galaxies contain classical bulges smaller than any that are made in hierarchical clustering simulations. Only M 31 and M 81 have classical bulges with B / T ≃ 1/3, and only two more galaxies are ellipticals with B / T = 1.
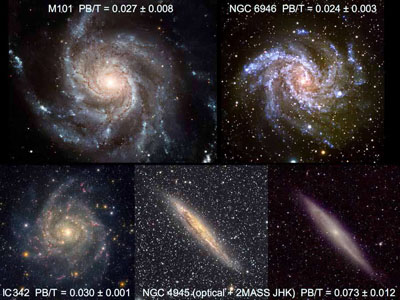 |
Figure 49. Face-on, completely bulgeless, pure-disk galaxies. All four galaxies have bulge-to-total luminosity ratios of B / T = 0. They have the smallest pseudobulges in the local sample of giant galaxies (outer rotation velocities Vcirc > 150 km s-1; for these galaxies, 174–210 km s-1) in Table 3. The pseudobulge-to-total luminosity ratios PB / T are given in the figure. Unless otherwise noted, the images are from http://www.wikisky.org or Kormendy et al. (2010). |
Fisher & Drory (2011) derive similar statistics in the D ≤ 11 Mpc volume.
| Galaxy | Type | D (Mpc) | MK | MV | Vcirc (km s-1) | B / T | PB / T |
| (1) | (2) | (3) | (4) | (5) | (6) | (7) | (8) |
| NGC 6946 | Scd | 5.9 | -23.61 | -21.38 | 210 ±10 | 0 | 0.024 ±0.003 |
| NGC 5457 | Scd | 7.0 | -23.72 | -21.60 | 210 ±15 | 0 | 0.027 ±0.008 |
| IC 342 | Scd | 3.28 | -23.23 | -21.4 : | 192 ± 3 | 0 | 0.030 ±0.001 |
| NGC 4945 | SBcd | 3.36 | -23.21 | -20.55 | 174 ±10 | 0 | 0.036 ±0.009 |
| NGC 5236 | SABc | 4.54 | -23.69 | -21.0 | 180 ±15 | 0: | 0.074 ±0.016 |
| NGC 5194 | Sbc | 7.66 | -23.94 | -21.54 | 240 ±20 | 0: | 0.095 ±0.015 |
| NGC 253 | SBc | 3.62 | -24.03 | -20.78 | 210 ± 5 | 0: | 0.15 |
| Maffei 2 | SBbc | 3.34 | -23.0 : | -20.8 : | 168 ±20 | 0: | 0.16 ±0.04 |
| Galaxy | SBbc | 0.008 | -23.7 | -20.8 : | 220 ±20 | 0: | 0.19 ±0.02 |
| Circinus | SABb: | 2.8 | -22.8 | -19.8 | 155 ± 5 | 0: | 0.30 ±0.03 |
| NGC 4736 | Sab | 4.93 | -23.36 | -20.66 | 181 ±10 | 0: | 0.36 ±0.01 |
| NGC 2683 | SABb | 7.73 | -23.12 | -19.80 | 152 ± 5 | 0.05 ±0.01 | 0: |
| NGC 4826 | Sab | 6.38 | -23.71 | -20.72 | 155 ± 5 | 0.10 | 0.10 |
| NGC 2787 | SB0/a | 7.48 | -22.16 | -19.19 | 220 ±10 | 0.11 | 0.28 ±0.02 |
| NGC 4258 | SABbc | 7.27 | -23.85 | -20.95 | 208 ± 6 | 0.12 ±0.02 | 0: |
| M31 | Sb | 0.77 | -23.48 | -21.20 | 250 ±20 | 0.32 ±0.02 | 0 |
| M81 | Sab | 3.63 | -24.00 | -21.13 | 240 ±10 | 0.34 ±0.02 | 0 |
| Maffei 1 | E | 2.85 | -23.1 : | -20.6 : | (264 ±10) | 1 | 0 |
| NGC 5128 | E | 3.62 | e-23.90 | -21.34 | (192 ± 2) | 1 | 0: |
NOTE. - Galaxies are ordered from pure disk to pure elliptical by increasing pseudobulge-to-total luminosity ratio PB / T and then by increasing bulge-to-total luminosity ratio B / T. Column (2): Hubble type. Column (3): Distance. Columns (4) and (5): Absolute magnitudes MK and MV are calculated from apparent integrated magnitudes (in K band, from Jarrett et al. 2003; in V band, preferably from HyperLeda, otherwise from NED). Galactic absorptions are from Schlegel et al. (1998). Column (6): Circular rotation velocity at large radii, Vcirc, corrected to edge-on inclination. Values in parentheses are √2σ Columns (7) and (8) are averages of measured classical bulge-to-total and pseudobulge-to-total luminosity ratios. Quoted errors are from the variety of decompositions in multiple sources. The smallest values are unrealistically optimistic estimates of the true measurement errors and indicate fortuitously good agreement between published values (e. g., for IC 342). Colons indicate uncertainty in the sense that no observational evidence suggests that this component is present but there is also no rigorous proof that a small contribution by this component is impossible. From Kormendy et al. (2010), who give sources. |
|||||||
Kormendy et al.
(2010)
conclude that giant bulgeless galaxies
do not form the rare tail of a distribution of formation histories that
include a few fortuitously mergerless galaxies. In the field, the
problem of forming giant, pure-disk galaxies by hierarchical clustering
is acute. In contrast, in the Virgo cluster,
 2/3 of the
stellar mass is in merger remnants. Therefore the problem of explaining
pure-disk galaxies is a strong function of environment.
2/3 of the
stellar mass is in merger remnants. Therefore the problem of explaining
pure-disk galaxies is a strong function of environment.
This is a sign that AGN feedback, the physics popularly used to address the problem, is not the answer. The effectiveness of energy feedback depends on galaxy mass. In contrast, galaxies tell us that environment is the controlling factor. Giant, pure-disk galaxies (Fig. 49) are more massive than small ellipticals. And the thin disk of our Galaxy – which, given its boxy bar, is a giant pure-disk galaxy – contains stars as old as 10 Gyr (Oswalt et al. 1996; Winget & Kepler 2008). So I suggest that the solution to the pure-disk problem is not to use energy feedback to delay disk star formation in order to give the halo time to grow without forming a classical bulge. I believe that a viable solution must use the environmental dependence of the pure-disk galaxy problem in an essential way (e. g., Peebles & Nusser 2010).
6.2. Supermassive black holes do not correlate with pseudobulges
Kormendy & Ho (2013) review a modest revolution that is in progress in studies of supermassive black holes (BHs) in galaxy centers. For more than a decade, observed BH demographics have suggested a simple picture in which BH masses M• show a single correlation each with many properties of their host galaxies (Fig. 50). Most influential was the discovery of a tight correlation between M• and the velocity dispersion σ of the host bulge at radii where stars mainly feel each other and not the BH (Ferrarese & Merritt 2000; Gebhardt et al. 2000; Tremaine et al. 2002; Gültekin et al. 2009). Correlations are also observed between M• and bulge luminosity (Kormendy 1993a; Kormendy & Richstone 1995; Magorrian et al. 1998), bulge mass (Dressler 1989; McLure & Dunlop 2002; Marconi & Hunt 2003; Häring & Rix 2004); core parameters of elliptical galaxies (Milosavljevic et al. 2002; Ravindranath et al. 2002; Graham 2004; Ferrarese et al. 2006; Merritt 2006; Lauer et al. 2007; Kormendy & Bender 2009), and globular cluster content (Burkert & Tremaine 2010; Harris & Harris 2011). These have led to the belief that BHs and bulges coevolve and regulate each other's growth (e. g., Silk & Rees 1998; Richstone et al. 1998; Granato et al. 2004; Di Matteo et al. 2005; Springel et al. 2005; Hopkins et al. 2006; Somerville et al. 2008).
This simple picture is now evolving into a richer and more plausible story in which BHs correlate differently with different kinds of galaxy components. BHs do not correlate at all with galaxy disks (Kormendy & Gebhardt 2001;
Kormendy et al. 2011; Kormendy & Ho 2013), although some pure-disk galaxies contain BHs (see Ho 2008 for a review). And despite contrary views, (Ferrarese 2002; Baes et al. 2003; Volonteri et al. 2011), it is clear that BHs do not correlate tightly enough with dark matter halos to imply any special relationship between them beyond the fact that dark matter controls most of the gravity that makes hierarchical clustering happen (Ho 2007; Kormendy & Bender 2011; Kormendy & Ho 2013). So BHs coevolve only with bulges.
What about pseudobulges? They are closely connected with disks, but some contain BHs. The best example is our Galaxy (Genzel et al. 2010).
Hu (2008) finds, for a small sample, that BHs in pseudobulges have smaller M• than BHs in classical bulges and ellipticals of the same σ. Graham (2008) reports the possibly related result that barred galaxies also deviate from the M• – σ relation in having small M•, but interpretation is complicated by the fact that some of his barred galaxies contain classical bulges (e. g., NGC 1023, NGC 4258), some contain pseudobulges (e. g., NGC 3384, our Galaxy) and some contain both (NGC 2787). More definitively, results similar to Hu's are found by Nowak et al. (2010) and by Greene et al. (2010).
Kormendy et al. (2011) and Kormendy & Ho (2013) now show for larger samples that pseudobulges correlate little enough with M• so coevolution is not implied (Fig. 50). This simplifies the problem of coevolution by focusing our attention on galaxy mergers. It is a substantial success of the secular evolution picture that a morphological classification of bulges separates them into two kinds that correlate differently with BHs.
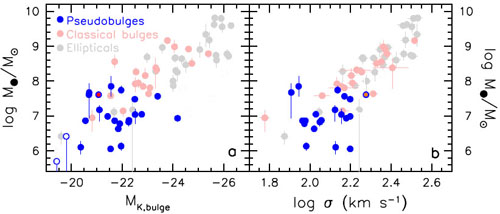 |
Figure 50. Correlation of dynamically measured BH mass M• with (left) K-band bulge absolute magnitude and (right) velocity dispersion averaged inside re. Pseudobulges with dynamical BH detections are shown with blue filled circles and those with M• upper limits are shown with blue open circles. NGC 2787 may have both a small classical and a large pseudo bulge (Erwin et al. 2003); its blue symbol has a red center. Classical bulges and ellipticals are shown in ghostly light colors to facilitate comparison. This is a preliminary figure from Kormendy & Ho (2013). |
3 In this review, as in Kormendy et al. (2010), I will adopt the sufficient and practical definition that a "large" galaxy is one in which the circular orbit rotation velocities of massless test particles at large radii are Vcirc ≥ 150 km s-1. Back.