4.2.2. Central Velocity Dispersions. I. The Faber-Jackson Relation

Since the measurement of rotation and dispersion profiles in ellipticals has only recently become practical, the most extensive stellar-kinematic measurements available are those of central velocity dispersions . These allow us to estimate central mass-to-light ratios (section 4.2.3). Also, the discovery of a correlation between and metallicity indicates that ellipticals are at least a two-parameter family (section 4.2.4). A necessary tool for both of these applications is a fundamental correlation between and the total blue luminosity L_B (Faber and Jackson 1976), which has the approximate form L_B ⁿ, n 4.

This relation has since been confirmed by many authors (S²BS; Schechter and Gunn 1978; Schechter 1980; Terlevich et al. 1981; Tonry and Davis 1981b; de Vaucouleurs and Olson 1982, and others), who added more measurements and refined the parameters n and the zero point. Since L_B is proportional to the adopted distance and is not, the Faber-Jackson relation also provides a new way of measuring relative distances. In particular, it has been used to map the velocity field in the local supercluster, and thereby to derive the Local Group infall velocity W₀ toward Virgo. Two independent solutions give the following results:

Schechter (1980):

(20)

Tonry and Davis (1981b):

The power n of the Faber-Jackson relation is not sensitive to the Virgocentric flow field. Kormendy and Illingworth (1982b) obtain n = 5.4 (+0.9, -0.7) by assuming a uniform Hubble flow and n = 5.2 (+0.8, -0.6) based on a linear model (Schechter 1980) with W₀ = 300 km s^-1 (Aaronson et al. 1982). I will adopt n = 5.4 here, but note that this is uncertain, with values as small as n = 3.6 ± 0.3 reported (de Vaucouleurs and Olson 1982). The adopted relation is shown in Figure 33.

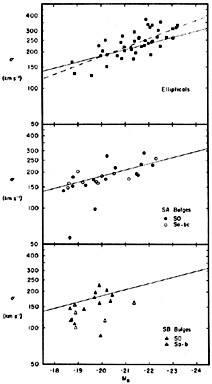Figure 33. Correlations between central velocity dispersions and absolute magnitudes MB for elliptical galaxies and for bulges of unbarred and barred disk galaxies (Kormendy and Illingworth 1982b). Edge-on galaxies are omitted. The solid line is in each case the L n relation for SA0-bc galaxies, n = 7.8(+1.9, -1.3), 21 = 208 km s-1. The two very discrepant galaxies, NGC 1172 (upper) and NGC 7457, have been omitted from this solution. The dashed line is a least-squares fit for the ellipticals, n = 5.4(+0.9, -0.7), 21 = 217 km s-1. Zero points for least-squares fits to various subsamples of the data are given under the figure. For each set of solutions the value of n is fixed.

Recent work suggests that the Faber-Jackson relation may not be a simple power law. At the low-luminosity end, a downward curvature of the relation in Figure 33 is indicated by Tonry's (1981) analysis of galaxies down to M_B = -18.0. This study has the advantage that the galaxies are all in the Virgo cluster, so relative distance errors cannot affect the value of n. For ellipticals fainter than M_B ~ - 20, Tonry finds that L_B ^3.2±0.2. Similarly, Davies et al. (1983) find n = 2.4 ± 0.9 for 14 ellipticals fainter than M_B = -20, and n = 4.2 ± 0.9 for 30 brighter ellipticals. If this effect is confirmed in larger samples, several explanations are possible. (1) The mass-to-light ratio may be a steeper function of luminosity in faint galaxies than in bright ones; see section 4.2.3. (2) Rotation may provide some of the dynamical support in low-luminosity ellipticals, which are known to rotate rapidly (section 4.2.6). (3) Velocity dispersion gradients may be averaged by the large measuring aperture used, 3" × 12". This is a special problem for faint ellipticals because they, have small dynamical characteristic radii. If the - r_c relation of Figure 22 holds for these galaxies, they have core radii r_c ~ 0.5". Any dispersion gradients generally begin just outside r = r_c (see Fig. 35). But r_c is much smaller than the measuring aperture.

At the high-luminosity end, Malumuth and Kirshner (1981, Fig. 1) suggest that the log - M_B correlation levels off, in that brightest cluster galaxies do not have larger dispersions than slightly fainter galaxies. The deviation from the adopted L ⁿ relation is especially large for three cD galaxies measured. However, most of this effect is due to the contribution to M_B of the cD halo; when this is removed the galaxies do not deviate significantly. As noted by Malumuth and Kirshner, this is consistent with the assumption (see section 3.3.4) that the halos are dynamically distinct features added to basically normal, although very bright, ellipticals.

Table 4. ZERO POINTS OF L ⁿ RELATIONS

E (n = 5.4):	21 =	217 ± 6 km s-1	E (n=7.8):	21 =	222 ± 6 km s-1
SA0 :		218 ± 10 km s-1	SA0 :		211 ± 9 km s-1
SAa-bc :		217 ± 9 km s-1	SAa-bc :		205 ± 7 km s-1
SB0-b :		185 ± 10 km s-1	SB0-b :		172 ± 10 km s-1

In section 3.4.1 I discussed a number of physical differences between ellipticals and the bulges of spiral galaxies. The Faber-Jackson relation provides another probe of such differences. Interestingly, the nuclear dynamics of ellipticals and normal bulges are found to be indistinguishable.

Early reports seemed to tell a different story. Whitmore, Kirshner and Schechter (1979) and Whitmore and Kirshner (1981, hereafter collectively WKS) found that ellipticals and S0s have the same L ⁿ relation, but bulges of spiral galaxies have velocity dispersions smaller by 17 ± 8% than ellipticals of the same luminosity. If we use as the zero point of the L ⁿ relation the dispersion ₂₁ at M_B = - 21(H₀ = 50 km s^-1 Mpc^-1), then WKS found ₂₁ = 228 ± 11 km s^-1 in ellipticals, 220 ± 15 km s^-1 in S0 bulges and 190 ± 10 km s^-1 in spiral bulges. All bulge magnitudes were corrected for disk light as in section 3.4.3. Several effects could contribute to the above difference. (1) Much of the photometry used was not accurate enough for reliable profile decomposition. (2) Rotation of bulges would decrease the amount of velocity dispersion required to support the galaxy. However, S0 bulges rotate as rapidly as those of spirals (section 4.2.6) and are not colder than ellipticals. (3) The bulges could have lower M/L values due to recent star formation. In fact, Whitmore and Kirshner (1981) make the prophetic statement that "most of the spiral bulges do fall very close to the [L ⁿ] line for ellipticals, and only a few galaxies (perhaps NGC 4303 and NGC 4321 . . . ) undergoing a recent burst [of star formation] provide the increased scatter and the gap with the ellipticals."

Recently, Kormendy and Illingworth (1982b) have re-examined the L ⁿ relation for disk-galaxy bulges, motivated by the following worries about the WKS analysis. First, many WKS "bulges" clearly contain Population I material. Essentially, the galaxies are too late in type to contain bulges which resemble ellipticals. What is the L ⁿ relation for bulges, like those of M31 and M81, which are similar to ellipticals? Second, we will see in section 5 that bulges of barred galaxies are more disk-like than SA bulges. Do they also have different L ⁿ relations? Third, Kormendy and Illingworth retain only those galaxies with photometrically well determined bulge magnitudes. Finally, they verify that plausible Virgocentric flow fields do not affect the conclusions.

The results are shown in Figure 33. Galaxies which clearly have young stars in their nuclei are omitted (e.g., NGC 4321, see the spectrophotometry of Turnrose 1976). There is then no difference in the slope or zero point for ellipticals, or bulges of unbarred S0 or Sa-bc galaxies. On the other hand, many bulges of barred galaxies, even SB0s, have lower dispersions than SA bulges of the same luminosity. A possible interpretation is discussed in section 5.2.

Evidently the difference in zero point found by WKS was due to the inclusion of late-type galaxies whose disks contribute light even at the center, and barred galaxies whose bulges differ systematically from SA bulges. The global differences between ellipticals and ordinary bulges are not reflected in their central dynamics, to the accuracy of the present observations. This is not implausible if the global differences are caused by a combination of rotation and the disk potential in bulges; neither effect should be very important near the center.