1. INTRODUCTION

The advent of new large telescopes coupled with new instrumentation technologies in the last decade has been extremely powerful in expanding our view of the high-redshift Universe. In particular, we have seen a flowering of the topic of high-redshift galaxy kinematics which studies their internal motions through high spatial and spectral resolution observations. The number of papers has exploded and we have seen a variety of surveys of observational approaches, analysis techniques, and theoretical interpretations. This has led to new paradigms of the nature of young galaxies but it has also raised problems in understanding as many new techniques have been used making comparison with the local Universe and traditional techniques difficult.

The Publications of the Astronomical Society of Australia has decided to launch this new series of major reviews in honour of Lt. William Dawes. I have chosen to write it on the topic of these exciting new studies of the kinematics of high-redshift star-forming galaxies, one which has not had a major review and is in need of one. This is the first such Dawes review and as such there is no tradition to follow, instead one gets to set the tradition. I will choose to write this as a high-level introduction to the field, perhaps akin to the style of lecture notes, for the new worker in the field (for example an incoming postgraduate student). As such I will try and favour clarity and simplicity of explanations over totally complete lists of all possible references and ideas on a topic and will discuss analysis techniques in some detail. I will highlight the main surveys and the main ideas and warn in advance that some things may get left out. I will also allow myself the freedom to give more scientific speculation of my own than would occur in a traditional review, however it will be clearly indicated what is a speculation. Obviously I will use the first person when needed as this seems appropriate for my approach.

1.1. Background and scope of this review

The rotation of the 'spiral nebulae' was one of the earliest and most fundamental observations of their nature and the second important discovery from their spectroscopy. Almost exactly 100 years ago in 1912 September, Vesto M Slipher measured the first spectrum and first redshift of a galaxy using a new fast spectrograph he had built (Slipher 1913). This galaxy was M31 and the redshift was actually a blueshift of 300 km/s — this was highly unexpected at the time, it was ten times higher than any previous velocity measured for an astronomical object. Slipher himself thought it good evidence for the extragalactic model of spiral nebulae (Bartusiak 2009) and proceeded to embark on a campaign to measure many more velocities (Slipher 1917) eventually resulting in one axis of Hubble's famous diagram (Hubble 1929).

Less well-known is that during this first campaign Slipher also discovered the rotation of galaxies (Slipher 1914) — he noticed the tilt of the spectral lines whilst observing the Sa galaxy M104 and noted the similarity to the same phenomenon when observing planets. Slipher had worked for Lowell for many years measuring the day lengths of various planets. Slipher commented: 'Although from the time of Laplace it has been thought that nebulae rotate, this actual observation of the rotation is almost as unexpected as was the discovery that they possessed enormously high radial velocities'.

We now regard galaxies as gravitationally bound extragalactic objects and their internal motions relate to fundamental questions about their masses and assembly history. In particular the last seven years have seen a wealth of new high-redshift observations measuring for the first time the kinematics of galaxies in the early Universe and producing new pictures of star-forming galaxies. These are the topic for this review. I note that I will favour the term 'kinematics' which describes, from observations, the motions of astronomical objects (as opposed to the term 'dynamics' which describes the theoretical causes of such motions).

Figure 1. William Dawes was a Royal Marine officer on the 'First Fleet' arriving in Australia in 1788. He was a man of many talents: engineer, map maker, botanist, and amateur astronomer. He was one of the first to document the Aboriginal Australian languages spoken in the Sydney region. He was the first person to make astronomical observations in Australia using telescopes from a place in Sydney Cove now known as Dawes Point (Mander-Jones 1966). Image Credit: miniature oil painting of Lieutenant William Dawes, 1830s, artist unknown. Collection: Tasmanian Museum and Art Gallery. Reproduced with their permission.

Large 8-10m class optical telescopes ¹ with their light grasp and angular resolution have been critical for the development of this subject but equally important has been the associated development of astronomical instrumentation sitting at the focal plane.

Integral Field Spectroscopy (IFS) has played a pivotal role due to the complex structures of high-redshift objects. With this technique, it is possible to collect a spectrum of every point in the 2D image of an object, which is contrasted with the classical technique of long-slit spectroscopy where spectra are collected along a 1D slice (whose direction must be chosen in advance) through an object. An IFS generally works by reformatting a 2D focal plane, and there are various ways of accomplishing this (for a review of the technology, see Allington-Smith 2006) but a general principle is that because instruments are limited by the number of pixels in their focal plane detectors, an IFS typically has a small field of view with spatial sampling of order 1000 elements ² suitable for single object work. (This is an area that is likely to improve in the future with new instruments and ever large pixel-count detectors).

Adaptive Optics (AO) technology which corrects for atmospherical turbulent blurring of images has also become routine on large telescopes (Davies & Kasper 2012) over the last decade and has allowed the achievement of the angular diffraction limit on 8-10m telescopes — typically 0.1 arcsec instead of the 0.5-1 arcsec seeing limit imposed by the atmosphere. This is important as 1 arcsec corresponds to 8 kpc for 1 < z < 3 which is comparable to the sizes of disc galaxies at these redshifts (e.g. Ferguson et al. 2004, Buitrago et al. 2008, Mosleh et al. 2011). AO observing comes with its own sets of limitations imposed by the requirements to have bright stars or laser beacons to measure AO corrections from and have generally not been possible for all objects in large samples.

It is important when writing a review to carefully define the scope. The topic will be the kinematics of star-forming galaxies at high-redshift (which I will define as z > 0.5), with a focus on what we have learned and how we have learned it, from IFS and AO observations. It is not possible to cover, with any comprehensiveness related topics such as (i) general physical properties of high-redshift star-forming galaxies, (ii) the kinematics of star-forming galaxies in the local Universe, and (iii) the kinematics of non-star forming 'red and quiescent' galaxies at high-redshift. The first two are already the subject of extensive reviews to which I will refer, and the last is a rapidly burgeoning field which will probably be due for its own review in 2-3 years as the number of observations increases tremendously with the advent of multi-object near-IR spectrographs. ³ However, some non-comprehensive discussion of each of these (especially the first two) will be given to set the scene.

The plan and structure of this review is as follows. Firstly, in the remainder of this introduction I will briefly discuss the kinematic properties of galaxies in the modern Universe to frame the comparisons with high-redshift. In Section 2, I will review the earliest kinematic observations of star-forming galaxies at high-redshift from longslit techniques. In Section 3, I will review the most important large high-redshift IFS surveys, how they are selected and carried out and their most important conclusions. In Section 4, I will review the kinematic analysis techniques used by IFS surveys with reference to the surveys in Section 3. In Section 5, I will compare and contrast what we are learning about the physical pictures of high-redshift star-forming galaxies from the various IFS surveys and discuss, in particular, the 'turbulent clumpy disc' paradigm that has arisen from these works. In Section 6, I will point to the future, the outstanding questions and the future instruments, telescopes, surveys and techniques that may address them.

This review will adopt a working cosmology of Ω_m = 0.3, Ω_Λ = 0.7, H₀ = 70 km s^-1 Mpc^-1 (Spergel et al. 2003). Since most of the work discussed has been in the last decade, the authors have adopted cosmologies very close to these resulting in negligible conversion factors in physical quantities. I will adopt the use of AB magnitudes.

1.2. Kinematics of star-forming galaxies in the local Universe.

In the local Universe, we see a distinct separation of galaxies in to two types with red and blue colours (Strateva et al. 2001, Baldry et al. 2004) commonly referred to as the 'red sequence' and 'blue cloud' reflecting the relative tightness of those colour distributions. The separation is distinct in that there is a clear bimodality with a lack of galaxies at intermediate colours. These colour classes are very strongly correlated with morphology either as determined visually or via quantitative morphological parameters — a detailed recent review of these properties as derived from large statistical surveys such as the Sloan Digital Sky Survey and exploration of their dependence on other parameters such as environment is given by Blanton & Moustakas (2009). The correlation is sufficiently strong that virtually every massive system on the blue cloud is a rotating star-forming disc galaxy (usually spiral), though there is a rare population of 'red spirals' which overlap the red sequence (which is mostly ellipticals) that may arise from truncated star-formation, greater older stellar population contributions or dust (Masters et al. 2010, Cortese 2012).

There has been a number of reviews on the topic of the kinematics of local disc galaxies over the years, which should be referred to for a comprehensive discussion. In this section, I will discuss the most important points mostly referencing recent results whilst noting that the subject has a long history which has been well covered elsewhere. I refer the reader for more depth and history to van der Kruit & Allen (1978), who review the kinematics of spiral and irregular galaxies and Sofue & Rubin (2001), which is a more focussed review on the topic of rotation curves. A classic review of the structure of the Milky Way in particular was done by Gilmore et al. (1989). Recently vander Kruit & Freeman (2011) wrote a very comprehensive recent review of all properties of galaxy discs including kinematics.

For comparison with high-redshift, the most fundamental properties of local star-forming galaxies are their rotation and velocity dispersion, whose most important points I will review below. However, as we will see later in this review, star-forming galaxies at high-redshift show more kinematic diversity than in the local Universe including high fractions which are not dominated by rotation or which show complex kinematic signatures of mergers. Given evolutionary paths from high-redshift to low-redshift and from star-forming to quiescent are not obvious I will also discuss briefly the kinematics of local elliptical galaxies and mergers.

1.2.1. Rotation of local star forming galaxies

The earliest published work on disc galaxy rotation was that of Slipher (1914) but also see Pease (1916). They measured the rotation of several spirals between 1914 and 1925 including M31 and M104. The review of Sofue & Rubin (2001) gives a historical introduction, so also does the one of van der Kruit & Allen (1978). The early optical work was limited to the central regions of galaxies, the advent of radio telescopes and neutral hydrogen HI observations (van de Hulst et al. 1957) permitted measurements out at large radii where most of the angular momentum lies. Radio observations led to the well-known and most fundamental scaling of disc galaxies: the 'Tully-Fisher Relation' first reported by Tully & Fisher (1977) between optical luminosity and HI line width. If the HI line width, from an unresolved or marginally resolved single-dish observation, is thought of as tracing the total kinematic shear, then this becomes a relation between luminosity and rotation velocity, and hence luminosity and a measure of mass. Later, Tully-Fisher work has benefited from greatly increased spatial resolution and 2D kinematic mapping of the rotation field.

In the standard pictures, we now think of galaxies as inhabiting haloes of Cold Dark Matter (CDM), a non-baryonic component that dominates the dynamics and sets the scene for galaxy formation (Blumenthal et al. 1985, Ostriker 1993). The most fundamental of observations supporting this picture is the 'flat rotation curves' of disc galaxies (Rubin & Ford 1970, Roberts & Rots 1973, Rubin et al. 1978). The general picture is of a steeply rising rotation curve in the innermost few kpc followed by the 'flat' portion, which really means a turnover and then a slight slow decline in more luminous galaxies or a flatter more constant rotation in lower luminosity galaxies (Persic et al. 1996, Sofue & Rubin 2001). This occurs in a regime where the optical surface brightness is exponentially dropping off and the rotation velocity, as traced by HI, stays high past the outer edge of the optical disc. If light traced mass the velocity would drop off more sharply, this is the basic evidence for dark matter haloes (though is not universally accepted, for an alternative paradigm involving 'Modified Newtonian Dynamics' see Sanders & McGaugh 2002). If a dark matter halo was spherical and isothermal (ρ ∝ r^-2), one expects a perfectly flat rotation curve, in reality simulations predict more complex profiles for dark matter haloes (Navarro et al. 1997) and this, together with the stellar contributions, must be carefully considered when fitting rotation curve models (Kent 1987, Blais-Ouellette et al.2001). As such when defining the 'rotation velocity', one must be careful to specify at what radius this is measured. A common convention is to use 2.2 disc scalelengths ⁴ (from the surface photometry) as this is the radius where the rotation curve of a self-gravitating ideal exponential disc peaks (Freeman 1970a). This 'v_2.2' can also be related to the HI line width (Courteau 1997) which also probes the outer rotation. The typical values for large disc galaxies are in the range 150-300 km/s.

The original Tully-Fisher relation displayed a slope of L ∝ V^2.5 (based upon the luminosity from blue-sensitive photographic plates), modern determinations find an increasing slope with wavelength rising to a slope of V⁴ in the K-band or with stellar mass (Bell & de Jong 2001, Verheijen 2001). This is consistent with galaxies having a roughly constant ratio of dark matter to stellar mass globally ⁵ — which is in contrast to the resolved distribution within galaxies where clearly it does not. CDM theory predicts a slope closer to V³ based on scaling of dark matter halo properties (Mo et al. 1998). Some authors have argued that this represents an unreasonable 'fine-tuning' of the ΛCDM model and have proposed an alternative gravity 'MOND' mode without dark matter (e.g. Sanders & McGaugh 2002, McGaugh & de Blok 1998, McGaugh 2012), however small scatter can be accommodated within the ΛCDM framework (Gnedin et al. 2007, Avila-Reese et al. 2008, Dutton 2012). MOND does not seem to explain well larger scale structures such as galaxy groups and clusters in the sense that even with MOND there is still a need to invoke dark matter to explain the kinematics (Angus et al. 2008, Natarajan & Zhao 2008). This review will only consider the ΛCDM cosmological framework.

1.2.2. Velocity dispersion of local galaxy discs

We next consider the vertical structure and pressure support of galactic discs, as this will become quite a significant topic when comparing with high-redshift, where we will see substantial differences. The most obvious visible component of spiral galaxy discs is the so-called 'thin disc' which is where the young stellar populations dwell. The stellar component of the thin disc has an exponential scale height of 200-300 pc and a vertical velocity dispersion (σ_z) of ~ 20 km s^-1 (vander Kruit & Freeman 2011) — the dispersion is related to the vertical mass distribution by a gravitational equilibrium. This is σ_z² = a G Σ h_z where Σ is the mass surface density, h_z is the vertical exponential scale height, and a is a structural constant = 3π/2 for an exponential disc. In general, the dispersion of a stellar disc is a 3D ellipsoid (σ_R, σ_θ, σ_z). The radial (σ_R) and azimuthal (σ_θ) components are related by the Oort constants (giving σ_θ≃ 0.71 σ_R for a flat rotation curve) and the radial and vertical components are related to the discs structure and mass to light ratio with a typical value of σ_z / σ_R ~ 0.6 for large spirals (again see van der Kruit & Freeman and references therein for an extensive discussion of this).

The stellar age range of the Milky Way thin disc is up to 10 Gyr. Right in the middle of the thin disc is an even thinner layer where the gas collects — the neutral hydrogen, molecular clouds, dust, HII regions, and young OB and A stars all sit in this thinner layer which has a dispersion of only ~ 5-10 km s^-1 and scale height of 50 pc in the Milky Way. This thinner disc is where all of the star formation takes place today and in which the characteristic spiral structure of gas and young stars is apparent. In our Milky Way, the youngest stars (OBA spectral types) share the kinematics of the gas disc in which they form, as stellar age increases the velocity dispersion also increases — this kinematic evolution is interpreted as being due to stars on their orbits encountering 'lumps' in the disc, and scattering off them, such as giant molecular clouds (GMC's) and spiral arms. This gives rise to the thin stellar disc having on average a higher dispersion than the gas disc and young stars. The difference in velocity dispersion between different components gives rise to the phenomenon known as 'asymmetric drift'; for example, the rotation of the stellar disc lags behind that of the gas disc due to it's higher radial velocity dispersion which provides additional dynamical support against the galaxy's overall gravitational field.

Many external galaxies have their gas and kinematics observed in the Hα line of ionised hydrogen whose luminosity is generally dominated by HII regions. In the Milky Way, HII regions and GMCs share the low velocity dispersion (i.e. between cloud centres, Stark & Brand 1989) of the gas disc; however, it should be noted that the Hα line has a thermal broadening due to a characteristic temperature of 10⁴K of ~ 9 km s^-1 which will increase the observed line width. There is also a turbulent broadening due to internal motions in HII regions of order 20 km s^-1 (Mezger & Hoglund 1967, Shields 1990). Adding these in quadrature, we can see the typical dispersion is consistent with the range of 20-25 km s^-1 found by observations of external nearby spirals (Epinat et al. 2010, Andersen et al. 2006a).

The Milky Way also has a a so-called 'thick disc' stellar component (Gilmore & Reid 1983) (though there is still a debate as to whether this is a true dichotomy or a continuous stellar population sequence, e.g. Bovy et al. 2012a, Bovy et al. 2012b). Thick discs are now thought to be ubiquitous in spirals and may have masses that are, on average, up to values comparable to the thin disc (Comerón et al. 2011). The thick discs contain older, redder, and lower surface brightness populations and negligible on-going star-formation (Yoachim & Dalcanton 2008). The thick disc in our Milky Way has a scale height of ~ 1400 pc (Gilmore & Reid 1983). It is low metallicity ~ 1/4 Solar, is ~ 10 Gyr old (Gilmore et al. 1989) and has a vertical velocity dispersion of ~ 40 km s^-1 (Chiba & Beers 2000, Pasetto et al. 2012). Other spirals are thought to be similar. The origin of thick discs is a matter of debate and there are a variety of models — it may be formed from early merger events, satellite accretion, or secular evolution (see discussion in vander Kruit & Freeman 2011) and references therein). A particularly relevant scenario for our later discussion is the idea that the thick discs form in situ in early gas-rich high-dispersion discs (Bournaud et al. 2009).

Figure 2 illustrates these components schematically and also contrasts them with the emerging (but by no means certain) picture of z ~ 2 galaxies which we will return to in Section 5.1.

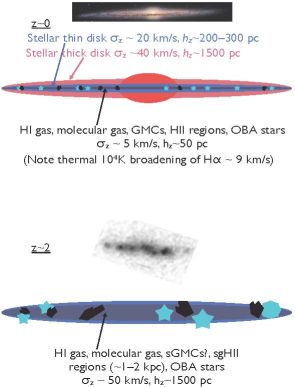Figure 2. Illustrative schematic showing the different structures of low-redshift and high-redshift disc galaxies in an edge-on view. Top: components of the Milky Way and similar local spirals (see Section 1.2.2) containing stellar thin/thick discs and a very thin gas disc in the centre. The latter contains all the Giant Molecular Clouds, HII regions, molecular and neutral gas and young stars. Bottom: a clumpy high-redshift disc (see Section 5.1). This contains a thick (~ 1 kpc scaleheight) and highly turbulent discs of molecular gas, young stars, super-giant HII regions (kpc scale star-forming 'clumps') and (presumably) super-Giant Molecular Clouds. Credit: inset images are of NGC 4565 (top, reproduced by permission of R. Jay GaBany, Cosmotography.com) and z ~ 3 galaxy UDF #6478 of Elmegreen & Elmegreen (2006) (their Figure 2, reproduced by permission of the AAS).

The measurement difference corresponds to whether we measure the line widths in spatially resolved spectra, and then average or whether we average the spectra and then measure the line width. Physically it is a distinction between different models of internal support against gravity (random motions vs rotational ones). In practise, any real observation, however fine, will average over some spatial scale and there will always be a contribution from large-scale and random motions to any line width, it is a question of degree and we will return to this point in Section 4.4. I will endeavour to be clear about what kind of velocity dispersion is being measured in what context.

1.3. Kinematic properties of elliptical galaxies.

While not the focus of this review, it is worth commenting briefly on the major kinematic properties of elliptical galaxies. In particular, one must bear in mind that possible evolutionary processes (such as star-formation 'quenching' and galaxy merging) may connect ellipticals at lower redshifts with star-forming galaxies at high-redshift. The historical picture of elliptical galaxies is of large, massive systems with negligible gas and star-formation with small rotation and kinematics dominated by velocity dispersion (de Zeeuw & Franx 1991). The elliptical galaxy analogy of the Tully-Fisher relation is the Faber-Jackson relation (Faber & Jackson 1976) relating the integrated velocity dispersion to the luminosity (or stellar mass). It should be noted that what was traditionally measured here is an integrated velocity dispersion of the brightest central part of the galaxy, usually with a long-slit spectrograph. The Faber-Jackson relation has now been extended to a 'Fundamental Plane' (Djorgovski & Davis 1987) where size, surface brightness, and velocity dispersion (equivalent to size, luminosity, and dispersion) are correlated to define a three parameter sequence with a reduced scatter (see reviews de Zeeuw & Franx 1991, Blanton & Moustakas 2009). ⁶

This classical picture has evolved considerably in the last decade with the availability of large-scale IFS observations of nearby elliptical galaxies. In particular, it is now known that a dominant fraction of elliptical galaxies are in fact rotating (Cappellari et al. 2007, Emsellem et al. 2007) and one can divide ellipticals in to two classes of 'slow rotators' and 'fast rotators' based on angular momentum. The slow rotators tend to be the most massive ellipticals (stellar masses > 3 × 10¹¹ M_⊙) and/or the ones found in the centres of rich clusters (Cappellari et al. 2011b, D'Eugenio et al. 2013). The kinematic division may relate to assembly history and the relative role of dissipative ('wet') and non-dissipative ('dry') mergers (e.g. Burkert et al. 2008) in building the most massive red-sequence galaxies. Detailed kinematics now goes beyond the simple fast/slow overall angular momentum division and in particular probing rotation in the outer parts of nearby ellipticals (i.e. well beyond the half-light radii) using IFS and multi-slit techniques provides detailed information on assembly histories (e.g. Proctor et al. 2009, Arnold et al. 2011).

So far these resolved kinematic observations of local ellipticals are limited to samples of only a few hundred objects, to be contrasted with Tully-Fisher observations of thousands of spiral galaxies, and it is not yet clear how the kinematic classes relate to the classical picture of the Fundamental Plane. This is likely to be an area of fruitful further research.

1.4. Kinematic properties of local mergers

As we will see, an important issue in studying galaxies at high-redshift is the kinematic separation of rotating disc galaxies from merging galaxies. At z > 1, the apparent merger rate is high and major mergers typically constitute up to 20-50% of observed samples depending on selection details and definition. So trying to systematically identify and classify them is important and critical to issues such as the high-redshift Tully-Fisher relationship.

Mergers are much rarer in the local Universe with major mergers being ~ 1-2% of all galaxies (Domingue et al. 2009, Xu et al. 2012) which is why Tully-Fisher relationships work so well. Departures from the mean relation may be correlated with peculiar velocity structures or recent star-formation history associated with merging (Kannappan et al. 2002, Mendes de Oliveira et al. 2003). There is actually a paucity of work systematically examining the kinematics of mergers perhaps due to this rarity. Typically papers discuss individual objects in detail, (Colina et al. 2005, Dasyra et al. 2006, Piqueras López et al. 2012) rather than trying to extract characteristic kinematic parameters for statistical analysis. Sources are generally selected to be major mergers as Ultra-Luminous IR Galaxies (Arribas et al. 2008, Alonso-Herrero et al. 2009), or 'ULIRGS', ⁷ aided by obvious morphological criteria (e.g. double-nuclei, tidal tails). Typically active on-going but pre-coalescence mergers display complex kinematic maps (in ionised gas) tracing the discs of each component (with large velocity offsets) plus kinematic disturbances induced by the merger. At high-redshift, non-parametric measures such as kinemetry are being increasingly applied to try and distinguish discs from mergers (see Section 4.5). Kinemetry (Krajnović et al. 2006) was originally developed to measure the fine kinematic structure of local elliptical galaxies and is the kinematic extension of photometric moments. It has been applied to a small sample of four local IR-selected merging galaxies by Bellocchi et al. (2012), who found good consistency with photometric classifications. There is no publication presenting quantitative or qualitative kinematic classification of a large sample of local mergers, so this would be valuable future work for comparison with high-redshift, where as we will see in Section 4.5 this has been done of necessity.

¹ The overwhelming majority of kinematic observations at z>0.5 have been optical/near-infrared utilising nebula emission lines, however radio/sub-mm observations will be mentioned and this balance is likely to change dramatically in the next decade with the advent of the Atacama Large Millimetre Array (ALMA). Back.

² IFS spatial sampling elements (e.g. lenslets or fibres) are often called 'spaxels'. This I mention solely to record for posterity this great quote: 'If spatial bins are spaxels, are spectral bins spexels and time bins tixels? But wait a tixel, those spaxels and spexels are all pixels or voxels! I say, purge the English language of these mongrel wordels!' (Matthew Colless, 2010, personal communication) Back.

³ I note that spatially resolved kinematic observations of red galaxies at high-redshift will prove very difficult as it would require the detection and measurement of stellar absorption lines at even higher angular resolution in smaller objects than has been done for the star-forming population. Back.

⁴ It is useful to also note that 2.2 scalelengths is also 1.3× the half-light radius for a pure exponential disc. Back.

⁵ i.e. if discs form a one parameter sequence of constant central surface brightness, then L∝ r² and with G M ∝ r V² one can easily show that if M ∝ L then L∝ V⁴ Back.

⁶ But see Nair et al. (2011) for a contrary opinion where the properties of elliptical galaxies are reduced to a 'Fundamental Line.' Back.

⁷ A note on the terminology: at z ~ 0 the 'LIRG' / 'ULIRG' boundary at L(IR) ≃ 10¹² L_⊙ seems to distinguish normal spirals from major mergers, however this may change to high-redshift in the sense that more galaxies in the LIRGS/ULIRGs are structurally star-forming discs due to the overall evolution in star-formation rates (Daddi et al. 2007, Daddi et al. 2008, Wuyts et al. 2011). Back.