Copyright © 2011 by Annual Reviews. All rights reserved
| Annu. Rev. Astron. Astrophys. 2011. 49:409-470
Copyright © 2011 by Annual Reviews. All rights reserved |
The statistical character of our sky's population of clusters of galaxies, viewed from radio to gamma-ray wavelengths, is sensitive to models of cosmology, astrophysics, and large-scale gravity. Galaxy clusters are cosmographic buoys that signal locations of peaks in the large-scale matter density. The population is shallow and finite. Surveys in the coming decades will definitively map our universe's terrain as defined by the highest ∼ 105 peaks. Current maps have advanced to the stage where Abell 2163, a cluster at redshift z ∼ 0.2 with a plasma virial temperature kT = 12.27 ± 0.90 keV (Mantz et al. 2010a) and galaxy velocity dispersion σgal = 1434 ± 60 km s−1 (Maurogordato et al. 2008), has been nominated a candidate for the most massive cluster in the universe (Holz & Perlmutter 2010), the cosmic equivalent of Mount Everest.
Physically, galaxy clusters are manifested in the most massive of the bound structures – termed halos (or haloes) – that emerge in the cosmic web of large-scale structure (LSS). The LSS web is a gravitationally amplified descendant of a weak noise field seeded by quantum fluctuations during an early, inflationary epoch (Bond, Kofman & Pogosyan 1996). Its evolutionary dynamics have been well studied into the non-linear regime by N-body simulations (Bertschinger 1998). Locally bound regions (the halos) emerge, initially via coherent infall within a narrow mass range and, subsequently, via a combination of infall and hierarchical merging that widen the dynamic range, pushing to increasingly larger halo masses. The merging process is of considerable interest for cluster studies, driving astrophysical signatures that can test physical models from the nature of dark matter (Clowe et al. 2006) to the magnetohydrodynamics of hot, dilute plasmas (e.g., Kunz et al. 2011). But merging also potentially confuses cosmological studies, by creating close halo pairs that may appear as one cluster in projection and by introducing variance into observable signals.
Halos are multi-component systems consisting of dark matter and baryons in several phases: black holes; stars; cold, molecular gas; warm/hot gas; and non-thermal plasma. After decades of study via N-body and hydrodynamic simulation and related methods (see recent review by Borgani & Kravtsov 2011), models for the detailed evolution of the baryons in clusters are growing in capability to describe an increasingly large and rich volume of observations. What is clear empirically is that the galaxy formation process is globally inefficient: a recent study by Giodini et al. (2009) finds that stellar mass accounts for only 12 ± 2 percent of the total baryon budget in the most massive halos. Radiative cooling of gas is overcome by feedback from various sources, including mechanical and radiative input from supernova winds and black hole jets, thermal conduction and other plasma processes, and ablation and harassment during gravitational encounters.
While the hierarchical nature of structure formation implies that galaxy and cluster formation are deeply intertwined and, therefore, that detailed understanding of cluster structure and evolution requires that we understand galaxy formation, the scales separating the most massive clusters from the largest galaxies – roughly a factor of 100 in length and 1000 in mass – allow progress to be made by approximate physical treatments. The dark matter kinematic structure, including remnant, fine-scale sub-halos (Moore et al., 1998; Springel et al. 2001), as well as the morphology and scaling behaviors of the hot, intracluster medium (ICM) that dominates the baryonic component (Evrard, 1990; Navarro, Frenk & White 1995; Bryan & Norman 1998), are examples of areas where direct simulations made good, early progress.
A key aspect of their multi-component nature is the fact that clusters offer multiple, observable signals across the electromagnetic spectrum (e.g., Sarazin 1988). At X-ray wavelengths, the hot ICM emits thermal bremsstrahlung and line emission from ionized metals injected into the plasma by stripping and feedback processes. Stellar emission from galaxies and intracluster light dominates the optical and near-infrared. At millimeter wavelengths, inverse Compton scattering within clusters distorts the spectrum of the cosmic microwave background (CMB). Gravitational lensing offers a unique probe into the total matter distributions in clusters. Synchrotron emission from relativistic electrons is visible at radio frequencies. These and other signatures discussed below provide physically coupled, and often observationally independent, lines of evidence with which to test astrophysical models of cluster evolution. A challenge to cluster cosmology is the construction of accurate statistical models that address survey observables explicitly while incorporating intrinsic property covariance.
1.1. Clusters as Cosmological Probes
The use of clusters to study cosmology has a history dating to Zwicky's discovery of dark matter in the Coma Cluster (Zwicky 1933). Brightest cluster galaxies were later employed as standard candles to study the local expansion history of the universe; Hoessel, Gunn & Thuan (1980) actually derived (with low significance) a negative deceleration parameter using this approach, implying accelerated expansion consistent with present findings. In the 1980's, measurement of the enhanced spatial clustering of clusters relative to galaxies supported the model of Gaussian random initial conditions expected from inflation (Bahcall & Soneira 1983). In the early 1990's, an apparent discrepancy between local baryon fraction measurements of clusters (Fabian 1991; Briel, Henry & Boehringer 1992) with primordial nucleosynthesis expectations helped rule out a model with critical matter density (White et al. 1993). The revelation of hot clusters at high redshift later that decade (Donahue et al. 1998; Bahcall & Fan 1998) presaged the ultimate discovery of dark energy from Type Ia supernova (SNIa) surveys. The turn of the millennium witnessed a flurry of activity aimed at measuring the amplitude of the matter power spectrum from cluster counts. X-ray studies in particular showed that the amplitude was lower than had been accepted previously (e.g., Borgani et al. 2001; Reiprich & Böhringer 2002; Seljak 2002; Pierpaoli et al. 2003; Allen et al. 2003; Schuecker et al. 2003), a result later confirmed by CMB and cosmic shear measurements. These studies also exposed the importance of understanding systematic effects associated with the use of directly observable quantities as proxies for mass (Henry et al. 2009).
Recent studies have used cluster counts or the ICM mass fraction in very massive systems (both methods described in more detail below) to constrain cosmological parameters. These studies are consistent with other observations that find a universe dominated by dark energy (73%), with sub-dominant dark matter (23%), and a small minority of baryonic material (4.6%) (Komatsu et al. 2011). A detailed pedagogical treatment of how cluster studies helped establish this reference cosmology is given in the review of Voit (2005). Rosati, Borgani & Norman (2002) review X-ray studies of clusters from the ROSAT satellite era.
Explaining the nature of the dark energy and dark matter are core problems of physics. The consensus ‘concordance’ cosmological model, ΛCDM, postulates that dark energy (DE) is associated with a small, non-zero vacuum energy, equivalent to a cosmological constant term in Einstein's equations. Another possibility is that DE arises from a light scalar field (or fields) that evolves over cosmic time. A third option is that DE is essentially an apparition, not a source term of Einstein's general relativistic equations but a reflection of their breakdown at length and time scales of cosmic dimensions (e.g., Copeland, Sami & Tsujikawa 2006). Sky surveys of cosmic systems, from supernovae to galaxies to clusters of galaxies, provide the means to discriminate among these alternatives.
Forthcoming cluster surveys at mm, optical/near-infrared, and X-ray wavelengths, discussed in Section 6.1, have the potential to find hundreds of thousands of groups and clusters. Figure 1 puts these efforts into historical perspective, by plotting size against year of publication for cluster samples that generated cosmological constraints discussed in this review. Symbol size is proportional to median sample redshift, and symbol types encode the selection method. The stars at far right show theoretical estimates of the all-sky number and median redshift of halos with masses above 1014 M⊙ and 1015 M⊙. The former mass limit roughly marks the transition from galaxy groups to galaxy clusters, while the latter marks the deepest potential wells with ICM temperatures kT ≳ 5 keV. Current surveys have made good progress, but the full population of clusters remains largely undiscovered.
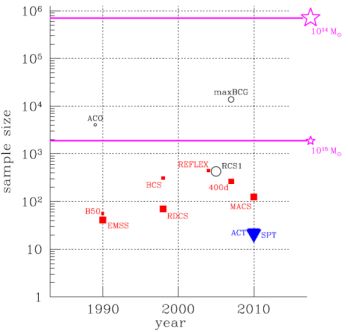 |
Figure 1. Yields from modern surveys of clusters used for cosmological studies are shown, with symbol size proportional to median redshift. Samples selected at optical (circles), X-ray (red squares), and mm (blue triangles) wavelengths are discussed in Section 3.2. Stars and horizontal lines show full sky counts of halos expected in the reference ΛCDM cosmology (see Section 2) with masses above 1015 and 1014 M⊙. Such halo samples have median redshifts of 0.4 and 0.8, respectively. |
Optical and X-ray surveys have the longest histories, but these traditional methods are being complemented by new approaches. Space-based surveys in the near-infrared extend optical methods to z > 1 (Eisenhardt et al. 2008; Demarco et al. 2010), and the first few clusters identified by their gravitational lensing signature have been published (Wittman et al. 2006). Ongoing mm surveys have released the first sets of clusters discovered through the Sunyaev-Zel’dovich (SZ) effect (Marriage et al. 2010; Vanderlinde et al. 2010; Planck Collaboration 2011a), with the promise of much more to come.
Panoramic, multi-wavelength surveys of common sky areas offer profound improvements to our understanding of clusters as astrophysical systems, which in turn further empowers their use for cosmological studies. And while considerable challenges to interpretation and modeling of survey data certainly exist, a halo model framework, discussed in Section 2, is rising to meet this task.
1.2. Cosmic Calibration via Simulations
A feature common to many techniques that study DE is the nature of the input data, which consists of catalogs of properties, x, of discrete objects that lie along our past light-cone. Upcoming wide-field surveys will generate x-catalogs of large dimension that will be distilled to constrain perhaps tens of cosmological and astrophysical parameters. Such catalogs may contain internal support through the use of complementary methods: besides galaxy clusters (CL), the same data set can be analyzed for baryon acoustic oscillations (BAO) and, for optical surveys, weak lensing (WL). (In the case of repeat observations, optical surveys can also be analyzed for Type Ia supernovae and gravitational time delay signatures.) Science processing leads to a compressed set of statistical signals, yi, where i indicates an aforementioned method. For large cluster surveys, y might consist of counts of clusters binned by sky area, redshift and detected signal.
Extracting accurate constraints on a set of cosmological model parameters, θ, from these surveys requires sophisticated likelihood analyses. The critical ingredient is p(yi | θ), the underlying likelihood that the CL (and other) statistics of the observed sky would be realized within a particular universe. Key capabilities that enable such likelihood analysis are:
Genuine understanding of cosmological models from observed cluster data is dependent on the degree to which theory and simulation can provide robust predictions for the observed signals. While numerical simulations of LSS can predict catalog-level yields for a given cosmology (e.g., Springel, Frenk & White 2006), such predictions necessarily entail additional astrophysical assumptions, meaning p(yi | θ) is actually p(yi | θ, α), where α represents degrees of freedom introduced by an assumed astrophysical model. Recovering cosmological information from survey data therefore necessitates marginalization over a reasonable range of astrophysical assumptions. On the other hand, as cosmological constraints from all methods improve, the cluster community can potentially invert the problem, recovering constraints on astrophysical models after marginalizing over cosmology.
We begin this review by describing the theoretical basis for cluster cosmology (Section 2), and include there an opening discussion of important sources of systematic error. Key observational windows are described in Section 3, and recent cosmological constraints are reviewed in Section 4. In Section 5, cluster contributions to particle physics and gravity are examined. In Section 6, we highlight opportunities for important, near-term progress. In closing, we emphasize some essential considerations in survey modeling and analysis (Section 7) before presenting our conclusions (Section 8).